In □ABCD is a quadrilateral. M is the midpoint of diagonal AC and N is the midpoint of diagonal BD. Prove that : AB2+BC2+CD2+DA2=AC2=AC2+BD2+4MN2.
 Solution in Tamil
Solution in Tamil
The correct Answer is:∴AB2+BC2+CD2+DA2=AC2=AC2+BD2+4MN2.
Step by step video & image solution for In squareABCD is a quadrilateral. M is the midpoint of diagonal AC and N is the midpoint of diagonal BD. Prove that : AB^(2)+BC^(2)+CD^(2)+DA^(2)=AC^(2)=AC^(2)+BD^(2)+4MN^(2). by Maths experts to help you in doubts & scoring excellent marks in Class 10 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CHALLENGING QUESTIONSBOOK - CHETAN PUBLICATIONCHAPTER - CHALLENGING QUESTIONSEXERCISE - Circle
9 Videos
- CHALLENGING QUESTIONSBOOK - CHETAN PUBLICATIONCHAPTER - CHALLENGING QUESTIONSEXERCISE - Geometric Constructions
6 Videos
- CHALLENGING QUESTIONSBOOK - CHETAN PUBLICATIONCHAPTER - CHALLENGING QUESTIONSEXERCISE - Similarity
9 Videos
- ARITHMETIC PROGRESSION BOOK - CHETAN PUBLICATIONCHAPTER - ARITHMETIC PROGRESSION EXERCISE - ASSIGENMENT -3
10 Videos
- CIRCLEBOOK - CHETAN PUBLICATIONCHAPTER - CIRCLEEXERCISE - Assignment - 3 (Solve any two of the following questions):
3 Videos
Similar Questions
From the end points of a diameter a diameter of circle perpendiculars are drawn to a tngent of the same circle. Show their feet on the tangent are equidistant from the centre of the circle.
View SolutionDraw a ΔABC with side BC=6 cm,∠B=45∘ and ∠A=100∘, then construct a triangle whose sides are 47 times the corresponding sides of ΔABC.
View SolutionBisector of ∠B and ∠C in ΔABC meet each other at P. Line Ap cuts the side BC at Q. Then prove that : APPQ=AB+BCBC

View SolutionWhat is the common difference (d) of the A.P. 2,-2,-6,-10….. ?
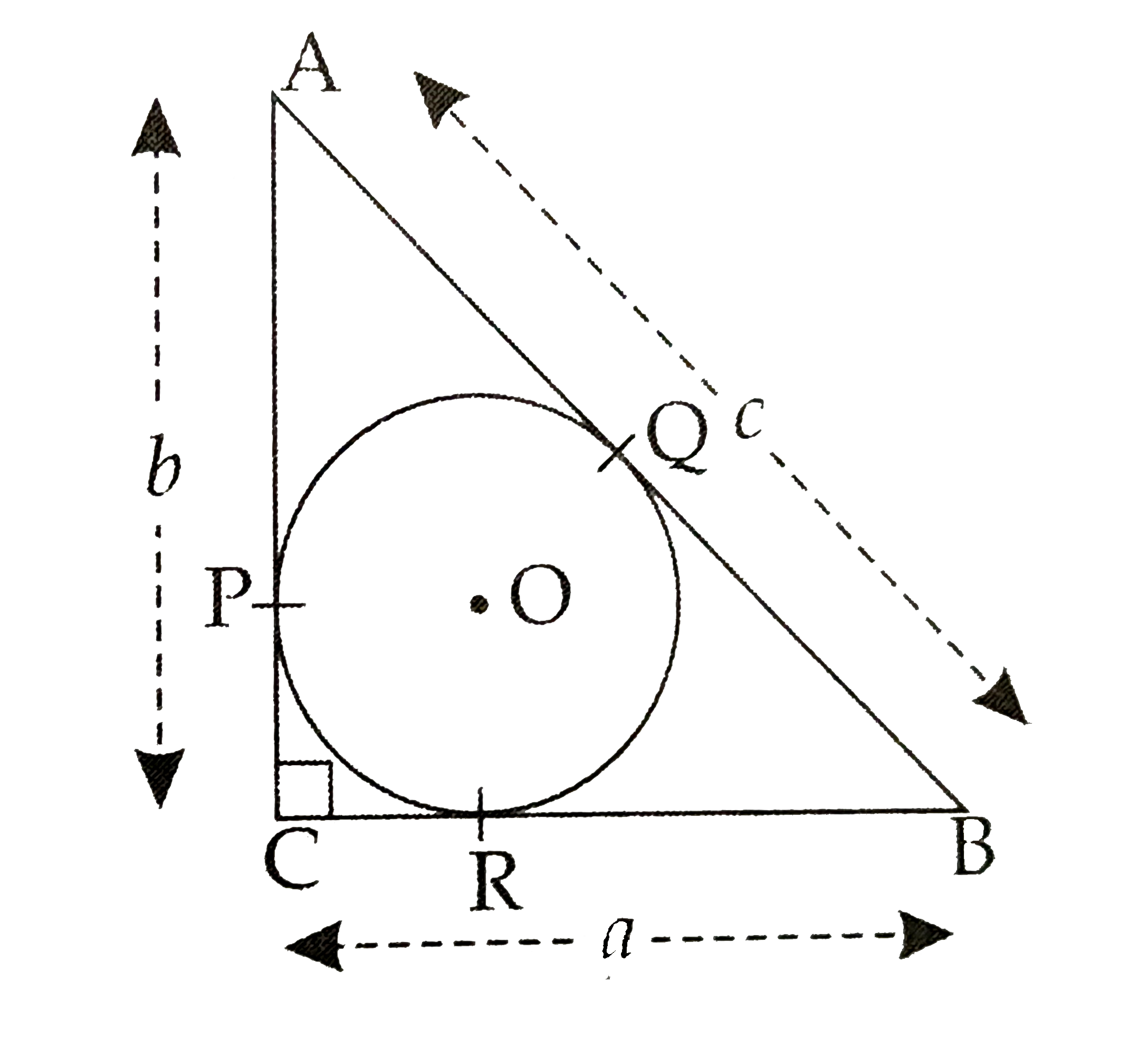
View SolutionA circle with centre 'O' is incircle of ΔABC.ΔBCA=90∘ . Radius of the circle is r.
Prove that : 2r = a + b - c.
View SolutionIn a quadrilateral ABCD, prove that AB2+BC2+CD2+DA2=AC2+BD2+4PQ2, where P and Q are middle points of diagonals AC and BD.
View SolutionIn a quadrilateral ABCD prove that AB2+BC2+CD2+DA2=AC2+BD2+4PQ2 where P and Q are middle points of diagonals AC and BD.
View SolutionThe midpoints of the sides AB,BC,CD and DA of a quadrilateral ABCD are joined to form a quadrilateral.If AC=BD and AC perp BD then prove that the quadrilateral formed is a square.
View SolutionIn the adjoining figure, ABCD is a quadrilateral. Its diagonals AC and BD intersect at point 'O'. Prove that :
(a) AB+BC+CD+DA<2(AC+BD)
(b) AB+BC+CD+DA>(AC+BD)

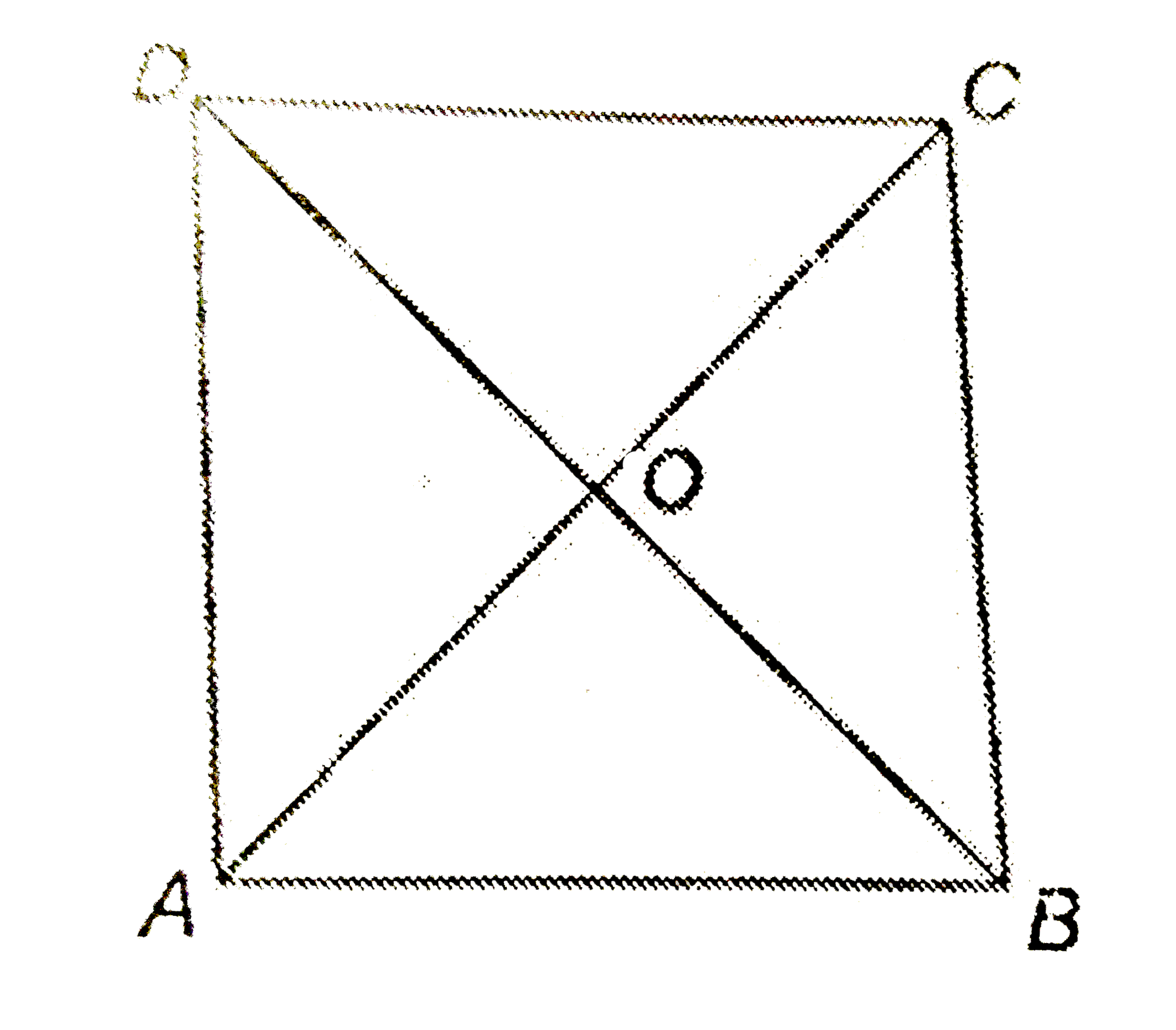
View SolutionIn the adjoining figure, the diagonals AC and BD of a quadrilateral ABCD intersect point O. Prove that :
AB+BC+CD+DA<2(AC+BD)
View SolutionIn the figure (not to scale) AB = AC and BD = CD. Find ∠ADB
ltimg src="https://d10lpgp6xz60nq.cloudfront.net/physics_images/PS_MATH_IX_C15_E05_003_Q01.png" width="80%"gt
View Solutionमाना एक चतुर्भुज ABCD है तो सिद्ध कीजिए कि AB2+BC2+CD2+DA2=AC2+BD2
View Solutionएक △ABC में, AB = AC तथा D, AC का मध्य बिंदु है और BC2=AC×CD हो तो सिद्ध कीजिए कि BD = BC
View Solutionयदि ABCD एक चतुर्भुर्ज है जिसके विकर्ण AC और BD,O पर परिच्छेद करते है तो सिद्ध कीजिए की :
(i)(AB+BC+CD+DA)>(AC+BD)
(ii) (AB+BC+CD+DA)<2(AC+BD)
View SolutionAB और CD का मध्य बिंदु O है | सिध्द करो कि (i)AOC≅BOD
(2)AC=BD तथा AC||BD.
View Solution
