A dielectric is introduced between the elements of the condenser kept at a constant potential difference, then the charge on condenser
decreases
increases
unchanged
may increase or decrease
The correct Answer is:B
To solve the problem of how the charge on a capacitor changes when a dielectric is introduced while keeping the potential difference constant, we can follow these steps:
1. Understand the Basic Formula:
The relationship between charge (Q), capacitance (C), and voltage (V) is given by the formula:
Q=C⋅V
Here, Q is the charge stored in the capacitor, C is the capacitance, and V is the potential difference across the capacitor.
2. Identify the Effect of Dielectric:
When a dielectric material is introduced between the plates of a capacitor, the capacitance increases. The new capacitance (C') with the dielectric can be expressed as:
C′=K⋅C
where K is the dielectric constant (K > 1).
3. Constant Voltage Condition:
Since the potential difference (V) across the capacitor is kept constant, we can express the new charge (Q') on the capacitor after the introduction of the dielectric:
Q′=C′⋅V
4. Substituting the New Capacitance:
Substitute the expression for C' into the equation for Q':
Q′=(K⋅C)⋅V
This simplifies to:
Q′=K⋅(C⋅V)=K⋅Q
5. Conclusion:
Since K is greater than 1, we conclude that:
Q′>Q
Therefore, the charge on the capacitor increases when a dielectric is introduced while keeping the potential difference constant.
Final Answer:
The charge on the capacitor increases when a dielectric is introduced between the plates while maintaining a constant potential difference.
The relationship between charge (Q), capacitance (C), and voltage (V) is given by the formula:
Q=C⋅V
Here, Q is the charge stored in the capacitor, C is the capacitance, and V is the potential difference across the capacitor.
When a dielectric material is introduced between the plates of a capacitor, the capacitance increases. The new capacitance (C') with the dielectric can be expressed as:
C′=K⋅C
where K is the dielectric constant (K > 1).
Since the potential difference (V) across the capacitor is kept constant, we can express the new charge (Q') on the capacitor after the introduction of the dielectric:
Q′=C′⋅V
Substitute the expression for C' into the equation for Q':
Q′=(K⋅C)⋅V
This simplifies to:
Q′=K⋅(C⋅V)=K⋅Q
Since K is greater than 1, we conclude that:
Q′>Q
Therefore, the charge on the capacitor increases when a dielectric is introduced while keeping the potential difference constant.
The charge on the capacitor increases when a dielectric is introduced between the plates while maintaining a constant potential difference.
Topper's Solved these Questions
Similar Questions
If the capacitor is kept connected with the battery and then the dielectric slab is inserted between the plates, then what will be the change in the charge, the capacitance the potential difference, the electric field an the stored energy?
View SolutionWhen a dielectric material is introduced between the plates of a charged condenser, after disconnected the battery the electric field between the plates
View Solution
Knowledge Check
A dielectric introduced between the plates of parallel plate condenser
Adecreases the electric field between the platesBdecreases the capacity of the condenserCincreases the charge stored in the condenserDincreases the capacity of the condenserA dielectric introduced between the plates of parallel plate condenser
Adecreases the electric field between the platesBdecreases the capacity of the condenserCincreases the charge stored in the condenserDincreases the capacity of the condenserThe capacity of a parallel plate condenserr is 5mF. When a glas plate is introduced between the plates of the condenser, its potential difference reduces to (1/8)th of the original value. The value of the dielectric constant of glass is
A6B8C4D10
The electric field between the two spheres of a charged spheres condenser
View SolutionThe capacity of parallel plate condenser is 5μF. When a glass plate is placed between the plates of the condenser, its potential difference reduces to 1/8 of the original value. The magnitude of relative dielectric constant of glass is
View SolutionWhen a dielectric slab of thickness 4cm is introduced between the plates of parallel plate condenser, it is found the distance between the plates has to be increased by 3cm to restore to capacity to original value. The dielectric constant of the slab is.
View SolutionWhen a dielectric slab of thickness 4cm is introduced between the plates of parallel plate condenser, it is found the distance between the plates has to be increased by 3cm to restore to capacity to original value. The dielectric constant of the slab is.
View SolutionTwo identical capacitors are connected as shown in the figure. A dielectric slab is introduced between the plates of one of the capacitors so as to fill the gap, the battery remaining connected. The charge on each capacitor will be (charge on each condenser is q0,k= dielectric constant).
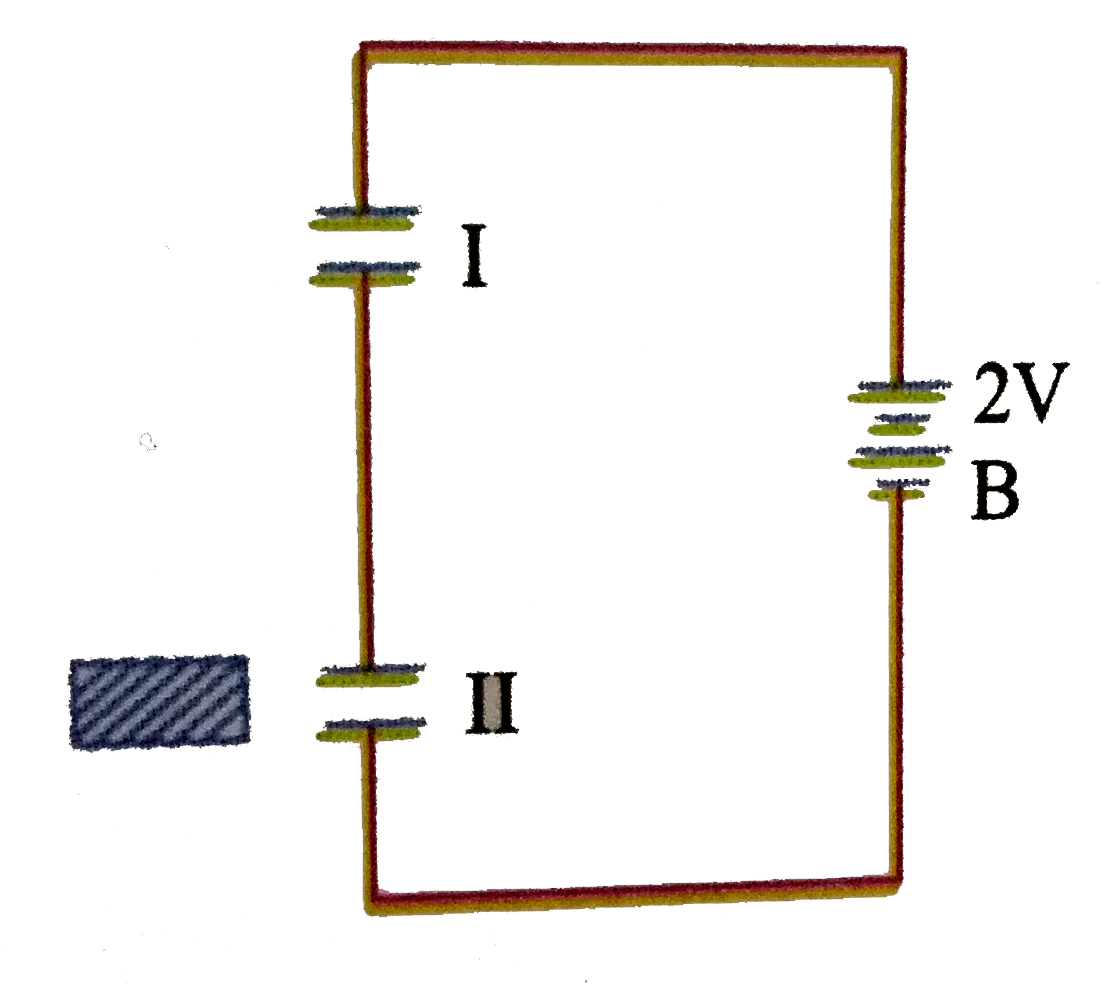 .
.
View Solution
