Question

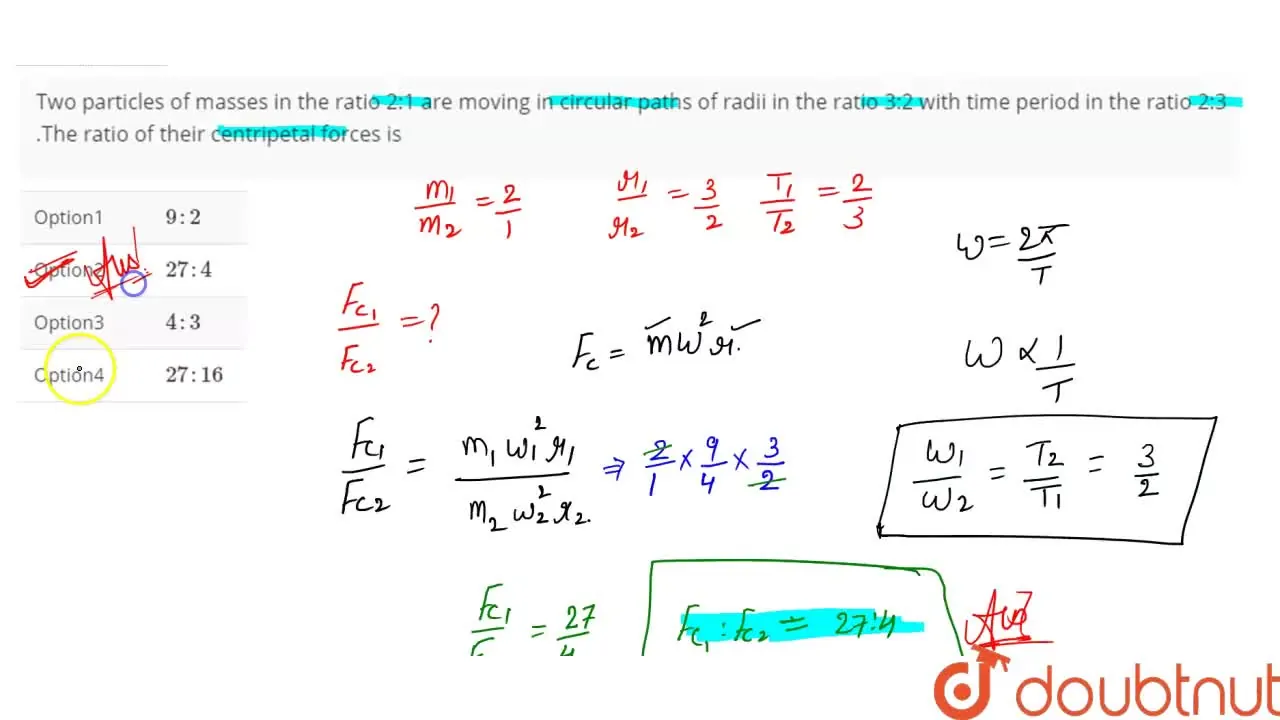
Two particles of masses in the ratio 2:1 are moving in circular paths of radii in the ratio 3:2 with time period in the ratio 2:3 .The ratio of their centripetal forces is

Options

A. `9:2`
B. `27:4`
C. `4:3`
D. `27:16`

Answer

B

Explanation

To solve the problem step by step, we will use the information given about the two particles and the formulas related to centripetal force.

Step 1: Understand the given ratios
We are given:
- Masses: \( m_1 : m_2 = 2 : 1 \)
- Radii: \( r_1 : r_2 = 3 : 2 \)
- Time periods: \( T_1 : T_2 = 2 : 3 \)

Step 2: Relate angular velocity to time period
The angular velocity \( \omega \) is related to the time period \( T \) by the formula:
\( \omega = \frac{2\pi}{T} \)
From the ratio of time periods, we can find the ratio of angular velocities:
\( \frac{\omega_1}{\omega_2} = \frac{T_2}{T_1} = \frac{3}{2} \)

Step 3: Write the expression for centripetal force
The centripetal force \( F_c \) for a particle moving in a circular path is given by:
\( F_c = m \omega^2 r \)
Thus, for the two particles, we have:
\( F_{c1} = m_1 \omega_1^2 r_1 \)
\( F_{c2} = m_2 \omega_2^2 r_2 \)

Step 4: Find the ratio of centripetal forces
Now, we can find the ratio of the centripetal forces:
\( \frac{F_{c1}}{F_{c2}} = \frac{m_1 \omega_1^2 r_1}{m_2 \omega_2^2 r_2} \)

Step 5: Substitute the known ratios
Substituting the ratios we have:
- \( \frac{m_1}{m_2} = \frac{2}{1} \)
- \( \frac{r_1}{r_2} = \frac{3}{2} \)
- \( \frac{\omega_1}{\omega_2} = \frac{3}{2} \) implies \( \frac{\omega_1^2}{\omega_2^2} = \left(\frac{3}{2}\right)^2 = \frac{9}{4} \)

Now substituting these into the ratio of centripetal forces:
\( \frac{F_{c1}}{F_{c2}} = \frac{2}{1} \cdot \frac{9}{4} \cdot \frac{3}{2} \)

Step 6: Simplify the expression
Now we simplify:
\( \frac{F_{c1}}{F_{c2}} = \frac{2 \cdot 9 \cdot 3}{1 \cdot 4 \cdot 2} = \frac{54}{8} = \frac{27}{4} \)

Final Answer
Thus, the ratio of the centripetal forces is:
\( \frac{F_{c1}}{F_{c2}} = \frac{27}{4} \)