A resistance R and an inductance L are connected in series in an a.c. circut. If ω is the angular frequency of the source, then the power factor is given by
ωLR
RωL
R√R2+ω2L2
R√R2−ω2L2
The correct Answer is:C
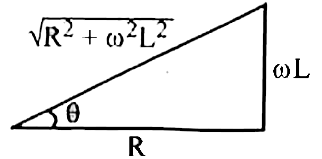
tanθ=ωLR
∴ Power factor = cosθ=R√R2+L2ω2
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTS BOOK - MARVEL PUBLICATIONCHAPTER - ELECTROMAGNETIC INDUCTION AND ALTERNATING CURRENTS EXERCISE - TEST YOUR GRASP - 16
30 Videos
- ELASTICITYBOOK - MARVEL PUBLICATIONCHAPTER - ELASTICITYEXERCISE - Test Your Grasp - 5
15 Videos
- ELECTRONS AND PHOTONS BOOK - MARVEL PUBLICATIONCHAPTER - ELECTRONS AND PHOTONS EXERCISE - TEST YOUR GRASP - 17
15 Videos
Similar Questions
Resistor of resistance R and capacitor of capacitance C are connected in series to an AC source of angular frequency ω . Rms current in the circuit is I. When frequency of the source is changed to one - third of initial value keeping the voltage same then current is found to be halved. Find the ratio of reactance of capacitor to that with resistance at the original frequency ω .
View SolutionA choke coil of resistance R and inductance is connected to an ac. source of frequency f and peak voltage V If angular frequency f will increase then, the average power dissipated in the choke
View Solution
Knowledge Check
200 Ω resistance and 1H inductance are connected in series with an A.C. circuit. The frequency of the source is 2002π Hz. Then phase difference in between V and I will be-
A30∘B60∘C45∘D90∘In an A.C. circuit, a resistance R=40Ω and an inductance L are connected in series. If the phase angle between voltage and current is 45∘, then the value of the inductive reactance will be
A20ΩB40ΩC10ΩD50ΩThe impedance of a circuit, when a resistance R and an inductor of inductance are connected in series in an AC circuit of frequency f is
A√R+2π2f2L2B√R+4π2f2L2C√R2+4π2f2L2D√R2+2π2f2L2
An inductor has a resistance R and inductance L .It is connected to an A.C source of e.m.f E_(v) and angular frequency omega , then the current I_(v) in the circuit is
View SolutionA resistance (R), inductance (L) and capacitance (C) are connected in series to an ac source of voltage V having variable frequency. Calculate the energy delivered by the source to the circuit during one period if the operating frequency is twice the resonance frequency.
View SolutionA capacitor with capacitance C and a coil with active resistance R and inductance L are connected in parallel to a source of sinusoidal voltage of frequency ω. Find the phase difference between the current fed to the circuit and the source voltage.
View SolutionA capacitor of 100μF, a resistor of 20Ω and an inductor of inductance L are connected in series with an a.c. source of frequency 50 Hz. Calculate the value of inductance L of the inductor, if phase angle between current and voltage is zero.
View SolutionIn the given LR circuit the source has angular frequency ω The power factore of the circuit is
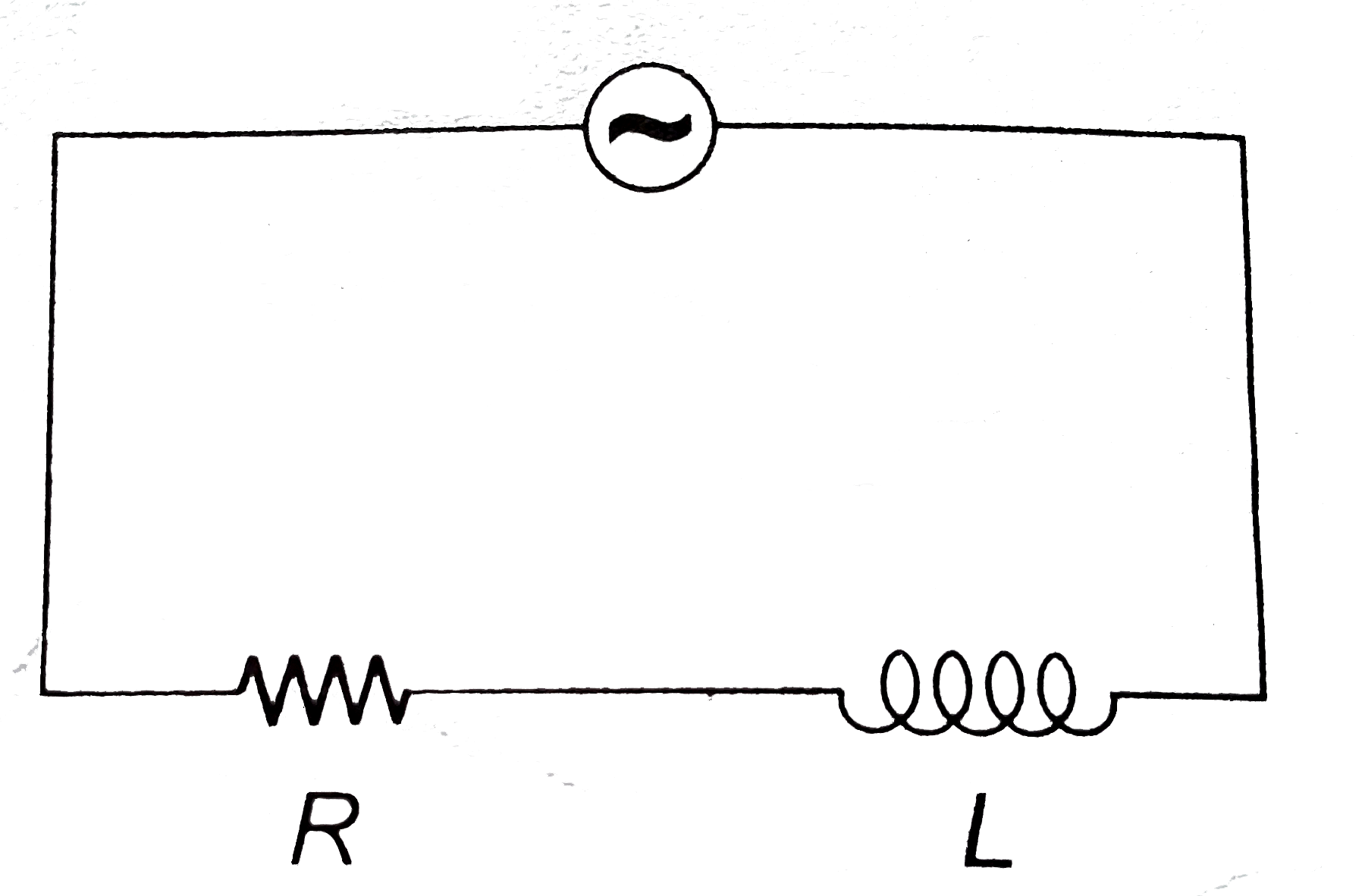 .
.
View Solution
