∫π/2−π/2sin(|x|)dx=
1
2
−1
−2
The correct Answer is:B
To solve the integral ∫π2−π2sin(|x|)dx, we can follow these steps:
Step 1: Understand the function
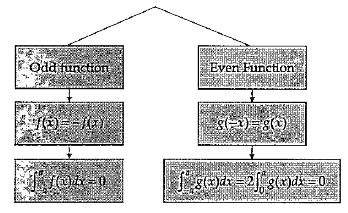
The function sin(|x|) means that for negative values of x, the function will reflect the positive values. Therefore, we can split the integral into two parts:
∫π2−π2sin(|x|)dx=∫0−π2sin(−x)dx+∫π20sin(x)dx
Step 2: Simplify the integral
Since sin(−x)=−sin(x), we can rewrite the first integral:
∫0−π2sin(−x)dx=−∫0−π2sin(x)dx
Thus, we have:
∫π2−π2sin(|x|)dx=−∫0−π2sin(x)dx+∫π20sin(x)dx
Step 3: Change the limits of the first integral
We can change the variable in the first integral. Let u=−x, then du=−dx. When x=−π2, u=π2 and when x=0, u=0:
−∫0−π2sin(x)dx=∫π20sin(u)du
So now we can combine the integrals:
∫π2−π2sin(|x|)dx=∫π20sin(x)dx+∫π20sin(x)dx=2∫π20sin(x)dx
Step 4: Evaluate the integral
Now we need to evaluate ∫π20sin(x)dx:
∫sin(x)dx=−cos(x)+C
Thus,
∫π20sin(x)dx=(−cos(x))π20=−cos(π2)−(−cos(0))=0−(−1)=1
Step 5: Final calculation
Now substituting back:
∫π2−π2sin(|x|)dx=2⋅1=2
Final Answer
Thus, the value of the integral is:
∫π2−π2sin(|x|)dx=2
The function sin(|x|) means that for negative values of x, the function will reflect the positive values. Therefore, we can split the integral into two parts:
∫π2−π2sin(|x|)dx=∫0−π2sin(−x)dx+∫π20sin(x)dx
Since sin(−x)=−sin(x), we can rewrite the first integral:
∫0−π2sin(−x)dx=−∫0−π2sin(x)dx
Thus, we have:
∫π2−π2sin(|x|)dx=−∫0−π2sin(x)dx+∫π20sin(x)dx
We can change the variable in the first integral. Let u=−x, then du=−dx. When x=−π2, u=π2 and when x=0, u=0:
−∫0−π2sin(x)dx=∫π20sin(u)du
So now we can combine the integrals:
∫π2−π2sin(|x|)dx=∫π20sin(x)dx+∫π20sin(x)dx=2∫π20sin(x)dx
Now we need to evaluate ∫π20sin(x)dx:
∫sin(x)dx=−cos(x)+C
Thus,
∫π20sin(x)dx=(−cos(x))π20=−cos(π2)−(−cos(0))=0−(−1)=1
Now substituting back:
∫π2−π2sin(|x|)dx=2⋅1=2
Thus, the value of the integral is:
∫π2−π2sin(|x|)dx=2
Topper's Solved these Questions
- INTEGRATION - DEFINITE INTEGRALS BOOK - MARVEL PUBLICATIONCHAPTER - INTEGRATION - DEFINITE INTEGRALS EXERCISE - MULTIPLE CHOICE QUESTIONS (PREVIOUS YEARS (MHT-CET EXAM QUESTIONS))
12 Videos
- INTEGRATION - DEFINITE INTEGRALS BOOK - MARVEL PUBLICATIONCHAPTER - INTEGRATION - DEFINITE INTEGRALS EXERCISE - TEST YOUR GRASP
20 Videos
- INTEGRATION - DEFINITE INTEGRALS BOOK - MARVEL PUBLICATIONCHAPTER - INTEGRATION - DEFINITE INTEGRALS EXERCISE - TEST YOUR GRASP
20 Videos
- DIFFERENTIATIONBOOK - MARVEL PUBLICATIONCHAPTER - DIFFERENTIATIONEXERCISE - MULTIPLE CHOICE QUESTIONS (TEST YOUR GRASP - II : CHAPTER 11)
24 Videos
- INTEGRATION - INDEFINITE INTEGRALSBOOK - MARVEL PUBLICATIONCHAPTER - INTEGRATION - INDEFINITE INTEGRALSEXERCISE - TEST YOUR GRASP
45 Videos
Similar Questions
Knowledge Check
∫π/2−π/2sin7xdx=
A7π16B2π35C2πD0∫π/2−π/2sin|x|dx is equal to
A0B1C2Dπ∫π/2−π/2sin|x|dx is equal to
A0B1C2Dπ
∫π/2−π/2sin|x|dx is equal to 1 (b) 2 (c) −1 (d) −2
View SolutionRead the following text and answer the followig questions on the basis of the same :
∫π/2−π/2sin3xdx =
View Solution∫π2−π2sin2xdx
View SolutionThe value of l=∫π/2−π/2|sinx|dx is
View SolutionFind our value of I=∫π/2−π/2sin2xdx :
View Solution
