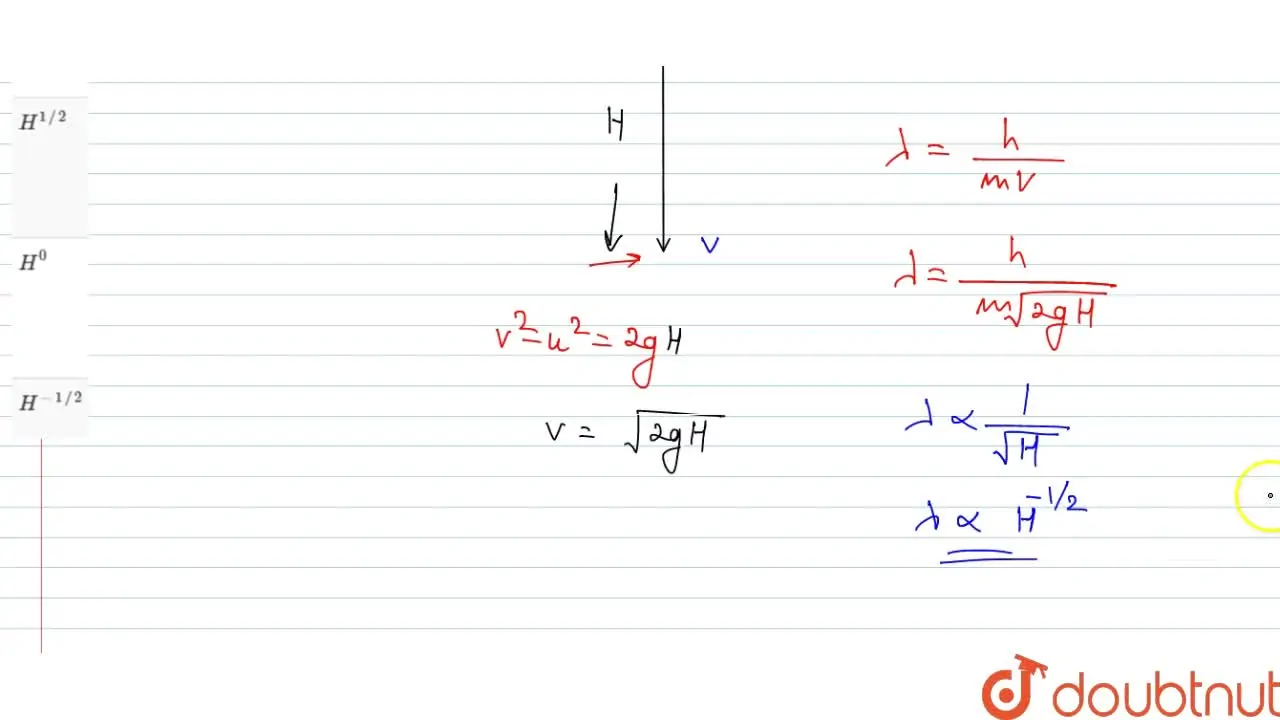
A particle is droped from a height H. The de-broglie wavelength of the particle as a funciton of height is proportional to
H
H1/2
H0
H−1/2
The correct Answer is:d
Velocity acquired by a particle while falling form a height H is, v=√2gH
λ=hmv=hm√2gHorλ∝H−1/2. Hence option (d) is correct.
Related Playlists
- BOOK - PRADEEPCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Value based question1videos
DUAL NATURE OF RADIATION AND MATTER
- BOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Problems for Practice (B)2videos
CURRENT ELECTRICITY
- BOOK - PRADEEPCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - Multiple Choice Questions1videos
ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENT
Similar Questions
A particle is dropped from a height H. The de Broglie wavelength of the particle as a function of height is proportional to
View SolutionThe de Broglie wavelength λ of a particle
View SolutionThe de Broglie wavelength λ of a particle
View SolutionThe de Broglie wavelength associated with particle is
View SolutionThe de-Broglie wavelength is proportional to
View SolutionThe de-Broglie wavelength associated with a particle of momentum p is given as :
View SolutionFor particles having same K.E., the de-Broglie wavelength is
View SolutionIf the kinetic energy of a particle is doubled, by what factor the de Broglie wavelength of the particle increase or decrease ?
View SolutionDe-Broglie wavelengths of the particle increases by 75% then kinetic energy of particle becomes.
View SolutionThe de Broglie wavelength (λ) of a particle is related to its kinetic energy E as
View Solution