The slope of frequency of incident light and stopping potential for a given surface will be
h
h/e
eh
e
The correct Answer is:b
To find the slope of the graph of frequency of incident light versus stopping potential for a given surface, we can use Einstein's photoelectric equation. Let's derive the relationship step by step.
1. Understanding the Photoelectric Effect:
The photoelectric effect occurs when light of sufficient frequency strikes a material and causes the ejection of electrons. The energy of the incident photons must be greater than the work function of the material for electrons to be emitted.
2. Einstein's Photoelectric Equation:
The equation is given by:
E⋅V0=hν−ϕ0
where:
- E is the charge of the electron,
- V0 is the stopping potential,
- h is Planck's constant,
- ν is the frequency of the incident light,
- ϕ0 is the work function of the material.
3. Rearranging the Equation:
We can rearrange the equation to express V0 in terms of ν:
E⋅V0=hν−ϕ0
Dividing through by E:
V0=hEν−ϕ0E
4. Identifying the Linear Relationship:
The equation V0=hEν−ϕ0E is in the form of y=mx+c, where:
- y is the stopping potential V0,
- x is the frequency ν,
- m (the slope) is hE,
- c (the y-intercept) is −ϕ0E.
5. Conclusion:
The slope of the graph of frequency of incident light versus stopping potential is:
slope=hE
Final Answer:
The slope of the frequency of incident light and stopping potential for a given surface will be hE.
The photoelectric effect occurs when light of sufficient frequency strikes a material and causes the ejection of electrons. The energy of the incident photons must be greater than the work function of the material for electrons to be emitted.
The equation is given by:
E⋅V0=hν−ϕ0
where:
- E is the charge of the electron,
- V0 is the stopping potential,
- h is Planck's constant,
- ν is the frequency of the incident light,
- ϕ0 is the work function of the material.
We can rearrange the equation to express V0 in terms of ν:
E⋅V0=hν−ϕ0
Dividing through by E:
V0=hEν−ϕ0E
The equation V0=hEν−ϕ0E is in the form of y=mx+c, where:
- y is the stopping potential V0,
- x is the frequency ν,
- m (the slope) is hE,
- c (the y-intercept) is −ϕ0E.
The slope of the graph of frequency of incident light versus stopping potential is:
slope=hE
The slope of the frequency of incident light and stopping potential for a given surface will be hE.
Topper's Solved these Questions
- DUAL NATURE OF RADIATION AND MATTERBOOK - PRADEEPCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Value based question
1 Videos
- CURRENT ELECTRICITYBOOK - PRADEEPCHAPTER - CURRENT ELECTRICITYEXERCISE - Problems for Practice (B)
2 Videos
- ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTBOOK - PRADEEPCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - Multiple Choice Questions
1 Videos
Similar Questions
The graph between frequency of incident radiations and stopping potential for a given photosensitive material as follows.
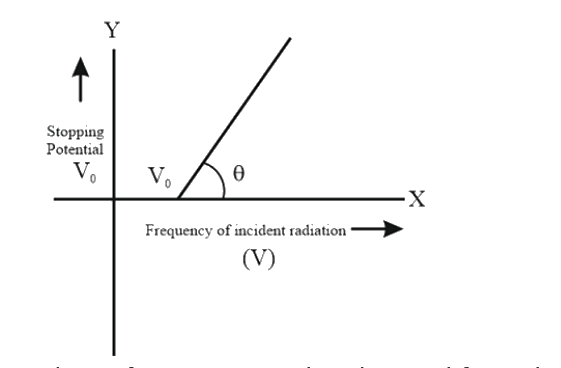
What information can be obtained from the value of the intercept on the potential axis ?
View SolutionSketch a graph between frequency of incident radiations and stopping potential for a given photosenstive material. What information can be obtained from the value of the intercept on the potential axis? A source of light of frequency greater than the threshold frequency is placed at a distance of 1m from the cathode of a photocell. The stopping potential is found to be V. If the distance of the light source from the cathode is reduced, explain giving reasons, what change will you observe in the (i) photoelectric current (i) stopping potential?
View Solution
Knowledge Check
In a photoelectric experiment, the graph of frequency v of incident light (in Hz) and stopping potential V (in V) is as shown in the figure. Planck's constant is (e is the elementary charge)
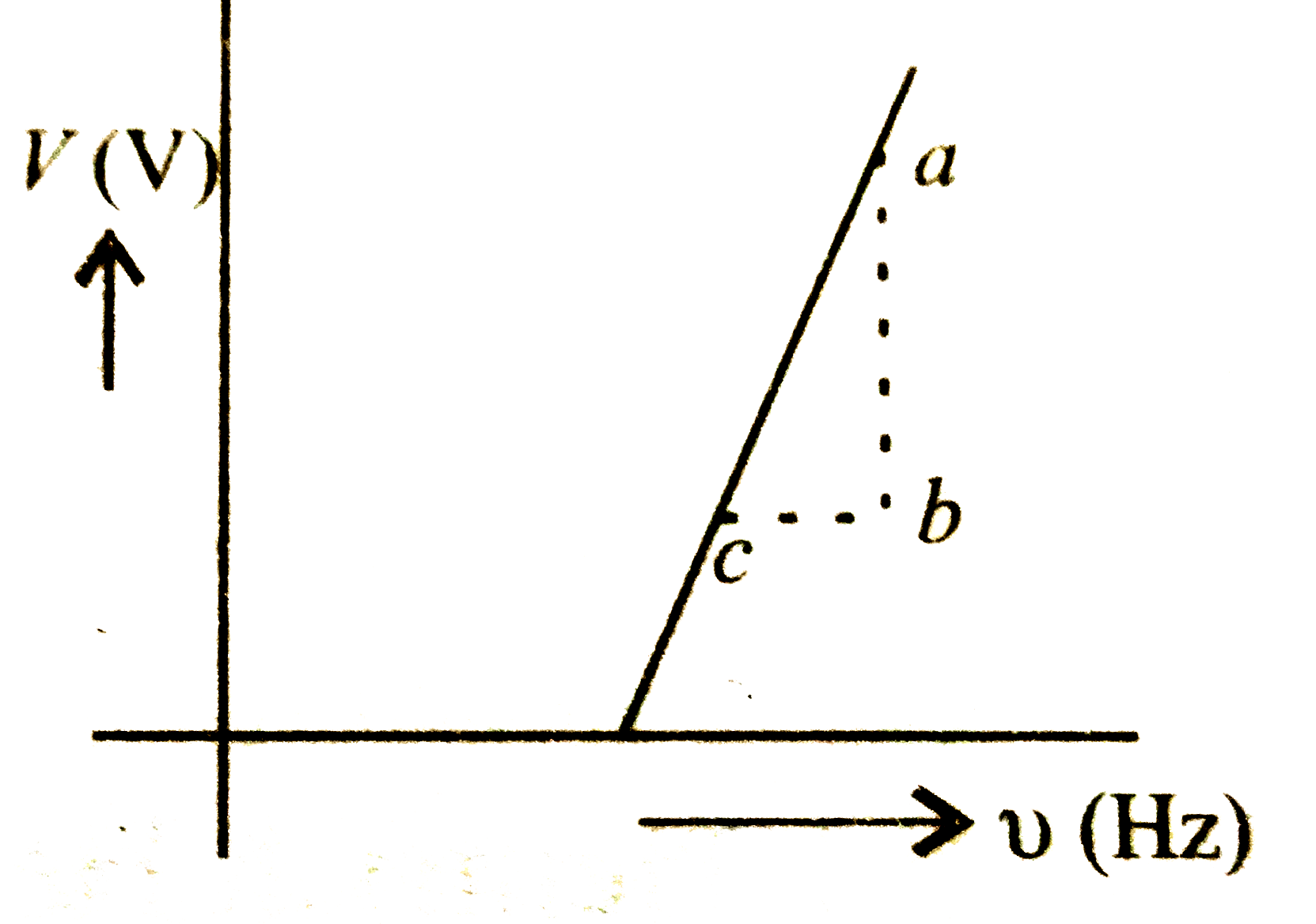 AeabbcBecbacCeacbcDeacab
AeabbcBecbacCeacbcDeacabThe photoelectric work function of the emitter of a photocell is 3.63 eV . The frequency of incident light radiation if the stopping potential of the emitter is 3 volt , is
A1.1×1015 HzB6.6×1015 HzC1.6×1015 HzD6.1×1015 HzIn photoelectric effect the slope of stop of stopping potential versus frequency of incident light for a given surface will be
Ahe−1Be hCeDh
The stopping potential (v0) varies linearly with the frequency (v)of the incident radiation for a given photosensitive surface with the slope remaining the same for different surface . Given reason.
View SolutionChoose the correct option for the graph between the frequency of incident light and the stopping potential.
View SolutionAssertion : The stopping potential depends on the frequency of incident light.
Reason : The stopping potential is related to maximum kinetic energy by eV0=Kmax.
View SolutionAssertion: If frequency of incident light is doubled, the stopping potential will also become two times
Reason: Stopping potential is given by V0=he(v−v0)
View SolutionMonochromatic light of frequency f1 incident on a photocell and the stopping potential is found to be V1. What is the new stopping potential of the cell if it is radiated by monochromatic light of frequency f2?
View Solution
