When a circuit element X is conneted across a.c. source, a current √2A flows through it and this current is in phase with applied voltage. When another element Y is connected across same a.c. source, the same current flows in the circuit, but it leads the voltages by π/2. Name the circuit elements X and Y. Find current that flows in the circuit when series combination of X and Y is connected acorss same a.c. voltage. Plot a graph showing variation of net impedance of series combination of X and Y as a function of angular frequency of applied voltage.
Step-by-Step Solution
1. Identify Circuit Elements X and Y:
- Element X has a current that is in phase with the applied voltage. This indicates that X is a resistor (R).
- Element Y has a current that leads the voltage by π2. This indicates that Y is a capacitor (C).
2. Determine the Impedance of Each Element:
- For the resistor (X):
- The current I=√2A implies that the voltage across the resistor can be calculated using Ohm's Law:
V=I⋅R
- For the capacitor (Y):
- The current leads the voltage by π2, which means the impedance of the capacitor is given by:
ZC=1jωC
3. Calculate the Resistance (R):
- Given that the current through the resistor is I=√2A, we can express the voltage across the resistor as:
VR=I⋅R=√2⋅R
- The RMS voltage across the resistor can be expressed as:
VRrms=√2⋅R
4. Calculate the Capacitive Reactance (X_C):
- The current through the capacitor is also I=√2A. The voltage across the capacitor can be expressed as:
VC=I⋅XC=√2⋅XC
- The capacitive reactance XC can be expressed as:
XC=VCrmsI=√2⋅XC√2=XC
5. Find the Total Impedance (Z) in Series:
- The total impedance Z of the series combination of R and C is given by:
Z=√R2+X2C
- Substituting R=VRrms√2 and XC=1ωC:
Z=
⎷(VRrms√2)2+(1ωC)2
6. Calculate the Current in the Series Circuit:
- The current in the series circuit can be calculated using:
Irms=VrmsZ
- Substituting the expression for Z:
Irms=Vrms√R2+X2C
7. Plotting the Graph:
- The graph of net impedance Z as a function of angular frequency ω can be plotted. The impedance will vary with frequency due to the capacitive reactance XC=1ωC.
- The graph will show a curve where Z decreases as ω increases because XC decreases with increasing frequency.
- Element X has a current that is in phase with the applied voltage. This indicates that X is a resistor (R).
- Element Y has a current that leads the voltage by π2. This indicates that Y is a capacitor (C).
- For the resistor (X):
- The current I=√2A implies that the voltage across the resistor can be calculated using Ohm's Law:
V=I⋅R
- For the capacitor (Y):
- The current leads the voltage by π2, which means the impedance of the capacitor is given by:
ZC=1jωC
- Given that the current through the resistor is I=√2A, we can express the voltage across the resistor as:
VR=I⋅R=√2⋅R
- The RMS voltage across the resistor can be expressed as:
VRrms=√2⋅R
- The current through the capacitor is also I=√2A. The voltage across the capacitor can be expressed as:
VC=I⋅XC=√2⋅XC
- The capacitive reactance XC can be expressed as:
XC=VCrmsI=√2⋅XC√2=XC
- The total impedance Z of the series combination of R and C is given by:
Z=√R2+X2C
- Substituting R=VRrms√2 and XC=1ωC:
Z=
⎷(VRrms√2)2+(1ωC)2
- The current in the series circuit can be calculated using:
Irms=VrmsZ
- Substituting the expression for Z:
Irms=Vrms√R2+X2C
- The graph of net impedance Z as a function of angular frequency ω can be plotted. The impedance will vary with frequency due to the capacitive reactance XC=1ωC.
- The graph will show a curve where Z decreases as ω increases because XC decreases with increasing frequency.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTBOOK - PRADEEPCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - Solved Examples (b)
1 Videos
- ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTBOOK - PRADEEPCHAPTER - ELECTROMAGNETIC INDUCTION & ALTERNATING CURRENTEXERCISE - Short Answer Qusetions
2 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - PRADEEPCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Exercise
191 Videos
- ELECTROMAGNETIC WAVESBOOK - PRADEEPCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - II Focus multiple choice question
5 Videos
Similar Questions
When a circuit element X is connected across on a.c. source of e.m.f. 220√2 volt, current of √2 A flows through it in phase with the voltage. When another element Y is connected across the same a.c. source, the same current flows through the circuit, but it leads the voltage by π/2 radian. Name the circuit element X and Y.
Find the current that flows in the circuit when series combination of X and Y is connected across the same source.
View Solution
Current flows through the above circuit when
View Solution
Knowledge Check
When an alternating voltage of 220V is applied across a device P, a current of 0.25A flows through the circuit and it leads the applied voltage by a angle π2 radian. When the same voltage source is connected across another device Q, the same current is observed in the circuit but in phase with the applied voltage. What is the current when the same source is connected across a series combination of P and Q?
A14√2 A lagging in phase by π4 with voltageB14√2 A leading in phase by π4 with voltageC1√2 A leading in phase by π4 with voltageD14√2 A leading in phase in π2 with voltageIn an ac circuit, the current lags behind the voltage by π/3. The components in the circuit are
AR and LBR and CCL and CDOnly RSeries LCR circuit is connected to an AC source of angular frequency ω. Current flowing in the circuit is found to lead the voltage by π/4. Magnitude of capacitance is
A1ω2(L+R)B1ω(L+R)C1ω2L+ωRD1ωL+ω2R
When an electric device X is connected to a 220 volt, 50 hertz a.c. supply, the current is 0.5 amp, and is in same phase as the applied voltage. When another device Y is connected to the same supply, the electric current is again 0.5amp, but it leads the potential difference by π/2 (i) What are the devices X and Y ? (ii) When X and Y are connected in serices across the same source, what will be the current ?
View SolutionWhen an alternating voltage of 220 V is applied across a device X, a current of 0.25 A flows which lags behind the voltage in phase by π2 rad. If the same voltage is applied across another device Y, the same current flows but now it is in phase with the applied voltage.
(a) Name the devices X and Y.
(b) Calculate the current flowing in the circuit when the same voltage is applied across the series combination of X and Y.
View SolutionYou are given three circuits X, Y and Z when the element X is connected across an a.c. source of a given voltage, the current and voltage are in same phase. When element Y is connected in series with X across the source, voltage is ahead of voltage by π/4. But the current is ahead of voltage by π/4, when Z is connected series with X, across the source. Identify the circuit elements X, Y and Z.
When all the three elements are connected in series across the same source, determine the impedance of the circuit . Draw a plot of current versus the frequency of applied source and mention the significance of this plot.
View SolutionIn the following circuit the current is in phase with the applied voltage. Therefore, the current in the circuit and the frequency of the source voltage respectively, are
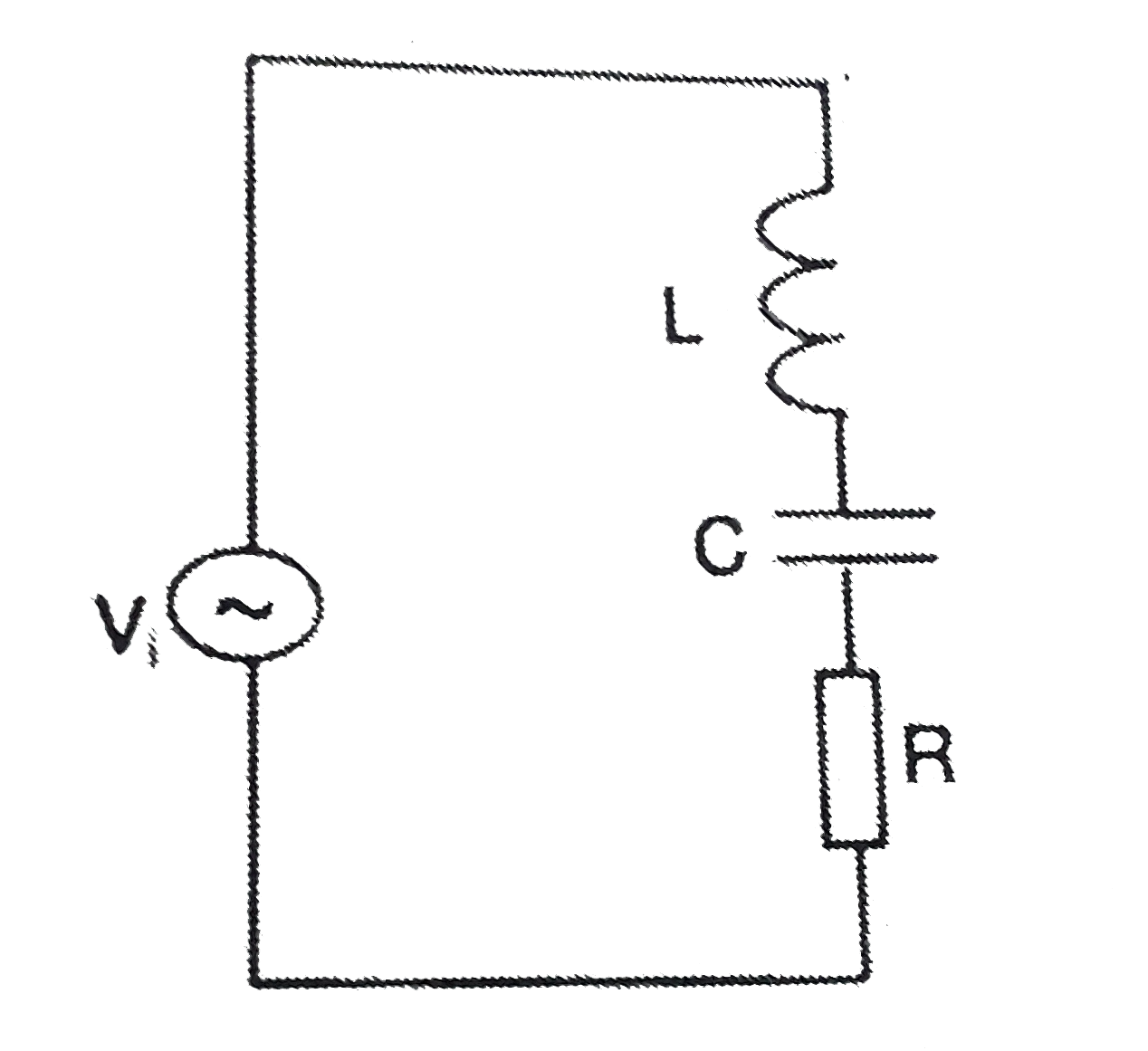
View SolutionWhen a circuit is switched on, current flows through the circuit with the speed of light because
View Solution
