A spring of force constant $1200 N m^{- 1}$ is mounted on a horizontal table as shown in figure. A mass of 3.0kg is attached to the free end of the spring, pulled side ways to a distance of 2.0cm and released , what is
(a) The speed of the mass when the spring is compressed by 1.0cm?
(b) Potential energy of the mass when it momentarily comes to rest ?
(c) Total energy of the oscillating mass.

Video Solution

Text Solution

Verified by Experts

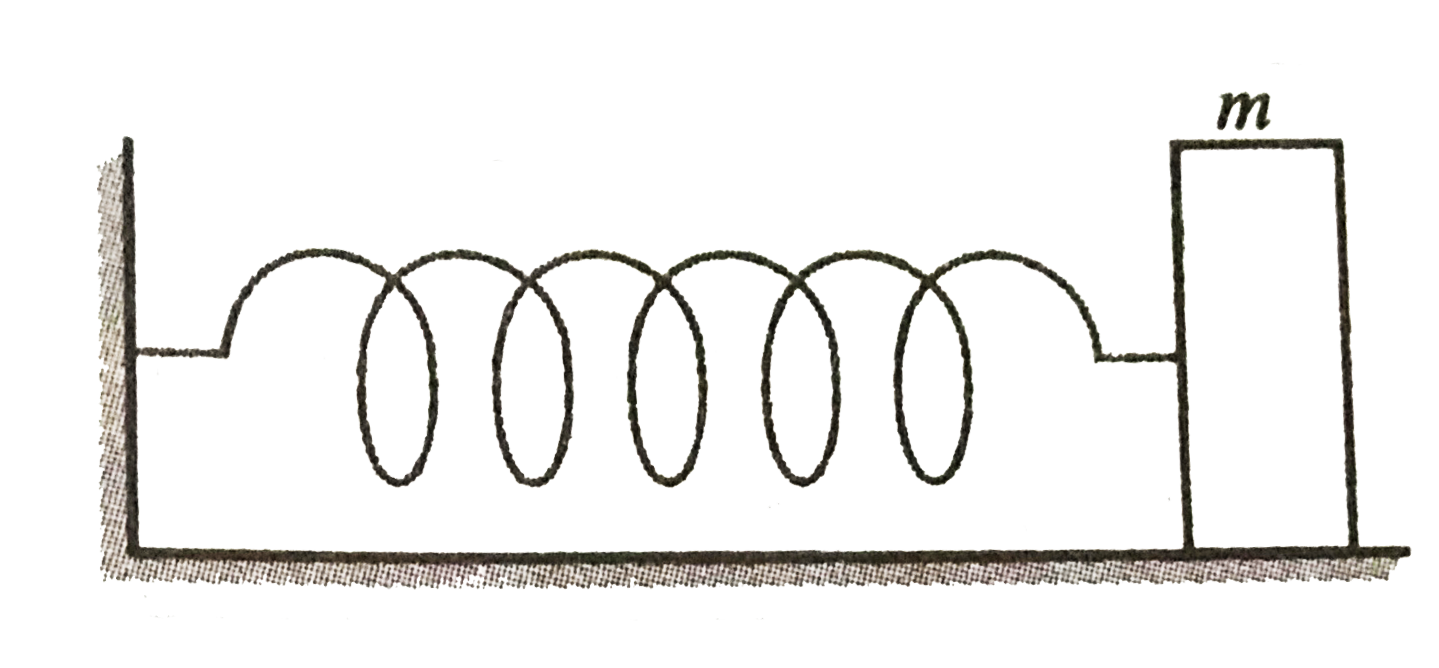
Here, $k = 1200 N m^{- 1}, m = 3.0 k g,$
$y = 1.0 c m = 0.01 m,$
$a = 2.0 c m = 0.02 m$
(a) Speed, $V = ω \sqrt{a^{2} - y^{2}}$
$= \sqrt{\frac{k}{m}} {(a^{2} - y^{2})}^{1 / 2}$
$\sqrt{\frac{1200}{3}} {[{(0.02)}^{2} - {(0.01)}^{2}]}^{1 / 2}$
$= 0.346 m s^{- 1}$
(b) The body is momentarily at rest, when $y = a$
$P . E . = \frac{1}{2} k a^{2} = \frac{1}{2} \times 1200 \times {(0.02)}^{2} = 0.24 J$
(c) Total energy $=$ P.E. $+$ K.E.
$= 0.24 + 0 = 0.24 J$

Updated on:21/07/2023

A spring of force constant $600 N m^{- 1}$ is mounted on a horizontal table. A mass of 1.5 kg is attached to the free end of the spring,pulled sideways to a distance of 2 cm and released . The speed of the mass when the spring is compressed by 1 cm is
A $0.175 m s^{- 1}$
B $0.35 m s^{- 1}$
C $0.7 m s^{- 1}$
D $1.4 m s^{- 1}$
A spring of force constant $600 N m^{- 1}$ is mounted on a horizontal table. A mass of 1.5 kg is attached to the free end of the spring, pulled sideways to a distance of 2 cm and released .P.E. of the mass when it momentarily comes to rest and total energy are
A $0.12 J, 0$
B $0, 0.12 J$
C $0, 0$
D $0.12 J, 0.12 J$
The spring is compressed by a distance a and released. The block again comes to rest when the spring is elongated by a distance $b$ . During this

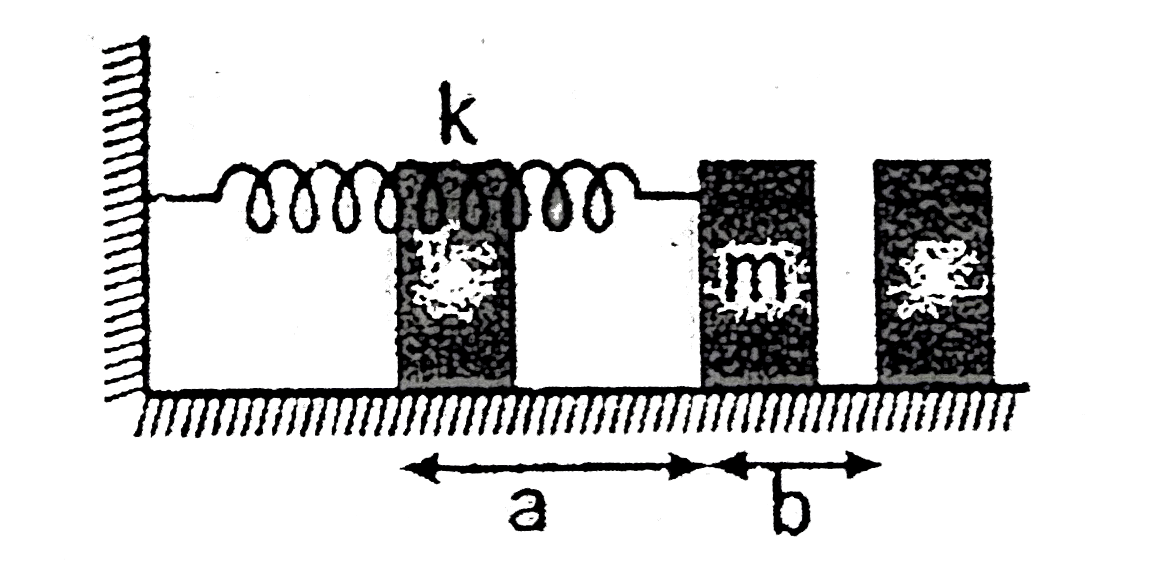Awork done by sprinn on the block $= \frac{1}{2} k {(a + b)}^{2}$
Bwork done by sprinn on the block $= \frac{1}{2} k (a^{2} - b^{2})$
Cco-eff. of frication $= \frac{k (a - b)}{2 m g}$
Dco-eff. of frication $= \frac{k (a + b)}{2 m g}$