A particle moves according to the law, . What is the distance covered by it in time interval to second.
To solve the problem, we need to determine the distance covered by the particle moving according to the equation x=acos(πt2) over the time interval from t=0 seconds to t=3 seconds.
1. Determine the position at t=0:
x(0)=acos(π⋅02)=acos(0)=a
The initial position of the particle is a.
2. Determine the position at t=3:
x(3)=acos(π⋅32)=acos(3π2)=a⋅0=0
The position of the particle at t=3 seconds is 0.
3. Analyze the motion:
- The particle starts at position a at t=0.
- It moves to position 0 at t=3.
4. Determine the path taken by the particle:
- From t=0 to t=1: The particle moves from a to 0 (mean position).
- From t=1 to t=2: The particle moves from 0 to −a (the negative extreme).
- From t=2 to t=3: The particle moves from −a back to 0.
5. Calculate the total distance covered:
- Distance from a to 0: a
- Distance from 0 to −a: a
- Distance from −a back to 0: a
Therefore, the total distance covered is:
Total Distance=a+a+a=3a
Final Answer:
The distance covered by the particle in the time interval from t=0 to t=3 seconds is 3a.
---
x(0)=acos(π⋅02)=acos(0)=a
The initial position of the particle is a.
x(3)=acos(π⋅32)=acos(3π2)=a⋅0=0
The position of the particle at t=3 seconds is 0.
- The particle starts at position a at t=0.
- It moves to position 0 at t=3.
- From t=0 to t=1: The particle moves from a to 0 (mean position).
- From t=1 to t=2: The particle moves from 0 to −a (the negative extreme).
- From t=2 to t=3: The particle moves from −a back to 0.
- Distance from a to 0: a
- Distance from 0 to −a: a
- Distance from −a back to 0: a
Total Distance=a+a+a=3a
The distance covered by the particle in the time interval from t=0 to t=3 seconds is 3a.
Topper's Solved these Questions
- OSCILLATIONS AND WAVESBook:PRADEEPChapter:OSCILLATIONS AND WAVESExercise:Long Answer Questions
Explore 33 Videos
- OSCILLATIONS AND WAVESBook:PRADEEPChapter:OSCILLATIONS AND WAVESExercise:Advanced Problems For Competitions
Explore 20 Videos
- OSCILLATIONS AND WAVESBook:PRADEEPChapter:OSCILLATIONS AND WAVESExercise:Very Short Answer Questions
Explore 99 Videos
- MATHEMATICAL TOOLSBook:PRADEEPChapter:MATHEMATICAL TOOLSExercise:Fill in the blanks
Explore 5 Videos
- PHYSICAL WORLD AND MEASUREMENTBook:PRADEEPChapter:PHYSICAL WORLD AND MEASUREMENTExercise:Competiton Focus Jee Medical Entrance
Explore 18 Videos
Similar Questions
A particle moves according to the law x=rcos(πt2). The distance covered by it in the time interval between t = 0 and t = 3 s in nr. What is the value of n?
View SolutionA particle moves along the x axis according to the lawx=acosωt. Find the distance that the particle covers during the time interval from t=0 to t.
View Solution
Knowledge Check
A particle moves according to the law, x=acos(πt/2). . What is the distance covered by it in time interval t=0 to t=3 second.
A2aB3aC4aDaA particle moves according to the equation x=acosπt. The distance covered by it in 2.5 s is
A3aB5aC2aD9aA particle moves according to equation x = A cos πt. The distance covered by it in 3.5 s is
A3AB7ACADzero
A particle moves in y plane according to the law x=4sin6t and y=4(1-cot6t) .The distance traversed by the particle in 4 seconds (in meter) s (x and y are in
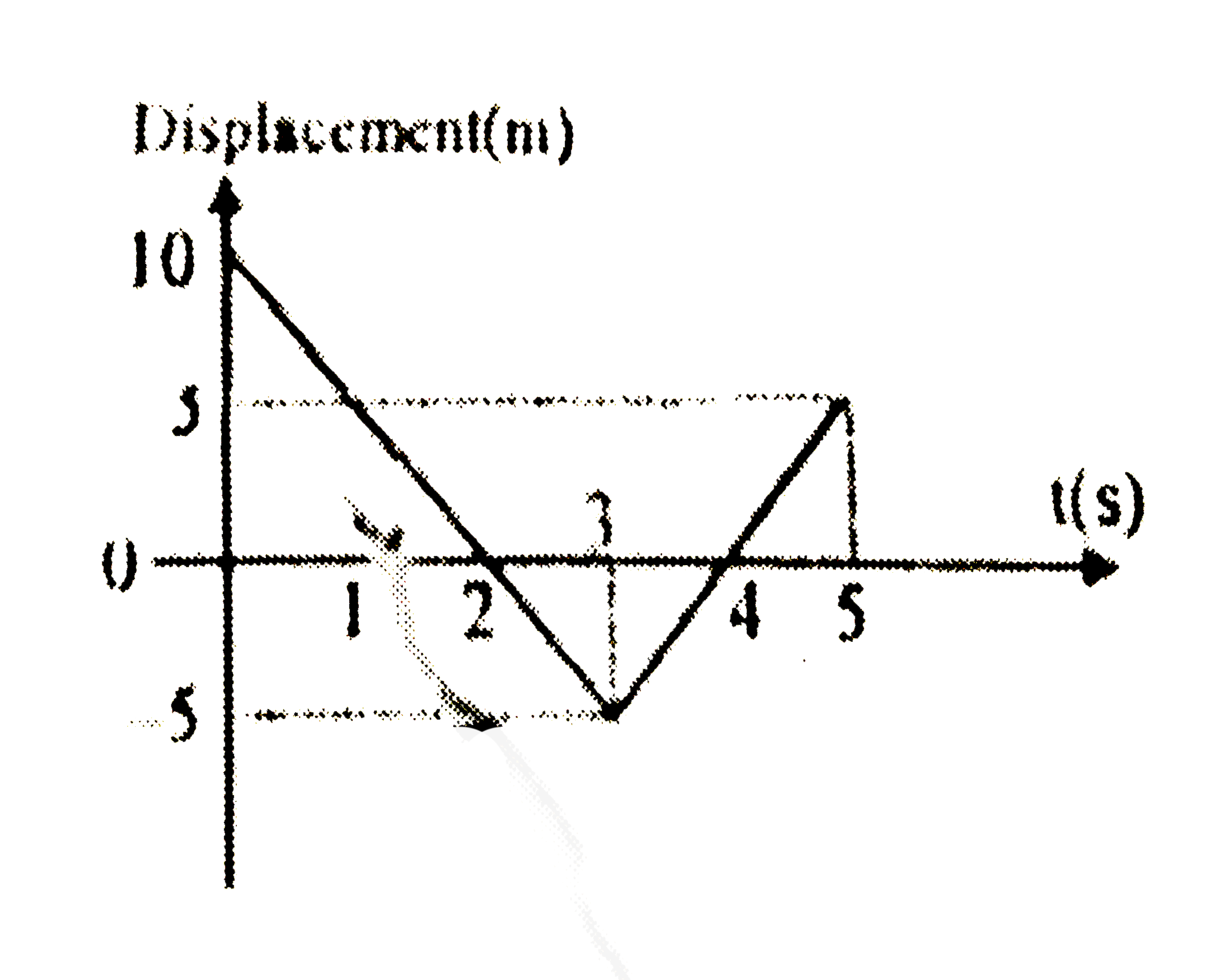
View SolutionThe diagram shows the displacement - time graph for a particle moving in a straight line. The distance (in m) coverted by the particle in the time interval t=0 to t=5s is
View SolutionA particle moves in x−y plane according to the law x=4sin6tandy=4(1−cos6t). The distance traversed by the particle in 4 second is (x&y are in meters)
View SolutionA particle moves along the x - axis according to x=A[1+sinωt]. What distance does is travel in time interval from t=0 to t=2.5π/ω ?
View SolutionA particle moves along x-axis according to the law x=(t3−3t2−9t+5)m. Then :-
View Solution