Question

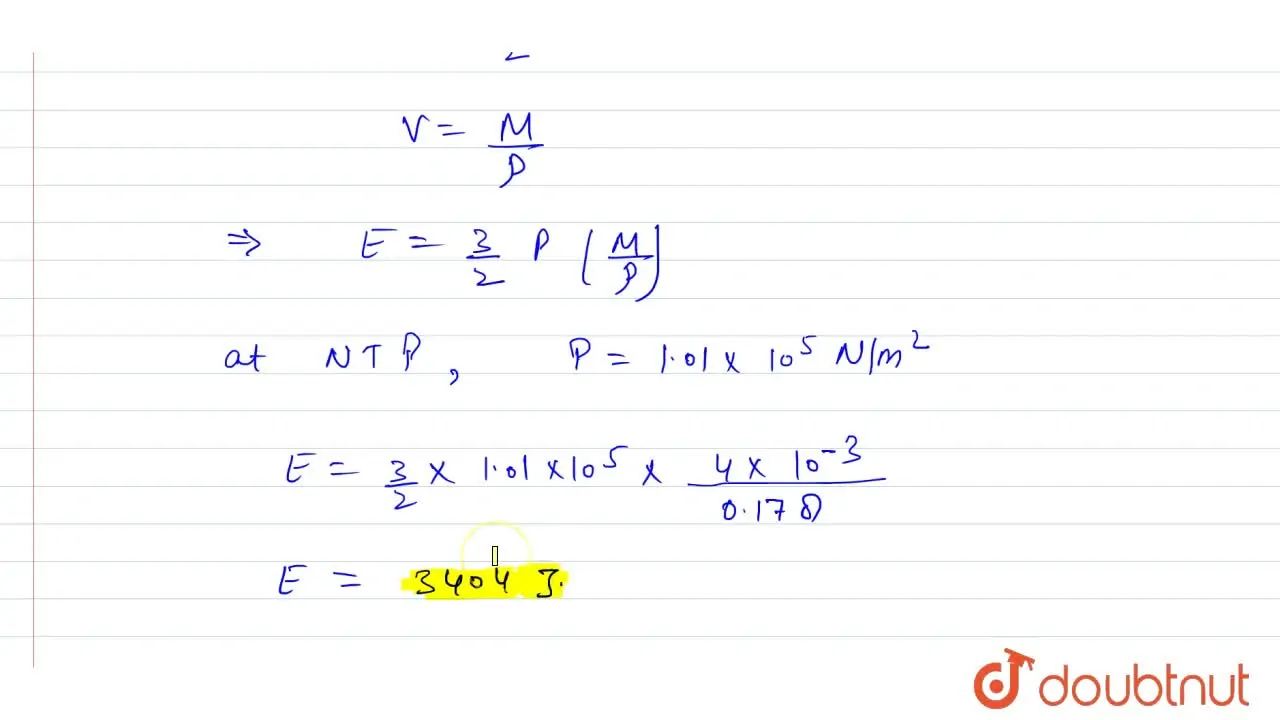
Calculate the kinetic energy of one gram mole of gas at NTP. Density of gas $=0.178kg{m}^{-3}$ at NTP. Its molecular weight = 4. Density of mercury $=13.6\times {10}^{3}kg{m}^{-3}$.

Answer

`3414 J`

Explanation

To calculate the kinetic energy of one gram mole of gas at Normal Temperature and Pressure (NTP), we can follow these steps:

Step 1: Understand the Given Data
- Density of gas, \( \rho = 0.178 \, \text{kg/m}^3 \)
- Molecular weight of gas, \( M = 4 \, \text{g/mol} = 4 \times 10^{-3} \, \text{kg/mol} \)
- Pressure at NTP, \( P = 1.01 \times 10^5 \, \text{N/m}^2 \)

Step 2: Use the Formula for Kinetic Energy
The kinetic energy \( E \) of one mole of an ideal gas can be expressed as:
\( E = \frac{3}{2} RT \)
Where \( R \) is the universal gas constant and \( T \) is the temperature in Kelvin. At NTP, \( T = 273 \, \text{K} \) and \( R = 8.314 \, \text{J/(mol K)} \).

Step 3: Calculate the Kinetic Energy Using Pressure and Density
We can also express the kinetic energy in terms of pressure and volume:
\( E = \frac{3}{2} PV \)
Where \( V \) (volume) can be expressed as:
\( V = \frac{m}{\rho} \)
Here, \( m \) is the mass of one mole of gas, which is \( 4 \times 10^{-3} \, \text{kg} \).

Step 4: Substitute Values into the Kinetic Energy Formula
Substituting \( V \) into the kinetic energy formula:
\( E = \frac{3}{2} P \left(\frac{m}{\rho}\right) \)
Substituting the known values:
\( E = \frac{3}{2} \times (1.01 \times 10^5) \times \left(\frac{4 \times 10^{-3}}{0.178}\right) \)

Step 5: Calculate the Volume
Calculating the volume:
\( V = \frac{4 \times 10^{-3}}{0.178} \approx 0.0225 \, \text{m}^3 \)

Step 6: Calculate the Kinetic Energy
Now substituting back into the equation for kinetic energy:
\( E = \frac{3}{2} \times (1.01 \times 10^5) \times 0.0225 \)
Calculating this gives:
\( E = \frac{3}{2} \times 1.01 \times 10^5 \times 0.0225 \approx 3404 \, \text{J} \)

Final Answer
The kinetic energy of one gram mole of gas at NTP is approximately:
\( E \approx 3404 \, \text{J} \)

---