(a) Pressure decreases as one ascends the atmosphere. If the density of air is ρ, What is the change in pressure dp over a differential height dh ? (b) Considering the pressure p to be proportional to the density, find the pressure p at a height h if the pressure on the sureface of the earth is p0. (c ) If p0=1.03×105Nm−2,ρ0=1.29kgm−3andg=9.8ms−2, at what height will the pressure drop to (1/10) the value at the surface of the earth ? (d) This model of the atmosphere works for relatively small distance. Identify the underlying assumption that limits the model.
(a) Pressure of air due to height h of air is p=hρg Differenting it w.r.t.h, we have dpdh=ρgordp=ρgdh Since pressure of air is decreasing upwards, so dp=−ρgdh (b) Let ρ0 be the density of air on the surface of earth. As per question pressure ∝ density so pp0=ρρ0orρ=ρ0pρ0
Pressure due to height h of air, p=hρg
dp=−ρgdh=−ρ0ρp0×gdh
Here -ve sign shown that pressure decreases with height ∵dpp=−ρ0gρ0g/(p0)dh intergrating it, within the limits as p changes from p0 to p,h changes from 0 to h. Hence
∫pp0dpp=−ρ0gp0∫h0dh
logep−logep0=−ρ0gp0horloge(p)p0=−ρ0gp0h.....(i)
orp=p0e−ρ0gh/p0
(c ) Here, pp0=110,h=h'
From (i), loge(110)=−ρ0gp0h'
h'=−p0ρ0gloge(110)=−1.03×1051.29×9.8×(−2.303)=1.03×1051.29×9.8×2.303=16×103m
(d) The assumption that P∝ρ is applicable only when temperature remains constant, which is true for small distance in air.
Similar Questions
Knowledge Check
The pressure at depth h below the surface of a liquid of density ρ open to the atmosphere is
Agreater than the atmospheric pressure by ρghBless than the atmospheric pressure by ρghCequal to the atmospheric pressureDincreases exponentially with depthIt P is the pressure and ρ is the density of a gas, then P and ρ are realted as :
AP∝ρBP∝ρ2CP∝1/ρDP∝1/ρ2If P is the pressure of a gas and ρ is its density , then find the dimension of velocity in terms of P and ρ .
AP1/2ρ−1/2BP1/2ρ1/2CP−1/2ρ−1/2DP−1/2ρ−1/2
The density of air at one atmospheric pressure is 0.9kgm−3 What will be its density at 4 atmospheric pressure,when temperature remains constant?
View SolutionA small air bubble of radius r in water is at depth h below the water surface. If P0 is the atmospheric pressure, ρ is the density of water and T is the surface tension of water, what is the pressure inside the bubble?
View SolutionIf the atompspheric pressure is Pa then the jpressure P at depth a below the surface of a liquid of density ρ open to the atmosphere is
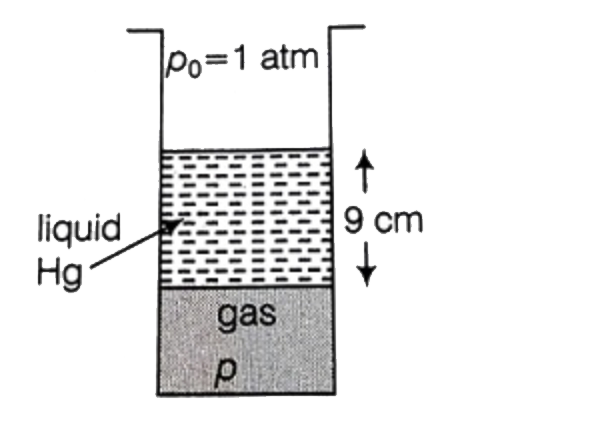
View SolutionIn the given figure, atmospheric pressure p0=1 atm and mercury column length is 9 cm. Pressure p of the gas enclosed in the tube is
View SolutionA beaker is filled with a liquid of density ρ upto a height h If the beaker is at rest, the mean pressure at the walls is:
View Solution

