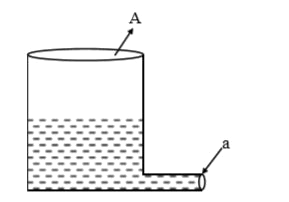
A light cylindrical vessel is kept on a horizontal surface. Its base area is A. a hole of cross sectional area a is made just at its bottom side (where a less than A). Find minimum coefficeint of friction necessary for sliding of the vesssel due to the impact force of the emerging liquid.

Let h be the height of liquid in vessel above the hole.
Velocity of efflux, υ=√2gh
Impact force of the imerging liquid on the vessel and liquid contents is
F=υdmdt=υ(aρυ)=aρυ2=aρ2gh
=2aρgh
The force of friction, f=μR=μAhρg
For just sliding the vessel , f=F
so, μAhρg=2aρghorμ=2aA.
Topper's Solved these Questions
- PROPERTIES OF BULK MATTERBOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - NCERT Questions
73 Videos
- PROPERTIES OF BULK MATTERBOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Higher Order thinking skells
17 Videos
- PROPERTIES OF BULK MATTERBOOK - PRADEEPCHAPTER - PROPERTIES OF BULK MATTEREXERCISE - Conceptual Problems
103 Videos
- PHYSICAL WORLD AND MEASUREMENTBOOK - PRADEEPCHAPTER - PHYSICAL WORLD AND MEASUREMENTEXERCISE - Competiton Focus Jee Medical Entrance
18 Videos
- RAY OPTICSBOOK - PRADEEPCHAPTER - RAY OPTICSEXERCISE - Problem For Practice(a)
25 Videos
Similar Questions
A light cylindrical vessel is kept on a horizontal surface. Its base area is A. a hole of cross sectional area a is made just at its bottom sdie (where a ltlt A). Find minimum coefficeint of friction necessary for sliding of the vesssel due to the impact force of the emerging liquid.

View SolutionA light cylindrical vessel is kept on a horizontal surface. Its base area is A. a hole of cross sectional area a is made just at its bottom side (where a less than A). Find minimum coefficeint of friction necessary for sliding of the vesssel due to the impact force of the emerging liquid.

View Solution
Knowledge Check
A light cylindrical vessel is kept on a horizontal surface it's base area is A. A hole of cross-sectional area a is made just at it's bottom side. The minimum coefficient of friction necessary for not sliding of vessel dueto the impact force of the emerging liquid.
AvaryingBaAC2aADnoneA large cylindrical tank has a hole of area A at its bottom. Water is oured in the tank by a tube of equal cross sectional area A ejecting water at the speed v.
Athe water level in the tank wil keep on rising.Bno water an be stored in the tankCthe water level will rise to a height v22g and then stopDthe water level will oscillate.A large container (with open top) of negligible mass and uniform cross-sectional area A has a small hole of cross-sectional area a in its side wall near the bottom. The container contains a liquid of density ρ and mass m. If the liquid starts flowing out of the hole at time t = 0, the velocity of efflux of the liquid when 75% of the liquid has drained out is:
A√3mg4AρB√2mgAρC2√mgAρD√mg2Aρ
A light cylindrical vessel is kept on a horizontal surface. Area of base is A. A hole of crosssectional area 'a' is made just at its bottom side. The minimum coefficient of friction necessary to prevent sliding the vessel due to the impact force of the emerging liquid is (a<<A):
View SolutionA cylindrical vessel of area of cross section A is filled with a liquid up to a height H. A very small hole of area of cross section a is made at the bottom of the vellel. Find the time taken by the vessel to become empty.
View SolutionA rod of length L kept on a smooth horizontal surface is pulled along its length by a force F. The area of cross-section is A and Young's modulus is Y. The extension in the rod is

View SolutionA cylindrical tank of cross sectional area a1 is filled with water to a height h. A hole of cross sectional area a2 is made at the bottom. If a1=5a2, then find the
(i) initial velocity with which the water falls in tank.
(ii) initial velocity with which water emerges out from hole.
(iii) time taken to make the tank empty.
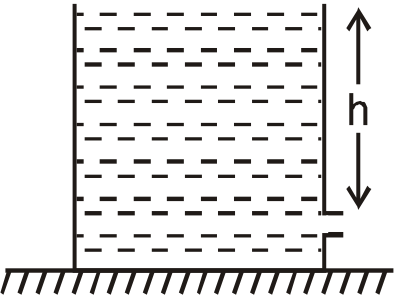
View SolutionIn the figure shown, a light container is kept on a horizontal rough surface of coefficient of friction μ=ShV. A very small hole of area S is made at depth 'h'. Water of volume 'V' is filled in the container. The friction is not sufficient to keep the container at rest. The acceleration of the container initially is
View Solution
