Statement: The addition of a small amount of a 'neutral' electrolyte (one that does not share a common ion) such as NaCI to a dilute solution of acetic acid, will cause an increase in the degree of dissociation of the acid.
Explanation: Due to the increased ionic strength, the mean ionic activity coefficient of H3O+ and CH3COO− will increase.
S is correct but E is wrong.
S is wrong but E is correc.
Both S and E are correct and E is correct explanation of S.
Both S and E are correct but E is correct explanation of S.
The correct Answer is:C
Explanation is correct reason for statement.
Topper's Solved these Questions
- IONIC EQUILIBRIUMBOOK - P BAHADURCHAPTER - IONIC EQUILIBRIUMEXERCISE - Exercise7
33 Videos
- GASEOUS STATEBOOK - P BAHADURCHAPTER - GASEOUS STATEEXERCISE - Exercise -9
1 Videos
- MOLE AND EQUIVALENT CONCEPTBOOK - P BAHADURCHAPTER - MOLE AND EQUIVALENT CONCEPTEXERCISE - Exercise 9 Advanced numerical problems
61 Videos
Similar Questions
Read the given passage and answer the questions number that follow :
Weak electrolytes like acetic acid have lower degree of dissociation at higher concentrations and hence for such electrolytes, the change in Λm with dilution is due to increase in the degree of dissociation and consequently the number of ions in total volume of solution that contains one mol of electrolyte. In such cases Λm increases steeply on dilution, especially near lower concentrations. Therefore, Λm cannot be obtained by extrapolation of Λm to zero concentration. At infinite dilution (i.e., concentration c → zero) electrolyte dissociates completely (α = 1), but at such low concentration the conductivity of the solution is so low that it cannot be measured accurately. Therefore, Λ∘m for weak electrolytes is obtained by using Kohlrausch law of independence migration of ions.
Why does the change in Λm take place on dilution in case of weak electrolytes ?
View SolutionRead the given passage and answer the questions number that follow :
Weak electrolytes like acetic acid have lower degree of dissociation at higher concentrations and hence for such electrolytes, the change in Λm with dilution is due to increase in the degree of dissociation and consequently the number of ions in total volume of solution that contains one mol of electrolyte. In such cases Λm increases steeply on dilution, especially near lower concentrations. Therefore, Λm cannot be obtained by extrapolation of Λm to zero concentration. At infinite dilution (i.e., concentration c → zero) electrolyte dissociates completely (α = 1), but at such low concentration the conductivity of the solution is so low that it cannot be measured accurately. Therefore, Λ∘m for weak electrolytes is obtained by using Kohlrausch law of independence migration of ions.
Why does the change in Am take place on dilution in case of weak electrolytes ?
View Solution
Knowledge Check
The degree of dissociation of 0.1 M weak acid HA is 0.5%. If 2 mL of 1.0MHA solution is diluted to 32 mL the degree of dissociation of acid and H3O+ ion concentration in the resulting solution will be respectively
A0.02and3.125×10−4B1.25×10−3and0.02C6.02and1.25×10−3D0.02and8.0×10−12
Strong acid versus strong base:
The principle of conductometric titrations is based on the fact that during the titration, one of the ions is replaced by the other and invariable these two ions differ in the ionic conductivity with the result that thhe conductivity of the solution varies during the course of the titration. take, for example, the titration between a strong acid, say HCl, and a string base, say NaOH before NaOH is added, the conductance of HCl solution has a high value due to the presence of highly mobile hydrogen ions. As NaOH is added, H+ ions are replaced by relatively slower moving Na+ ions. consequently the conductance of the solution decreases and this continues right upto the equivalence point where the solution contains only NaCl. Beyond the equivalence point, if more of NaOH is added, then the solution contains a excess of the fast moving OH− ions with the result that its conductance is increased ad it condinues to increase as more and more of NaOH is added. If we plot the conductance value versus the amount of NaOH added, we get a curve of the type shown in Fig. The descending portion AB represents the conductances before the equivalence point (solution contains a mixture of acid HCl and the salt NaCl) and the ascending portion CD represents the conductances after the equivalence point (solution contains the salt NaCl and the excess of NaOH). The point E which represent the minium conductance is due to the solution containing only NaCl with no free acid or alkali and thus represents the equivalence point. this point can, however, be obtained by the extrapolation of the lines AB and DC, and therefore, one is not very particular in locating this point expermentally as it is in the case of ordinary acid-base titrations involving the acid-base indicators.
Weak acid versus strong base:
Let us take specific example of acetic acid being titrated against NaOH. Before the addition of alkali, the solution shows poor conductance due to feeble ionization of acetic acid. Initially the addition of alkali causes not only the replacement of H+ by Na+ but also suppresses the dissociation of acetic acid due to the common ion Ac− and thus the conductance of the solution decreases in the beginning. but very soon the conductance start increasing as addition of NaOH neutralizes the undissociated HAc to Na+Ac− thus causing the replacement of non-conducting HAc with Strong-conducting electrolyte Na+Ac−. the increase in conductance continuous right up to the equivalence point. Beyond this point conductance increases more rapidly with the addition of NaOH due to the highly conducting OH− ions, the graph near the equivalence point is curved due to the hydrolysis of the salt NaAc. The actual equivalence point can, as usual, be obtained by the extrapolation method.
In all these graphs it has been assumed that the volume change due addition of solution from burrette is negnigible, hence volume change of the solution in beaker the conductance of which is measured is almost constant throughout the measurement.
Q. The nature of curve obtained for the titration between weak acid versus strong base as described in the above passage will be:A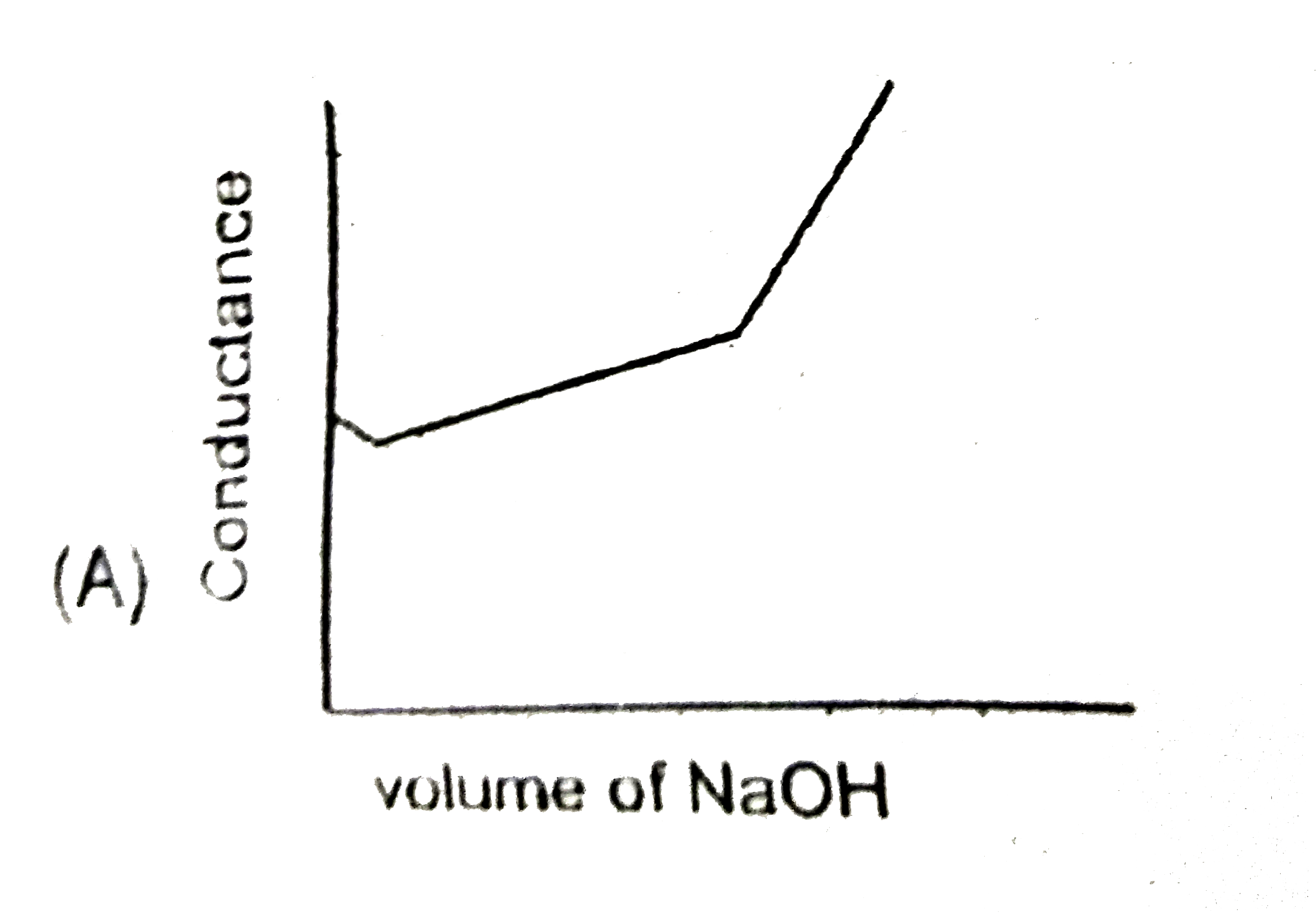 B
B C
C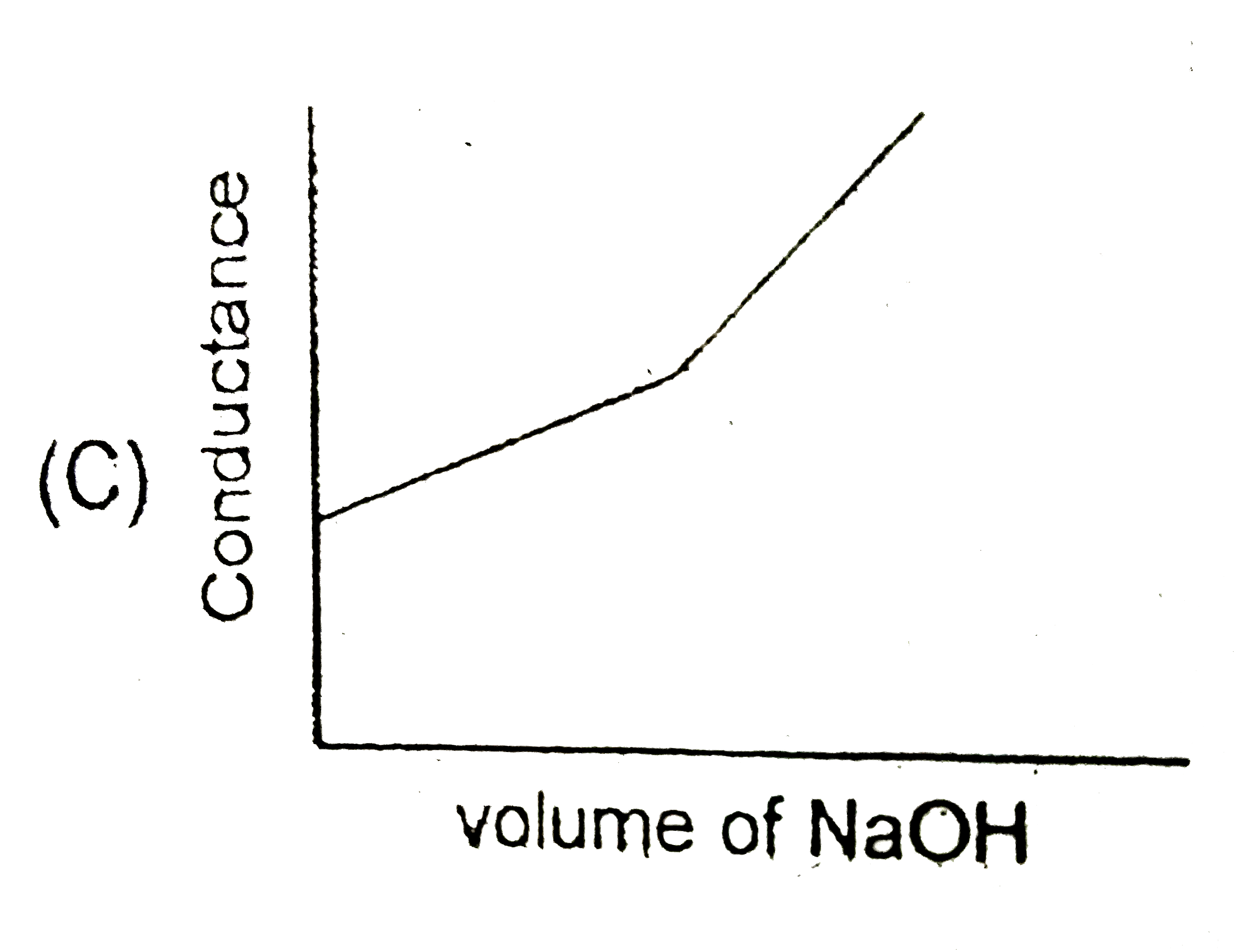 D
D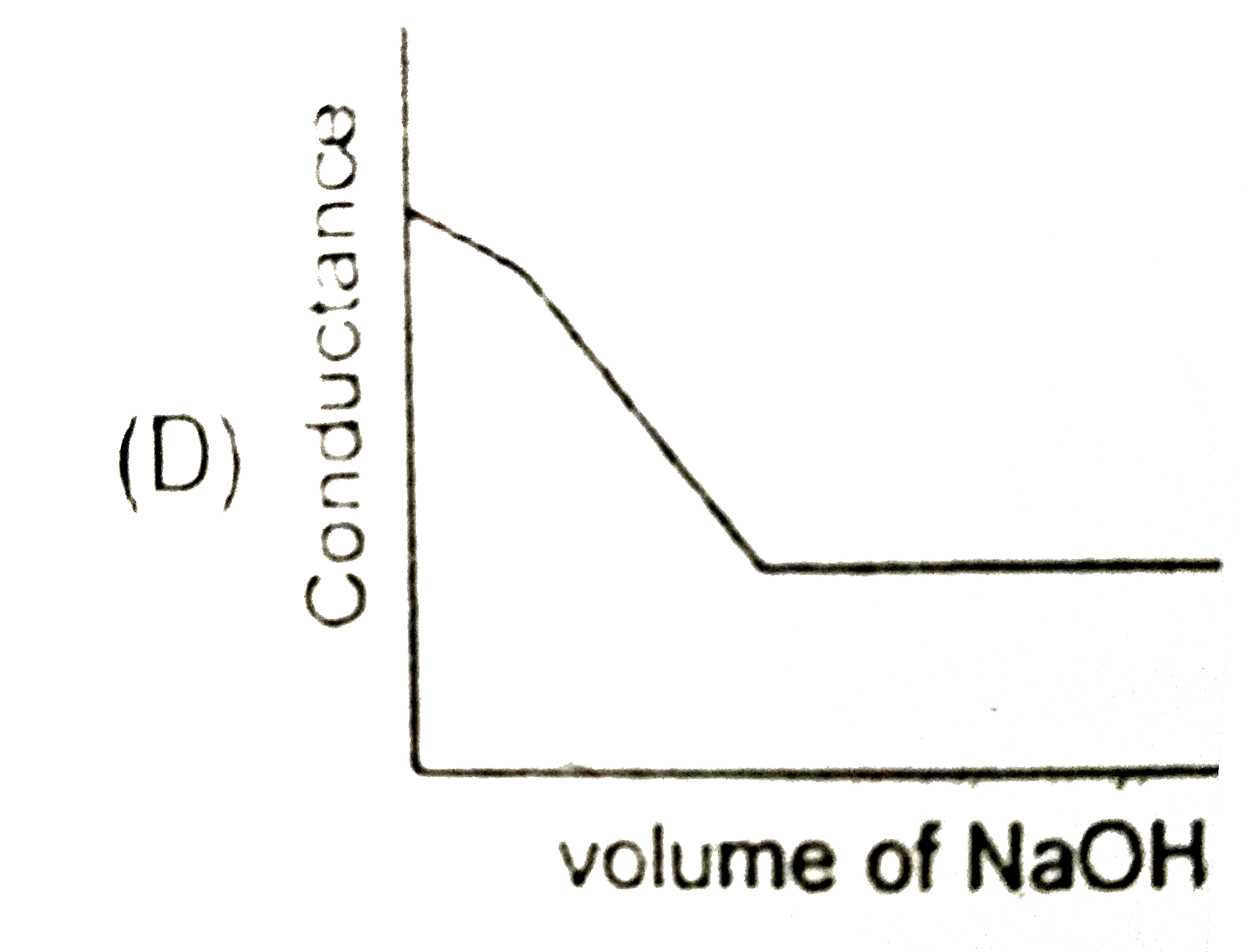

Strong acid versus strong base:
The principle of conductometric titrations is based on the fact that during the titration, one of the ions is replaced by the other and invariable these two ions differ in the ionic conductivity with the result that thhe conductivity of the solution varies during the course of the titration. take, for example, the titration between a strong acid, say HCl, and a string base, say NaOH before NaOH is added, the conductance of HCl solution has a high value due to the presence of highly mobile hydrogen ions. As NaOH is added, H+ ions are replaced by relatively slower moving Na+ ions. consequently the conductance of the solution decreases and this continues right upto the equivalence point where the solution contains only NaCl. Beyond the equivalence point, if more of NaOH is added, then the solution contains a excess of the fast moving OH− ions with the result that its conductance is increased ad it condinues to increase as more and more of NaOH is added. If we plot the conductance value versus the amount of NaOH added, we get a curve of the type shown in Fig. The descending portion AB represents the conductances before the equivalence point (solution contains a mixture of acid HCl and the salt NaCl) and the ascending portion CD represents the conductances after the equivalence point (solution contains the salt NaCl and the excess of NaOH). The point E which represent the minium conductance is due to the solution containing only NaCl with no free acid or alkali and thus represents the equivalence point. this point can, however, be obtained by the extrapolation of the lines AB and DC, and therefore, one is not very particular in locating this point expermentally as it is in the case of ordinary acid-base titrations involving the acid-base indicators.
Weak acid versus strong base:
Let us take specific example of acetic acid being titrated against NaOH. Before the addition of alkali, the solution shows poor conductance due to feeble ionization of acetic acid. Initially the addition of alkali causes not only the replacement of H+ by Na+ but also suppresses the dissociation of acetic acid due to the common ion Ac− and thus the conductance of the solution decreases in the beginning. but very soon the conductance start increasing as addition of NaOH neutralizes the undissociated HAc to Na+Ac− thus causing the replacement of non-conducting HAc with Strong-conducting electrolyte Na+Ac−. the increase in conductance continuous right up to the equivalence point. Beyond this point conductance increases more rapidly with the addition of NaOH due to the highly conducting OH− ions, the graph near the equivalence point is curved due to the hydrolysis of the salt NaAc. The actual equivalence point can, as usual, be obtained by the extrapolation method.
In all these graphs it has been assumed that the volume change due addition of solution from burrette is negnigible, hence volume change of the solution in beaker the conductance of which is measured is almost constant throughout the measurement.
Q. The most appropriate titration curve obtained when a mixture of a strong acid (say HCl) and a weak acid (say CH3COOH) is titrated with a strong base (say NaOH) will beA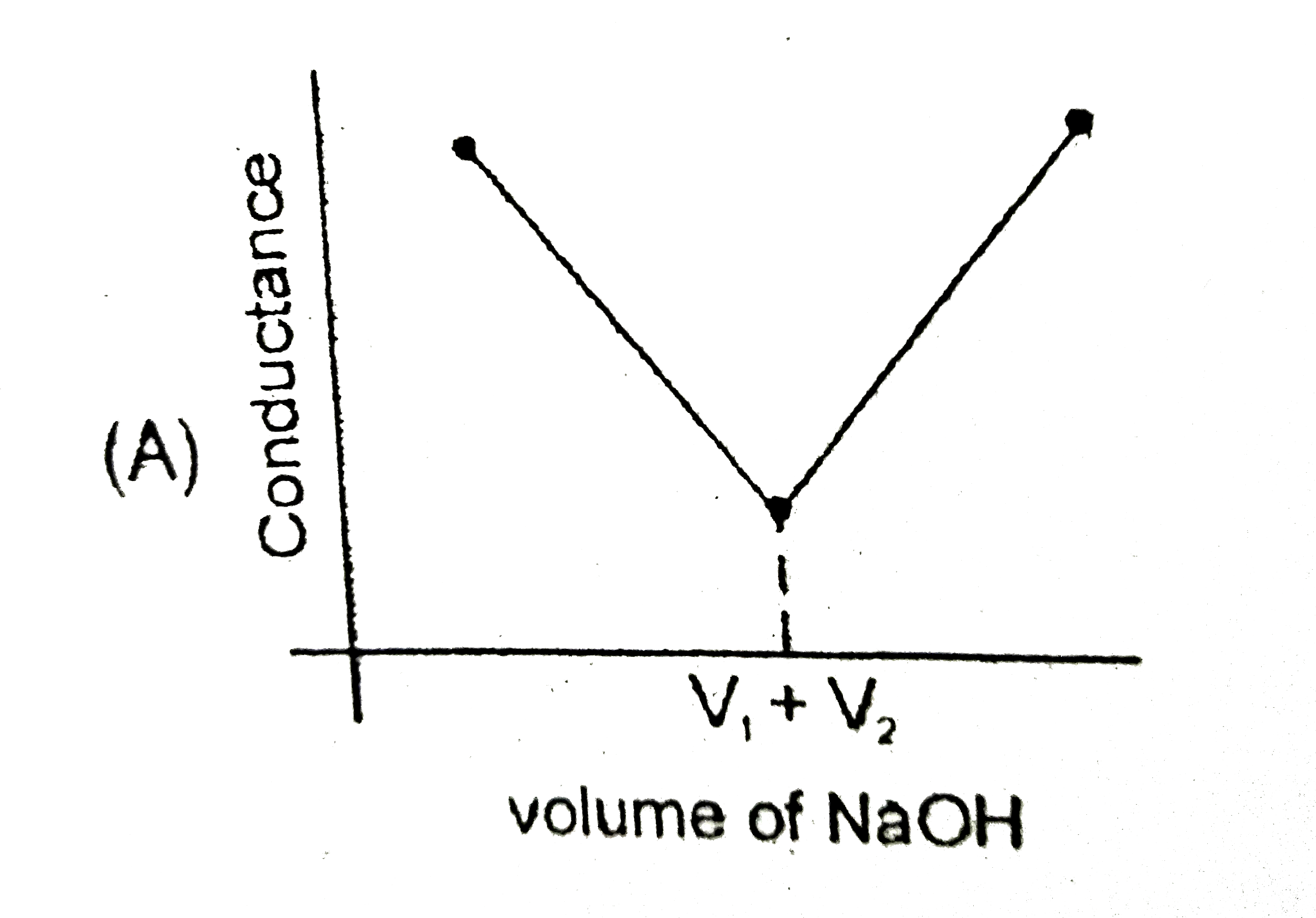 B
B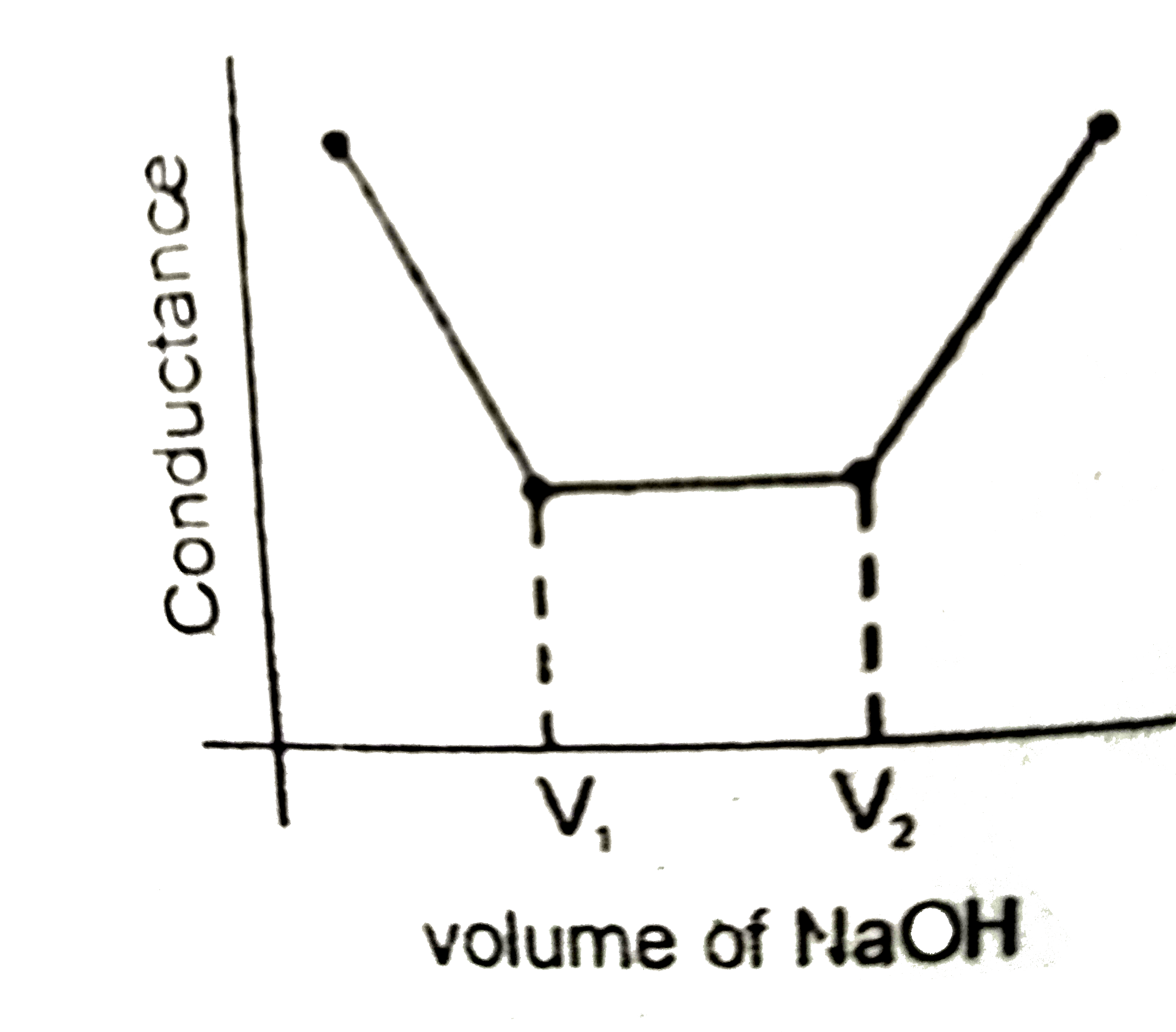 C
C D
D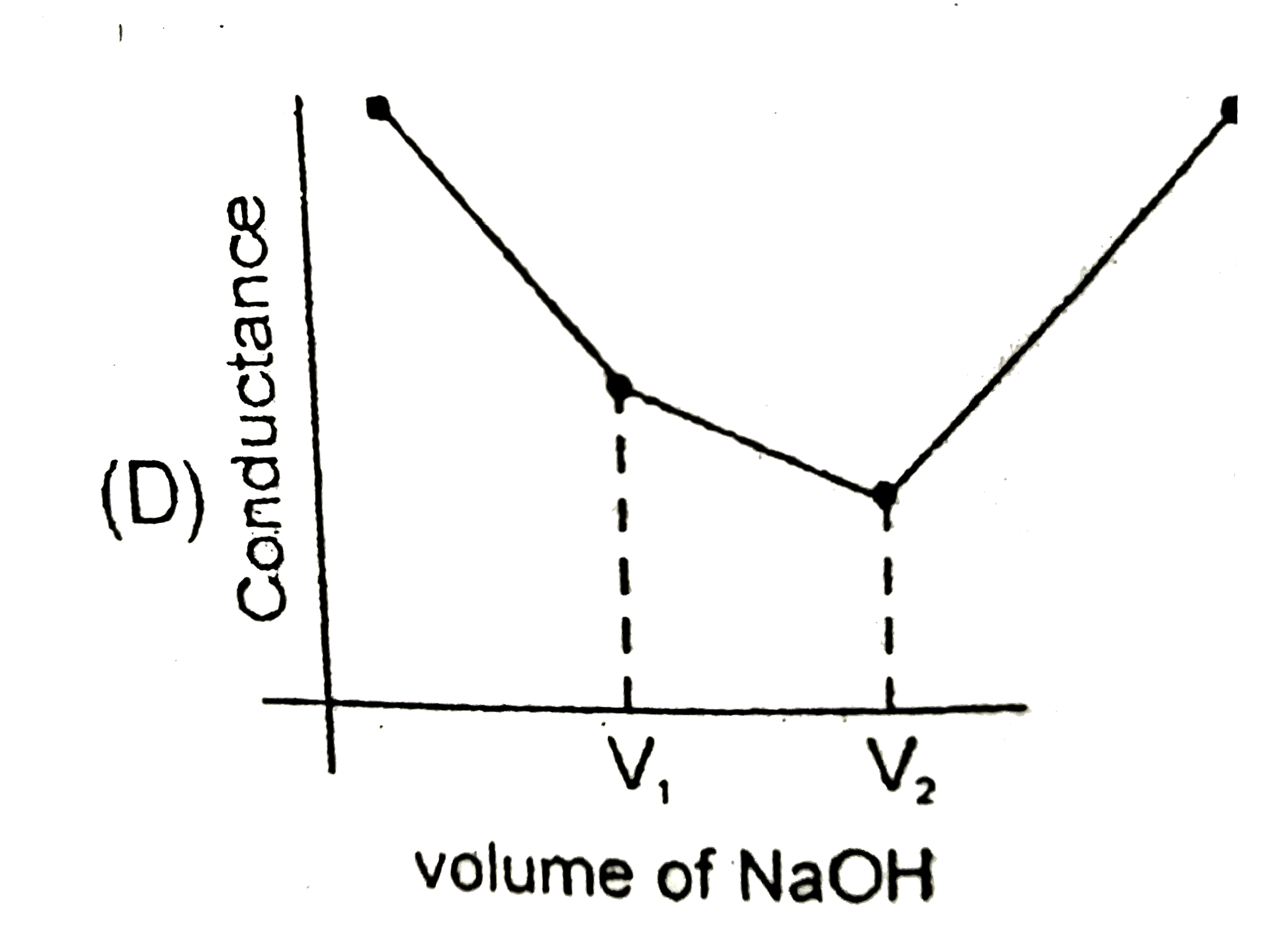
Read the given passage and answer the questions number that follow :
Weak electrolytes like acetic acid have lower degree of dissociation at higher concentrations and hence for such electrolytes, the change in Λm with dilution is due to increase in the degree of dissociation and consequently the number of ions in total volume of solution that contains one mol of electrolyte. In such cases Λm increases steeply on dilution, especially near lower concentrations. Therefore, Λm cannot be obtained by extrapolation of Λm to zero concentration. At infinite dilution (i.e., concentration c → zero) electrolyte dissociates completely (α = 1), but at such low concentration the conductivity of the solution is so low that it cannot be measured accurately. Therefore, Λ∘m for weak electrolytes is obtained by using Kohlrausch law of independence migration of ions.
Give the mathematical relation to calculate the degree of dissociation, α for a weak electrolyte.
View SolutionRead the given passage and answer the questions number that follow :
Weak electrolytes like acetic acid have lower degree of dissociation at higher concentrations and hence for such electrolytes, the change in Λm with dilution is due to increase in the degree of dissociation and consequently the number of ions in total volume of solution that contains one mol of electrolyte. In such cases Λm increases steeply on dilution, especially near lower concentrations. Therefore, Λm cannot be obtained by extrapolation of Λm to zero concentration. At infinite dilution (i.e., concentration c → zero) electrolyte dissociates completely (α = 1), but at such low concentration the conductivity of the solution is so low that it cannot be measured accurately. Therefore, Λ∘m for weak electrolytes is obtained by using Kohlrausch law of independence migration of ions.
Which law is used lo calculate Λ∘m for weak electrolyles ?
View SolutionRead the given passage and answer the questions number that follow :
Weak electrolytes like acetic acid have lower degree of dissociation at higher concentrations and hence for such electrolytes, the change in Λm with dilution is due to increase in the degree of dissociation and consequently the number of ions in total volume of solution that contains one mol of electrolyte. In such cases Λm increases steeply on dilution, especially near lower concentrations. Therefore, Λm cannot be obtained by extrapolation of Λm to zero concentration. At infinite dilution (i.e., concentration c → zero) electrolyte dissociates completely (α = 1), but at such low concentration the conductivity of the solution is so low that it cannot be measured accurately. Therefore, Λ∘m for weak electrolytes is obtained by using Kohlrausch law of independence migration of ions.
Why can't we obtain Λ∘m by extrapolation of Λm to zero concentration ?
View Solution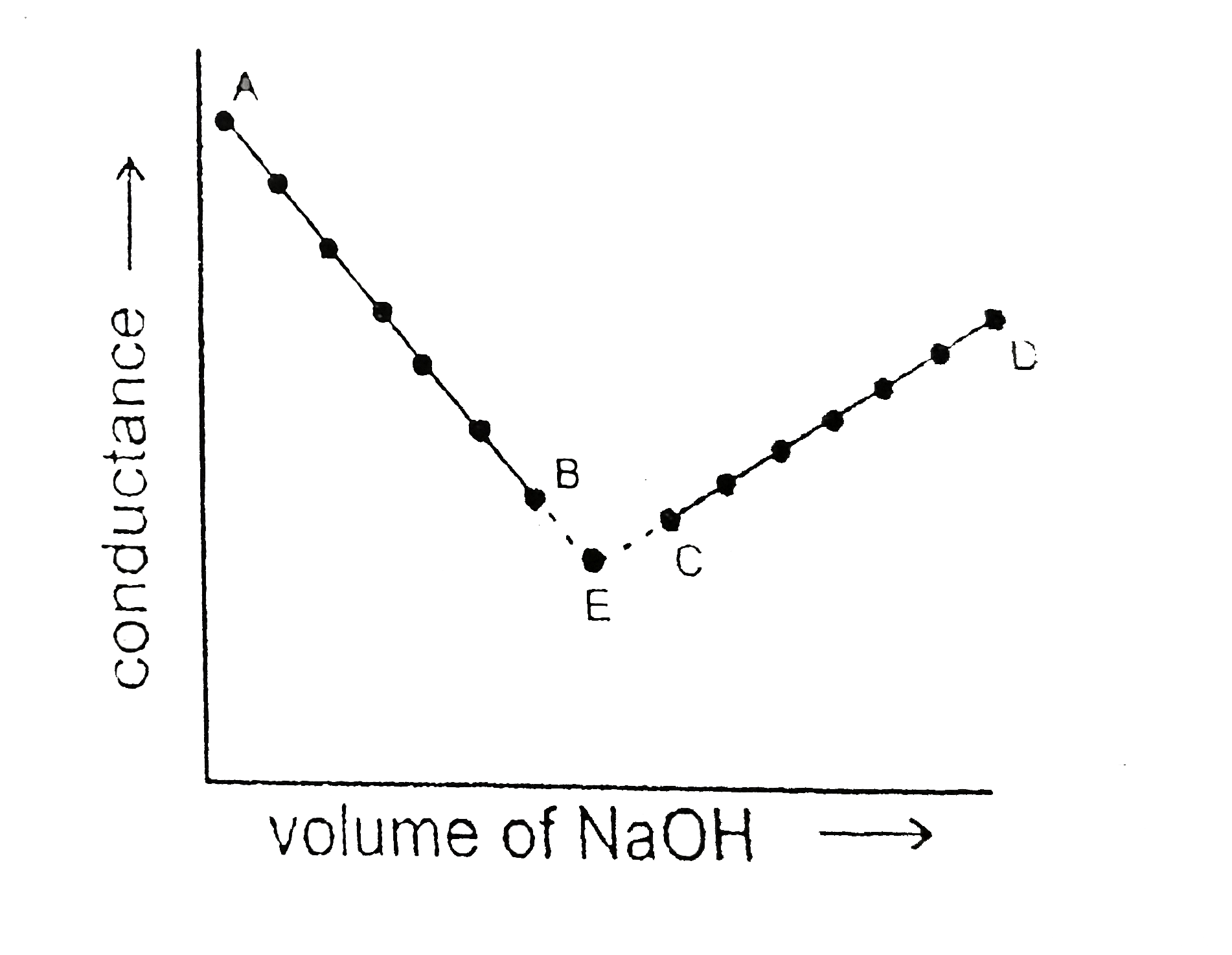
Strong acid versus strong base:
The principle of conductometric titrations is based on the fact that during the titration, one of the ions is replaced by the other and invariable these two ions differ in the ionic conductivity with the result that thhe conductivity of the solution varies during the course of the titration. take, for example, the titration between a strong acid, say HCl, and a string base, say NaOH before NaOH is added, the conductance of HCl solution has a high value due to the presence of highly mobile hydrogen ions. As NaOH is added, H+ ions are replaced by relatively slower moving Na+ ions. consequently the conductance of the solution decreases and this continues right upto the equivalence point where the solution contains only NaCl. Beyond the equivalence point, if more of NaOH is added, then the solution contains a excess of the fast moving OH− ions with the result that its conductance is increased ad it condinues to increase as more and more of NaOH is added. If we plot the conductance value versus the amount of NaOH added, we get a curve of the type shown in Fig. The descending portion AB represents the conductances before the equivalence point (solution contains a mixture of acid HCl and the salt NaCl) and the ascending portion CD represents the conductances after the equivalence point (solution contains the salt NaCl and the excess of NaOH). The point E which represent the minium conductance is due to the solution containing only NaCl with no free acid or alkali and thus represents the equivalence point. this point can, however, be obtained by the extrapolation of the lines AB and DC, and therefore, one is not very particular in locating this point expermentally as it is in the case of ordinary acid-base titrations involving the acid-base indicators.
Weak acid versus strong base:
Let us take specific example of acetic acid being titrated against NaOH. Before the addition of alkali, the solution shows poor conductance due to feeble ionization of acetic acid. Initially the addition of alkali causes not only the replacement of H+ by Na+ but also suppresses the dissociation of acetic acid due to the common ion Ac− and thus the conductance of the solution decreases in the beginning. but very soon the conductance start increasing as addition of NaOH neutralizes the undissociated HAc to Na+Ac− thus causing the replacement of non-conducting HAc with Strong-conducting electrolyte Na+Ac−. the increase in conductance continuous right up to the equivalence point. Beyond this point conductance increases more rapidly with the addition of NaOH due to the highly conducting OH− ions, the graph near the equivalence point is curved due to the hydrolysis of the salt NaAc. The actual equivalence point can, as usual, be obtained by the extrapolation method.
In all these graphs it has been assumed that the volume change due addition of solution from burrette is negnigible, hence volume change of the solution in beaker the conductance of which is measured is almost constant throughout the measurement.
Q. If a 100 mL solution of 0.1 M HBr is titrated using a very concentrated solution of NaOH, then the conductivity (specific conductance) of this solution at the equivalence point will be (assume volume change is negligible due to addition of NaoH) report your answer after multipling it with 10 in Sm−1
[Given λ∘(Na+)=8×10−3Sm2mol−1,λ∘(Br−)=4×10−3Sm2mol−1]
View SolutionWhich of the following combinations regarding True(T) and False (F) nature of following statement is correct ?
Statement-1: pH of water at any temperature will be 7.
Statement-2:Acid dissociation constant of water at 298 K is equal to 10−14
Statement-3:For any aqueous acid solution (pH+pOH) will be equal to 14 at 298 K
Statement-4:Degree of dissociation of water at 308 K will be greater than 1.6×10−7%
Statement-5: On increasing dilution extent of dissociation of HCl will increase.
View Solution
