The relation between the linear magnification m, the object distance u and the focal length f is
m=f−uf
m=fm−u
m=f+uf
m=ff+u
The correct Answer is:B
To derive the relation between linear magnification m, object distance u, and focal length f, we can follow these steps:
1. Understand the Definitions:
- The linear magnification m is defined as the ratio of the height of the image h′ to the height of the object h, given by:
m=h′h=−vu
where v is the image distance and u is the object distance.
2. Use the Lens Formula:
- The lens formula relates the object distance u, image distance v, and focal length f:
1f=1u+1v
3. Express v in terms of m and u:
- From the magnification formula, we can express v:
v=−mu
4. Substitute v into the Lens Formula:
- Substitute v=−mu into the lens formula:
1f=1u+1−mu
5. Simplify the Equation:
- This can be simplified as follows:
1f=1u−1mu
- Combine the fractions:
1f=m−1mu
6. Rearranging for m:
- Rearranging gives:
1m=uf⋅1m−1
- Inverting the equation yields:
m=ff−u
Final Relation:
The final relation between linear magnification m, object distance u, and focal length f is:
m=ff−u
- The linear magnification m is defined as the ratio of the height of the image h′ to the height of the object h, given by:
m=h′h=−vu
where v is the image distance and u is the object distance.
- The lens formula relates the object distance u, image distance v, and focal length f:
1f=1u+1v
- From the magnification formula, we can express v:
v=−mu
- Substitute v=−mu into the lens formula:
1f=1u+1−mu
- This can be simplified as follows:
1f=1u−1mu
- Combine the fractions:
1f=m−1mu
- Rearranging gives:
1m=uf⋅1m−1
- Inverting the equation yields:
m=ff−u
The final relation between linear magnification m, object distance u, and focal length f is:
m=ff−u
Topper's Solved these Questions
- GEOMETRICAL OPTICSBook:A2ZChapter:GEOMETRICAL OPTICSExercise:Refraction At Curved Surface
Explore 64 Videos
- GEOMETRICAL OPTICSBook:A2ZChapter:GEOMETRICAL OPTICSExercise:Prism Theory And Dispersion Of Light
Explore 45 Videos
- ELECTROMAGNETIC WAVES AND COMMUNICATION SYSTEM Book:A2ZChapter:ELECTROMAGNETIC WAVES AND COMMUNICATION SYSTEM Exercise:Section D - Chapter End Test
Explore 30 Videos
- MAGNETISM AND MATTERBook:A2ZChapter:MAGNETISM AND MATTERExercise:Section D - Chapter End Test
Explore 30 Videos
Similar Questions
The distance between the object and the real image formed by a convex lens is d. If the linear magnification is m, find the focal length of the lens in terms of d and m.
View SolutionAn object is placed at a distance of 1.5m from a screen and a convex lens is interposed between them. The magnification produced is 4. What is the focal length of the lens ?
View Solution
Knowledge Check
The relation between the linear magnification m, the object distance u and the focal length f is
Am=f−ufBm=fm−uCm=f+ufDm=ff+uThe magnifications produced by a convex lens for two different positions of an object are m1 and m2 respectively (m1>m2). If 'd' is the distance of separation between the two positions of the object then the focal length of the lens is
A√m1m2Bd√m1−m2Cdm1m2m1−m2Ddm1−m2A spherical mirror forms an image of magnification 3. The object distance, if focal length of mirror is 24cm, may be
A32cm,24cmB32cm,16cmC32cm onlyD16cm only
An object is placed at a distance of 1.5m from a screen and a convex lens is interposed between them. The magnification produced is 4. What is the focal length of the lens ?
View SolutionAn astronomical telescope under normal adjustment produces a magnification of 50 . If the objective has the focal length of 1 m , find the focal length of the eyepiece and the length of the telescope .
View SolutionA spherical mirror forms an image of magnification3. The object distance, if focal length of mirror is 24cm, may be
View SolutionThe following graph depicts the inverse of magnification versus the distance between the object and lens data for a setup. The focal length of the lens used in the setup is
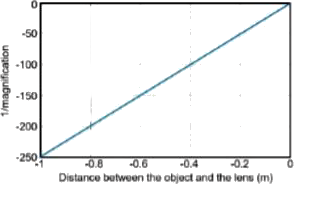
View SolutionThe graph in Fig. shows how the inverse of magnification 1/m produced by a convex thin lens varies with object distance u. What was the focal length of the lens used ?
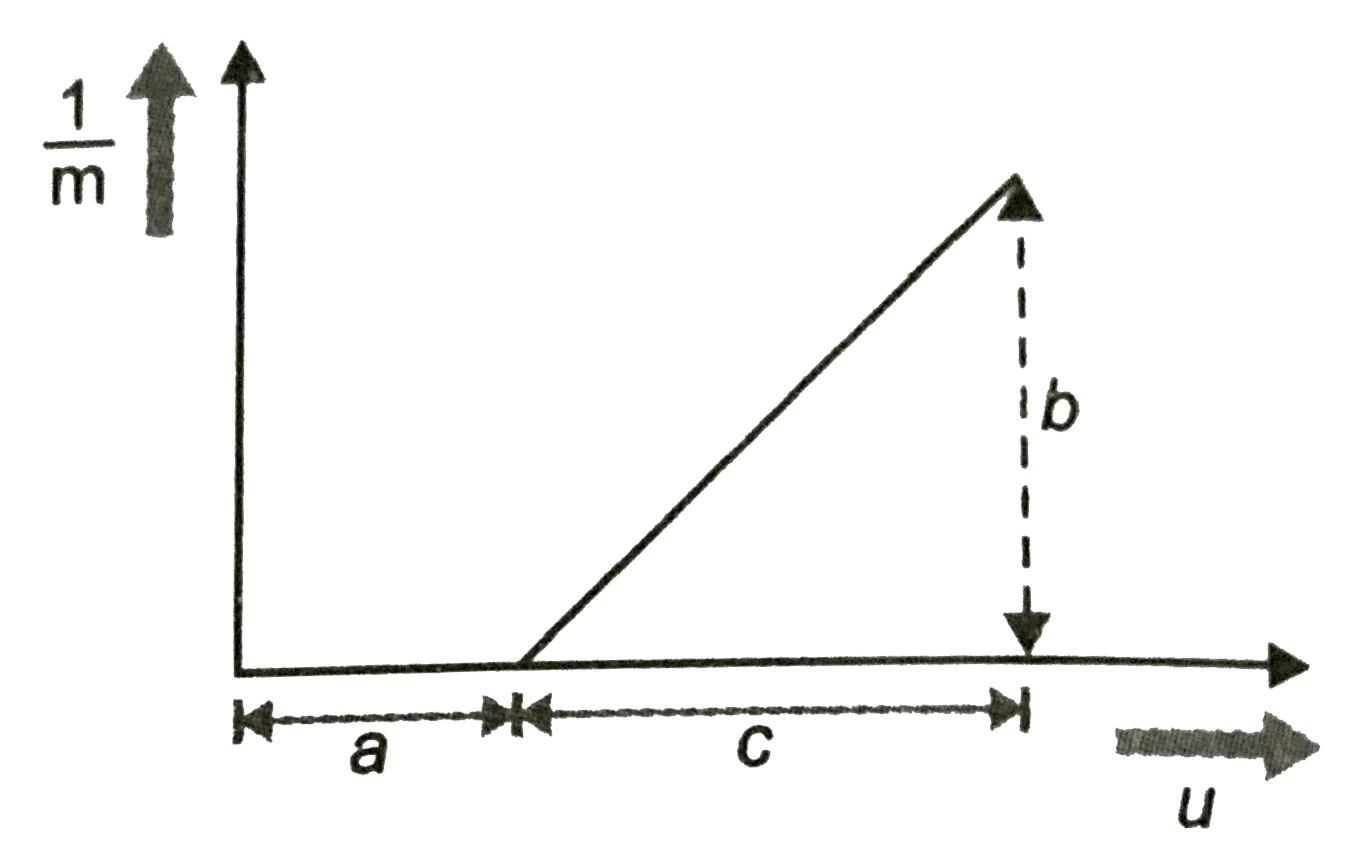 .
.
View Solution
