Question

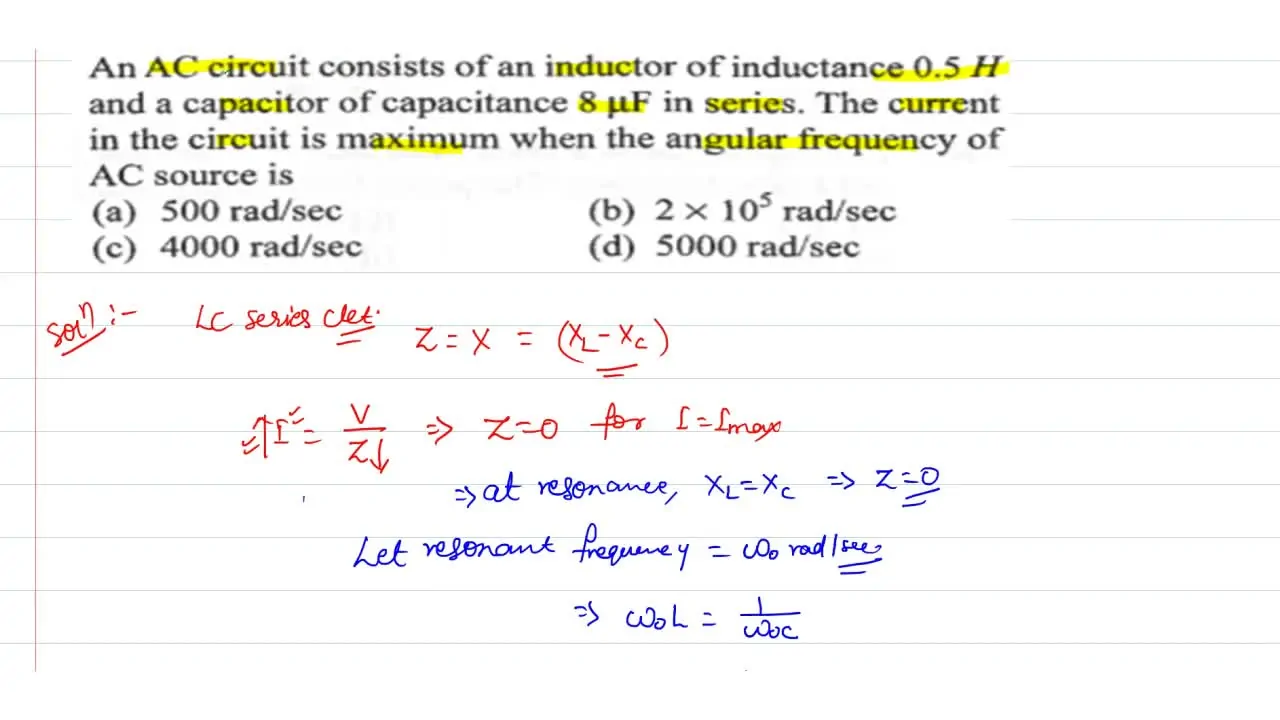
An $AC$ circuit consists of an inductor of inductance $0.5H$ and a capacitor of capacitance $8\mu F$ in series. The current in the circuit is maximum when the angular frequency of $AC$ source is

Options

A. `500 rad//sec`
B. `2xx10^(5) rad//sec`
C. `4000 rad//sec`
D. `5000 rad//sec`

Answer

A

Explanation

To find the angular frequency at which the current in an AC circuit consisting of an inductor and a capacitor in series is maximum, we can follow these steps:

Step 1: Identify the Components
We have:
- Inductance, \( L = 0.5 \, \text{H} \)
- Capacitance, \( C = 8 \, \mu\text{F} = 8 \times 10^{-6} \, \text{F} \)

Step 2: Understand Resonance Condition
In an LC circuit, the current is maximum when the circuit is at resonance. At resonance, the inductive reactance (\( X_L \)) equals the capacitive reactance (\( X_C \)):
\( X_L = X_C \)

Step 3: Write the Reactance Formulas
The reactance for the inductor is given by:
\( X_L = \omega L \)
The reactance for the capacitor is given by:
\( X_C = \frac{1}{\omega C} \)

Step 4: Set the Reactances Equal
At resonance:
\( \omega L = \frac{1}{\omega C} \)

Step 5: Rearrange the Equation
Multiplying both sides by \( \omega \):
\( \omega^2 = \frac{1}{LC} \)

Step 6: Substitute the Values
Now, substituting the values of \( L \) and \( C \):
\( \omega^2 = \frac{1}{0.5 \times 8 \times 10^{-6}} \)

Step 7: Calculate the Denominator
Calculating the denominator:
\( 0.5 \times 8 \times 10^{-6} = 4 \times 10^{-6} \)

Step 8: Calculate \( \omega^2 \)
Now substituting back:
\( \omega^2 = \frac{1}{4 \times 10^{-6}} = 250000 \, \text{rad}^2/\text{s}^2 \)

Step 9: Take the Square Root
Taking the square root to find \( \omega \):
\( \omega = \sqrt{250000} = 500 \, \text{rad/s} \)

Conclusion
Thus, the angular frequency at which the current is maximum in the given AC circuit is:
\( \omega = 500 \, \text{rad/s} \)