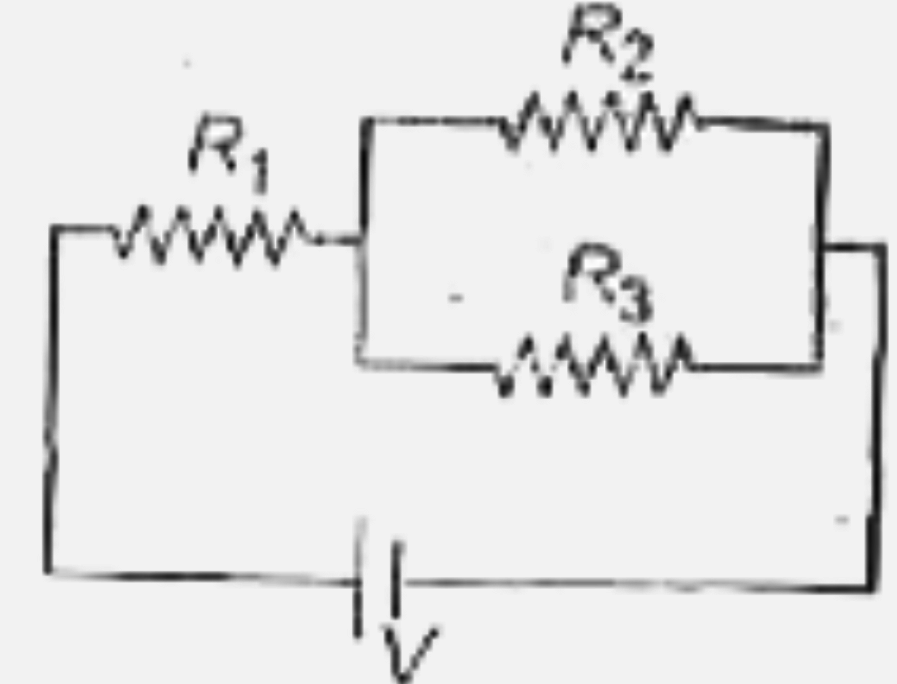
For ensuring dissipation of same energy in all three resistors (R1,R2,R3) connected as shown in figure, their values must be related s
R1=R2=R3
R2=R3 and R1=4R2
R2=R3 and R1=14R2
R1=R2+R3
The correct Answer is:C
(c ) As the voltage in R2 and R3 is same therefore, according to
H=V2R.t,R2=R3
Also the energy in all resistance is same.
∴i2R1t=i21R2t
using i1=R3R2+R3i=R3R3+R3i=12i
Thus i2R1t=i24R2r to R1=R24
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - A2ZCHAPTER - CURRENT ELECTRICITYEXERCISE - Problems Based On Mixed Concepts
34 Videos
- CURRENT ELECTRICITYBOOK - A2ZCHAPTER - CURRENT ELECTRICITYEXERCISE - Section - A
1 Videos
- CURRENT ELECTRICITYBOOK - A2ZCHAPTER - CURRENT ELECTRICITYEXERCISE - Potentiometer And Meter Bridge
45 Videos
- ATOMIC PHYSICSBOOK - A2ZCHAPTER - ATOMIC PHYSICSEXERCISE - Section D - Chapter End Test
30 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - A2ZCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - Section D - Chapter End Test
30 Videos
Similar Questions
Knowledge Check
For ensuring disspation of same energy in all three resistors (R1,R2,R3) connected as shown in figure, their values must be related as –
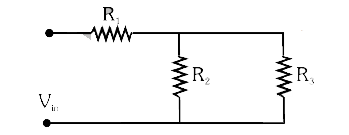 AR1=R2=R3BR2=R3andR1=4R2CR2=R3andR1=14R2DR1=R2+R3
AR1=R2=R3BR2=R3andR1=4R2CR2=R3andR1=14R2DR1=R2+R3Two ideal batteries of emf V1andV2 and three resistances R1,R2andR3 are connected as shown in the figure. The current in resistance R2 would be zero if
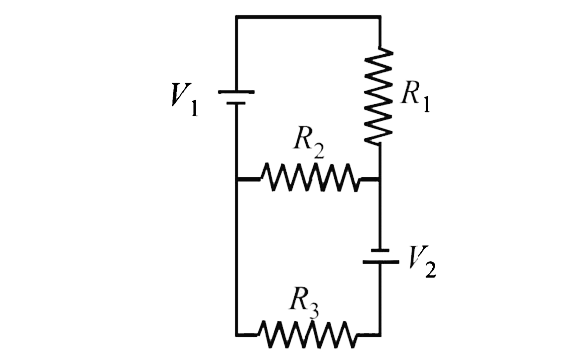 AV1=V2andR1=R2=R3BV1=V2andR1=2R2=R3CV1=2V2and2R1=2R2=R3D2V1=V2and3R1=R2=R3
AV1=V2andR1=R2=R3BV1=V2andR1=2R2=R3CV1=2V2and2R1=2R2=R3D2V1=V2and3R1=R2=R3Three equal resistors, each equals to r are connected as shown in figure. Then, the equivalent resistance between points A and B is
ArB3rCr3D2r3
Two ideal batteries of emf V1andV2 and these resistances R1,R2andR3 are connected as shown in the figure . The current in resistance R2 would be zero if
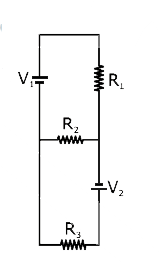
View SolutionIf resistors of resistance R1 and R2 are connected in parallel,then resultant resistance is "
View SolutionA resistor R is connected to a cell as shown in the figure. The value of R for which in its is maximum is:-
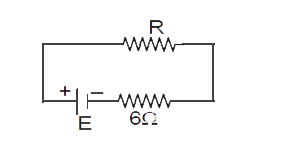
View SolutionFind the currents going through the three resistors R_(v),R_(2)andR_(3) in the circuit of figure.

View SolutionAn uncharged capacitor C and a variable resistance R are connected to an ideal source and two resistors with the help of a switch at t=0 as shown in the figure. Initially capacitor is uncharged and switch S is closed at t=0sec. The graph between VA−VB for variable Resistor R for its three different values R1, R2, and R3 versus time is shown in the figure II. Choose the correct statement
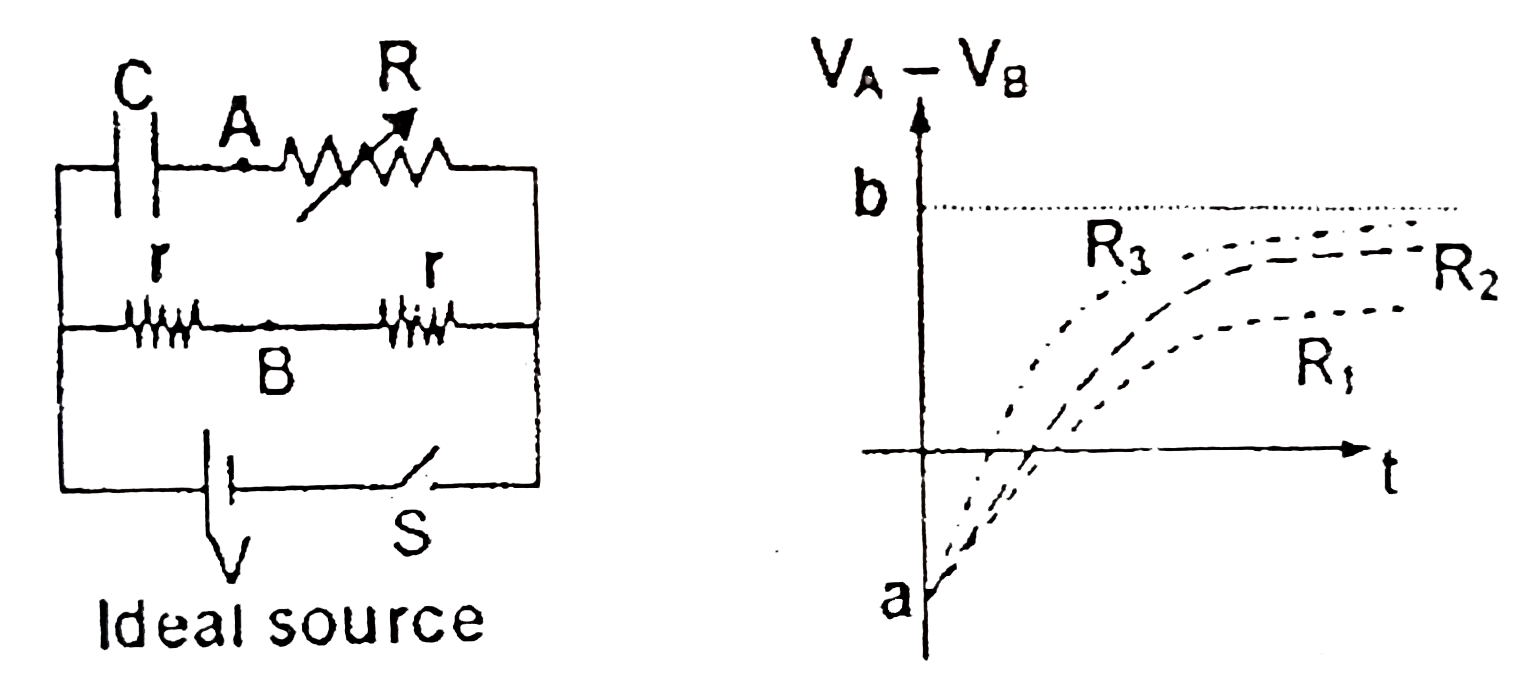
View Solution