Equal charges q are placed at the four corners A,B,C,Dof a square of length a. The magnitude of the force on the charge at B will be
3q24πε0a2
4q24πε0a2
(1+2√22)q24πε0a2
(2+1√2)q24πε0a2
The correct Answer is:c
To find the magnitude of the force on the charge at corner B of a square with equal charges placed at each corner, we can follow these steps:
Step 1: Identify the charges and their positions
We have four equal charges q placed at the corners of a square ABCD, where the length of each side of the square is a. The charges are located at:
- A (top left)
- B (top right)
- C (bottom right)
- D (bottom left)
Step 2: Determine the forces acting on charge B
The charge at B will experience repulsive forces due to the charges at A, C, and D. We need to calculate the magnitude of these forces.
Step 3: Calculate the force due to charge A on charge B
The distance between charges A and B is a.
Using Coulomb's law, the force FAB exerted by charge A on charge B is given by:
FAB=kq2a2
where k is Coulomb's constant.
Step 4: Calculate the force due to charge C on charge B
The distance between charges B and C is also a.
The force FBC exerted by charge C on charge B is:
FBC=kq2a2
Step 5: Calculate the force due to charge D on charge B
The distance between charges B and D is a.
The force FBD exerted by charge D on charge B is:
FBD=kq2a2
Step 6: Determine the directions of the forces
- The force FAB acts to the left (towards A).
- The force FBC acts to the right (towards C).
- The force FBD acts downward (towards D).
Step 7: Resolve the forces into components
Since FAB and FBD are perpendicular, we can use the Pythagorean theorem to find the resultant force Fnet acting on charge B due to charges A and D.
Step 8: Calculate the resultant force from A and D
The resultant force FAD can be calculated as:
FAD=√F2AB+F2BD=√(kq2a2)2+(kq2a2)2=√2⋅kq2a2
Step 9: Combine the forces
Now, we need to consider the force FBC acting horizontally. The total force Fnet on charge B is:
Fnet=FBC+FAD=kq2a2+√2⋅kq2a2
Fnet=kq2a2(1+√2)
Step 10: Final expression
Thus, the magnitude of the net force acting on charge B is:
Fnet=kq2a2(1+√2)
We have four equal charges q placed at the corners of a square ABCD, where the length of each side of the square is a. The charges are located at:
- A (top left)
- B (top right)
- C (bottom right)
- D (bottom left)
The charge at B will experience repulsive forces due to the charges at A, C, and D. We need to calculate the magnitude of these forces.
The distance between charges A and B is a.
Using Coulomb's law, the force FAB exerted by charge A on charge B is given by:
FAB=kq2a2
where k is Coulomb's constant.
The distance between charges B and C is also a.
The force FBC exerted by charge C on charge B is:
FBC=kq2a2
The distance between charges B and D is a.
The force FBD exerted by charge D on charge B is:
FBD=kq2a2
- The force FAB acts to the left (towards A).
- The force FBC acts to the right (towards C).
- The force FBD acts downward (towards D).
Since FAB and FBD are perpendicular, we can use the Pythagorean theorem to find the resultant force Fnet acting on charge B due to charges A and D.
The resultant force FAD can be calculated as:
FAD=√F2AB+F2BD=√(kq2a2)2+(kq2a2)2=√2⋅kq2a2
Now, we need to consider the force FBC acting horizontally. The total force Fnet on charge B is:
Fnet=FBC+FAD=kq2a2+√2⋅kq2a2
Fnet=kq2a2(1+√2)
Thus, the magnitude of the net force acting on charge B is:
Fnet=kq2a2(1+√2)
Topper's Solved these Questions
- ELECTRIC CHARGE, FIELD & FLUXBook:A2ZChapter:ELECTRIC CHARGE, FIELD & FLUXExercise:Electric Field Due To Point Charge
Explore 25 Videos
- ELECTRIC CHARGE, FIELD & FLUXBook:A2ZChapter:ELECTRIC CHARGE, FIELD & FLUXExercise:Electric Field And Motion
Explore 37 Videos
- DUAL NATURE OF RADIATION AND MATTERBook:A2ZChapter:DUAL NATURE OF RADIATION AND MATTERExercise:Section D - Chapter End Test
Explore 30 Videos
- ELECTRIC POTENTIAL & CAPACITANCEBook:A2ZChapter:ELECTRIC POTENTIAL & CAPACITANCEExercise:Section D - Chapter End Test
Explore 29 Videos
Similar Questions
Four identical point charges q are placed at four corners of a square of side a. Find the potential energy of the charge system.
 .
.
View SolutionConsider four equal charges (q, each) placed on the corners of a square with side a. Determine the magnitude and direction of the resultant force on the charge on lower right corner.
View Solution
Knowledge Check
Equal charges q are placed at the three corners B, C, D of a square ABCD of side a. The potential at A is
A14πε0qaB3q4πε0Cq4πε0a[2+1√2]Dq4πε0a[1+√2]Equal charges q are placed at the three corners B, C, D of a square ABCD of side 'a'. The ratio of potential at A to centre O is
A2√2+13√2B2+√26C2√2+16D2√2+13q,2q, 3q and 4q charges are placed at the four corners A,B,C and D of a square. The field at the centre P of the square has the direction parallel to
 AABBCBCACDBD
AABBCBCACDBD
Four identical charges q are placed at the corners of a square of side a. Find the potential energy of one of the charges due to the remaining charges.
 .
.
View SolutionFour equal charges Q are placed at the four corners of a square of each side is 'a'. Work done in removing a charge −Q from its centre to infinity is
View SolutionEqual charges q are placed at the vertices A and B of an equilatral triangle ABC of side a. The magnitude of electric field at the point C is
View SolutionFour charges are arranged at the corners of a square ABCD as shown in the figure. The force on the charge kept at the centre O will be:
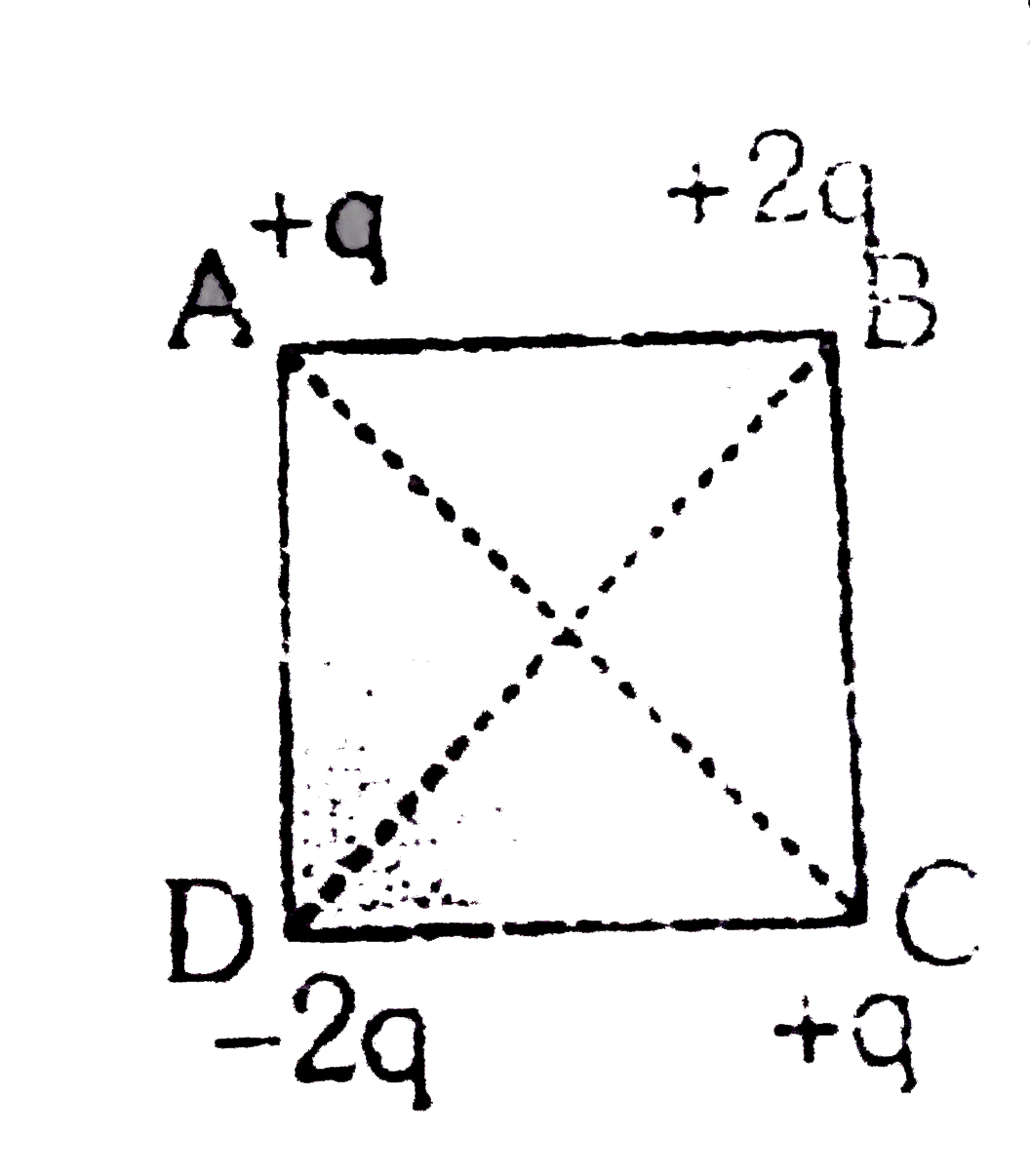
View SolutionThree point charges each of value +q are placed on three vertices of a square of side a metre. What is the magnitude of the force on a point charge of value −q coulomb placed at the centre of the square?
View Solution