An object is kept at 60 cm in front of a convex lens. Its real image is formed at 20 cm from the lens . Find the focal length and power of the lens.
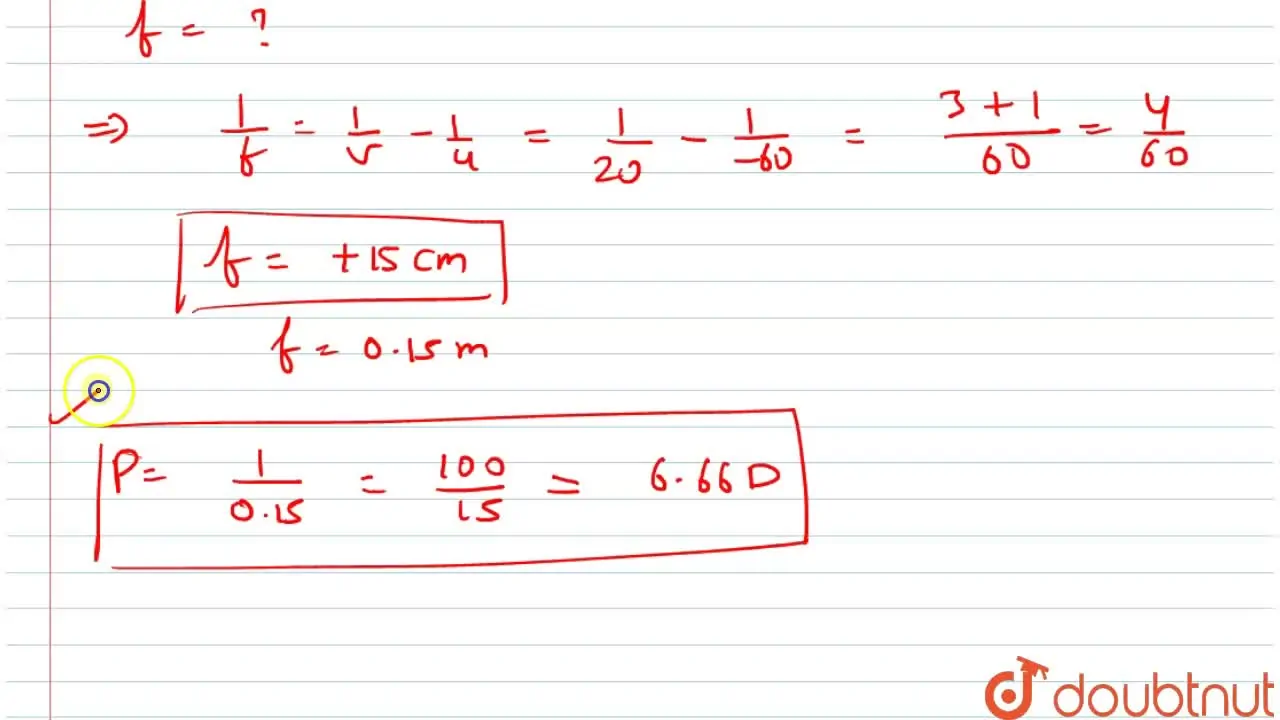
Data : Convex lens , u=−60 cm,
v=20 cm, f= ?
1f=1v−1u=120cm−1−60cm=120cm+160cm
=3+160cm+460cm=115cm
∴f=15cm
The focal length of the lens =15 cm.
Related Playlists
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - LENSESEXERCISE - Numerical Problems For Practice20videos
LENSES
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - LENSESEXERCISE - Paragraph5videos
LENSES
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - LAWS, RULES, UNITS AND DEFINITIONS EXERCISE - Carbon Compounds18videos
LAWS, RULES, UNITS AND DEFINITIONS
- BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - MAKING CONCEPT DIAGRAMS EXERCISE - Cell Biology and Biotechnology2videos
MAKING CONCEPT DIAGRAMS
Similar Questions
An object is kept at 20 cm in front of a convex lens and its real image is formed at 60 cm from the lens . Find The focal length and power of the lens .
View SolutionAn object is kept at 20 cm in front of a convex lens and its real image is formed at 60 cm from the lens. Find (1) the focal length of the lens (2) the image if the height of the object is 6 cm.
View SolutionAn object of height 2 cm is kept at 30 cm from a convex lens . Its real image is formed at 60 cm from the lens. Find the focal length of power of the lens.
View SolutionAn object is kept at 20 cm in front of a convex lens and its real image is formed at 60 cm from the lens . Find the height of the image if the height of the object is 6 cm.
View SolutionAn object is kept at 10 cm in front of a convex lens. Its imge is formed on the screen at 15 cm from the lens. Calculate the focal length of the lens.
View SolutionAn object is kept at 10 cm in front of a convex lens. Its image is formed on the screen at 15 cm from the lens. Calculate (1) the focal length of the lens (2) the magnification produced by the lens.
View SolutionAn object is kept at a distance of 60 cm in front of a convex lens. Its real and inverted image is fonned at a distance of 20 cm from the lens. Find the focal length of the lens.
View SolutionWhen an object is placed 40cm from a diverging lens, its virtual image is formed 20cm from the lens. The focal length and power of lens are
View SolutionAn object is kept at 10 cm in front of a convex lens. Its imge is formed on the screen at 15 cm from the lens. Calculate the magnification produced by the lens.
View SolutionAn object kept 60 cm from a lens gives a virtual image 20 cm in front of the lens . What is the focal length and power of the lens ? Is it is converging lens or diverging lens ?
View Solution