Seg RM and seg RN are tangent segments of a circle with centre O. Prove that seg OR bisects ∠MRN as well as ∠MON.
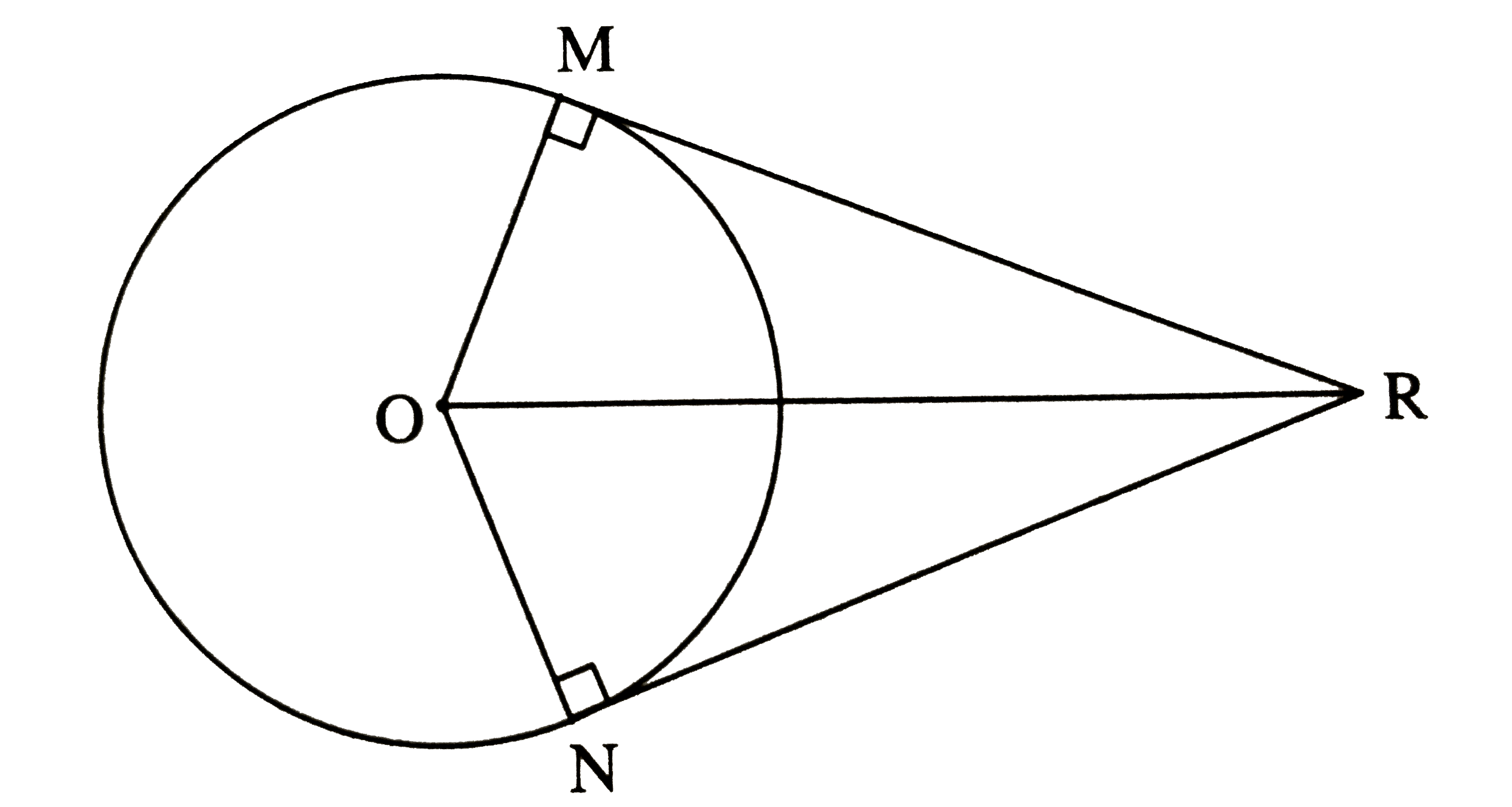
In △RMO and △RNO
∠RMO=∠RNO=90∘ ……( Tangent theorem )
Hypotenuse OR≅ hypotenuse OR …….( Common side )
Side OM≅ side ON ……( Radii of the same circle )
∴△RMO≅△RNO .......( Hypotenuse side theorem )
∴∠MRO≅∠NRO
and ∠MOR≅∠NOR
∴ seg OR bisects ∠MRN and ∠MON.
Topper's Solved these Questions
- CIRCLEBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - PRACTICE SET 3.2
8 Videos
- CIRCLEBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - PRACTICE SET 3.3
3 Videos
- CIRCLEBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CIRCLEEXERCISE - EXAMPLES FOR PRACTICE
40 Videos
- CHALLENGING QUESTIONSBOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - CHALLENGING QUESTIONSEXERCISE - SECTION 3 (MODEL QUESTION PAPER FOR PRACTICE ) Solve any one of the following subquestions :
1 Video
- COORDINATE GEOMETRY BOOK - NAVNEET PUBLICATION - MAHARASHTRA BOARDCHAPTER - COORDINATE GEOMETRY EXERCISE - Assignment 6.5
13 Videos
Similar Questions
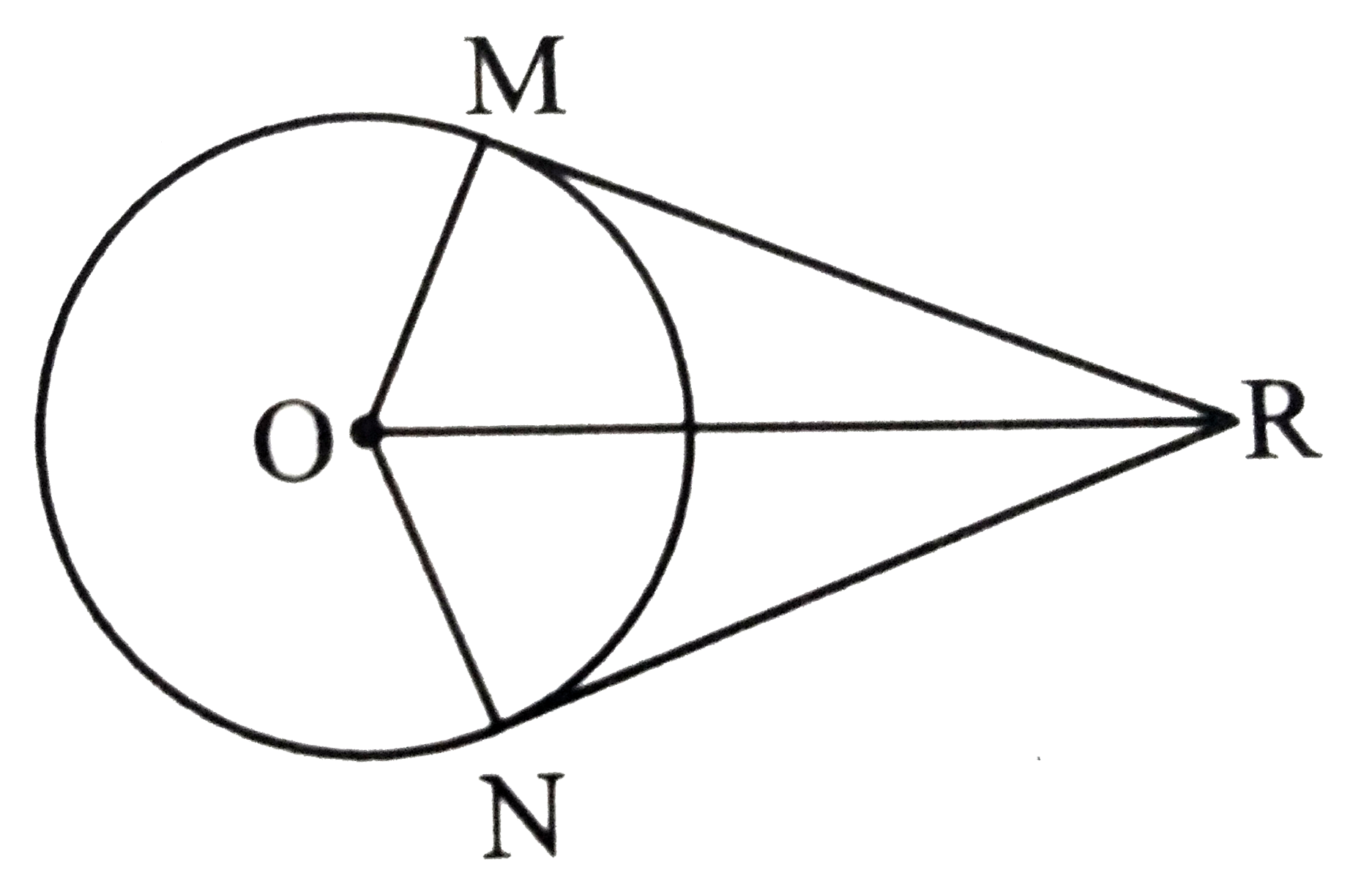
Seg RM and seg RN are tangents segments of a circle with centre O. Prove that seg OR bisects∠MRN as well as ∠MON .
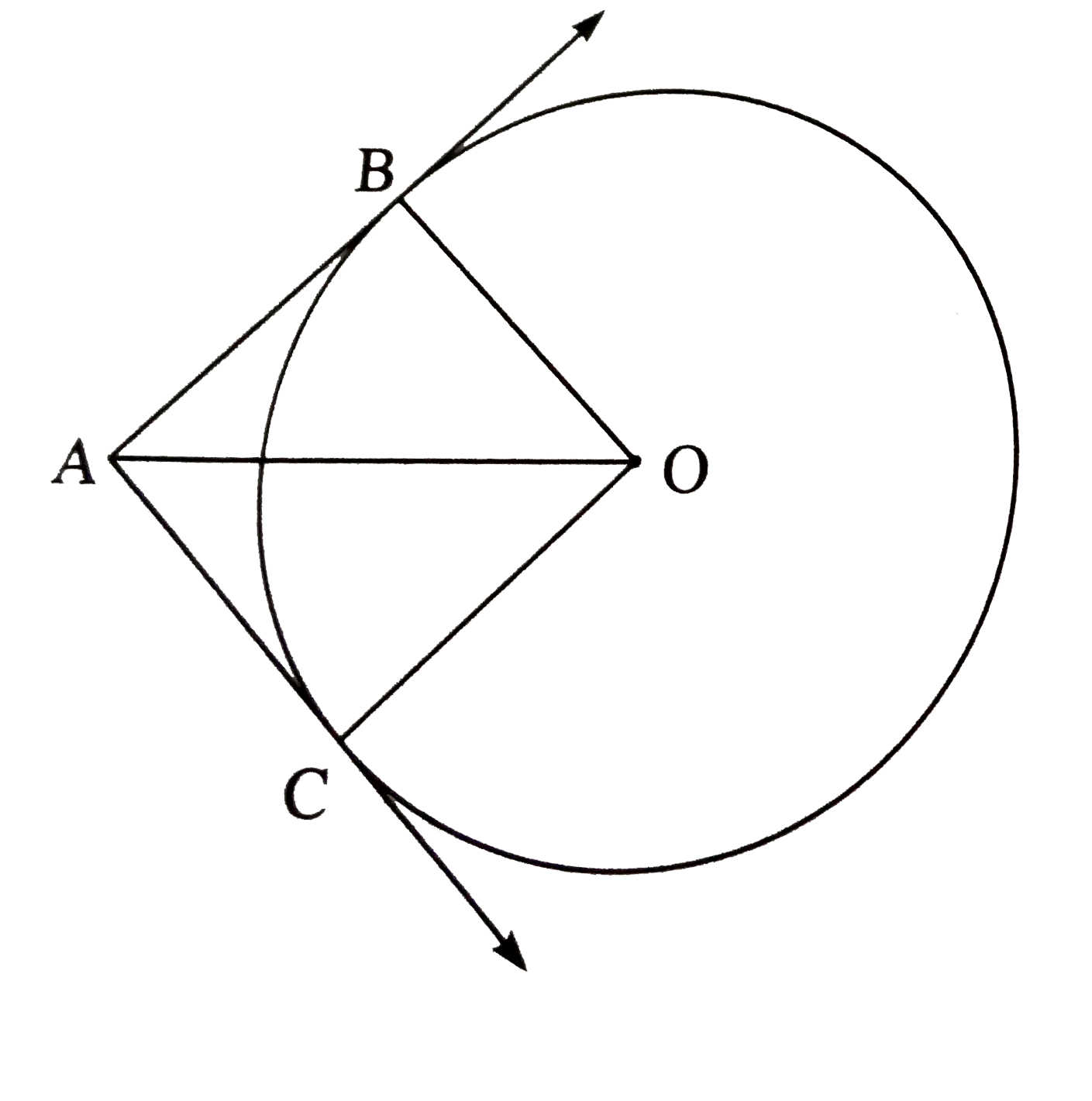
View SolutionIn the adjoining figure, O is the centre of the circle. Seg AB, seg AC are tangent segments. Radius of the circle is r and l(AB)=r. Prove that , □ ABOC is a square.
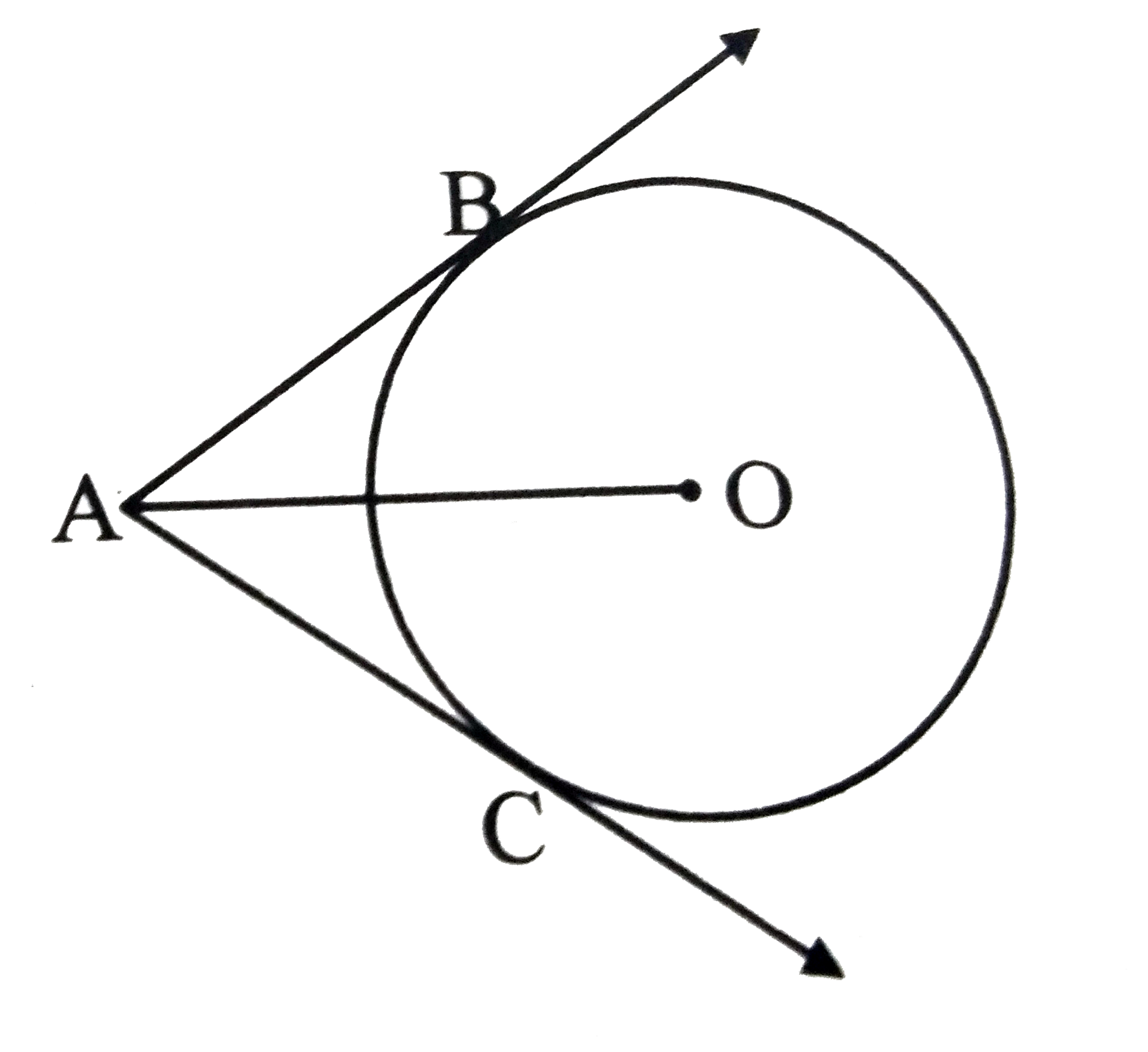
View SolutionIn Figure, BC is a tangent to the circle with centre O.OE bisects AP. Prove that AEO~ABC.
View SolutionIn the figure, O is the centre of the circle of the circle. Seg AB, seg AC are tangent segments. Radius of the circle is r and l(AB)=r. Prove that, □ABOC is a square.
View SolutionIn Figure, AB=CB and O is the centre of the circle. Prove that BO bisects ∠ABC.
View SolutionIn the figure , O is the radius of the circle. From point R, seg RM and seg RN are tangent segments touching the circle at M and N. If OR = 10cm and radius of the circle = 5 cm, then (i) What is the length of each tangent segment ? (iii) What is the measure of ∠MRO ? (iii) What is the measure of ∠MRN ?
View SolutionIn Fig. 10.64, BC is a tangent to the circle with centre O.OE bisects AP. Prove that ΔABC~ΔAEO
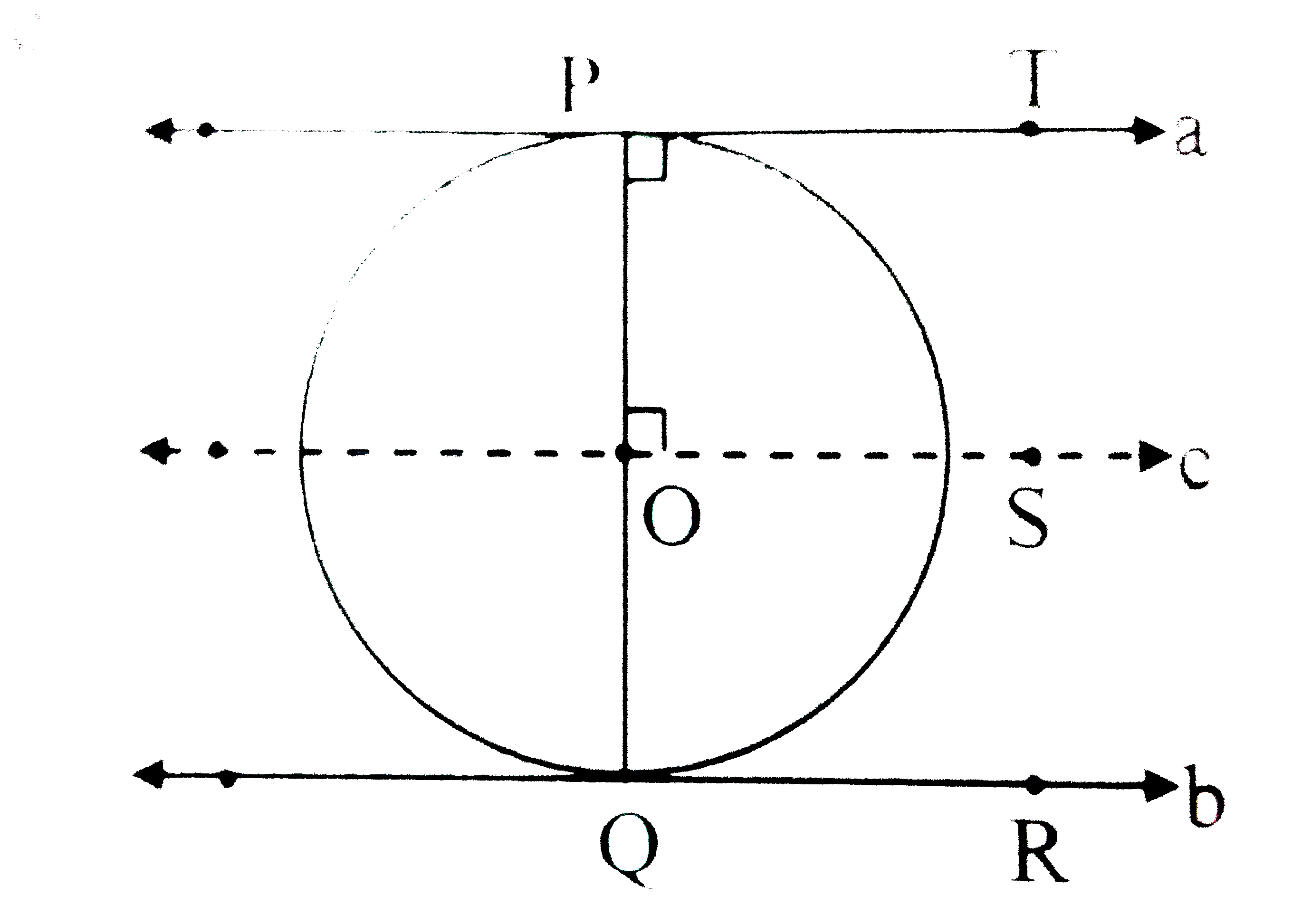
View SolutionPoint O is the centre of a circle . Line a and line b are parallel tangents to the circle at P and Q. Prove that segment PQ is a diameter of the circle.
View SolutionTwo tangent segments PA and PB are drawn to a circle with centre O such that ∠APB=120o . Prove that OP=2 AP .
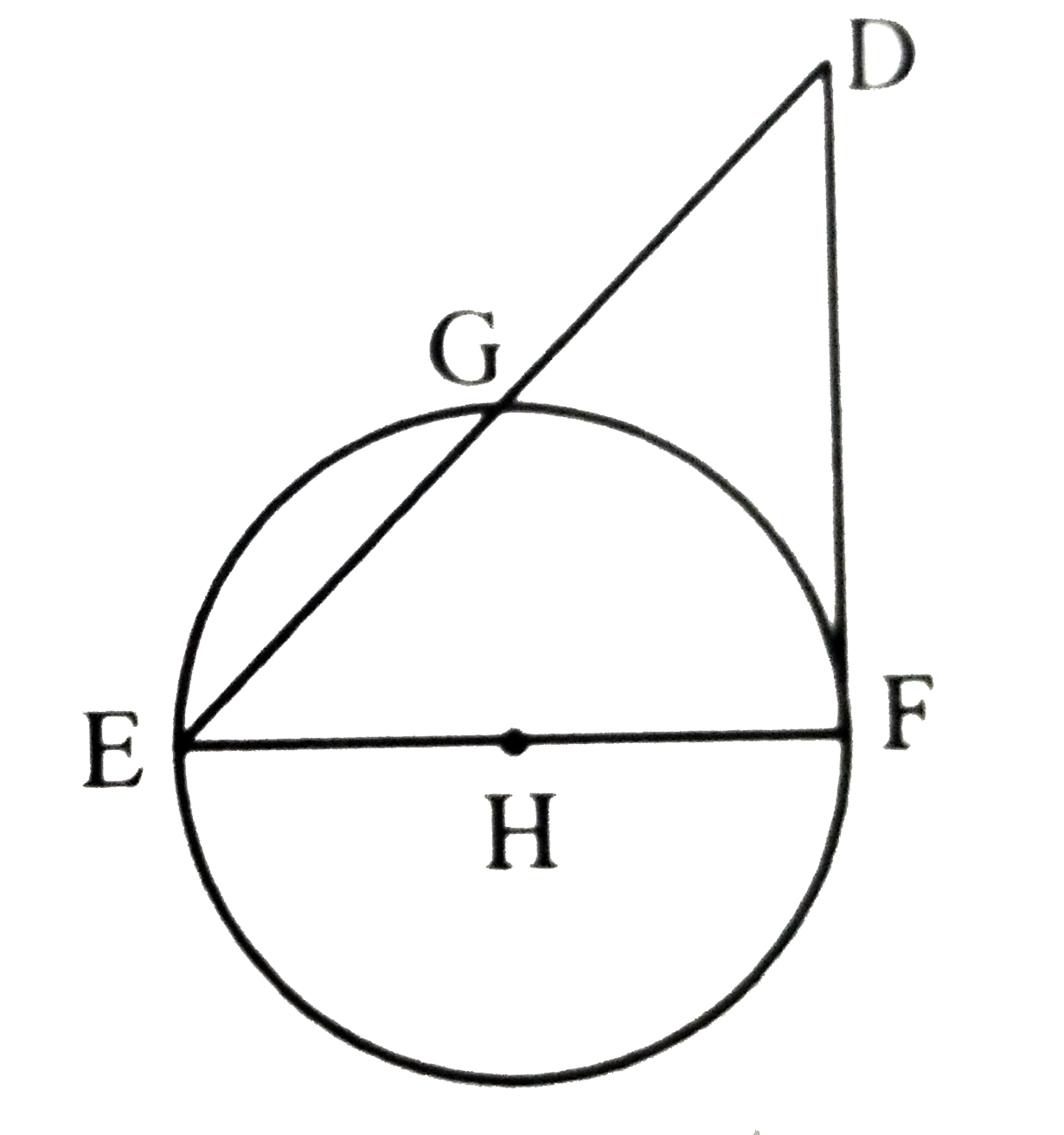
View SolutionIn the adjoining figure , seg EF is a diameter and seg DF is a tangent segment. The radius of the circle is r. Prove that, DE×GE=4r2.
View Solution