ज्ञात करें ∫ cosecnxcotxdx(n≠0)
Video Solution
Text Solution
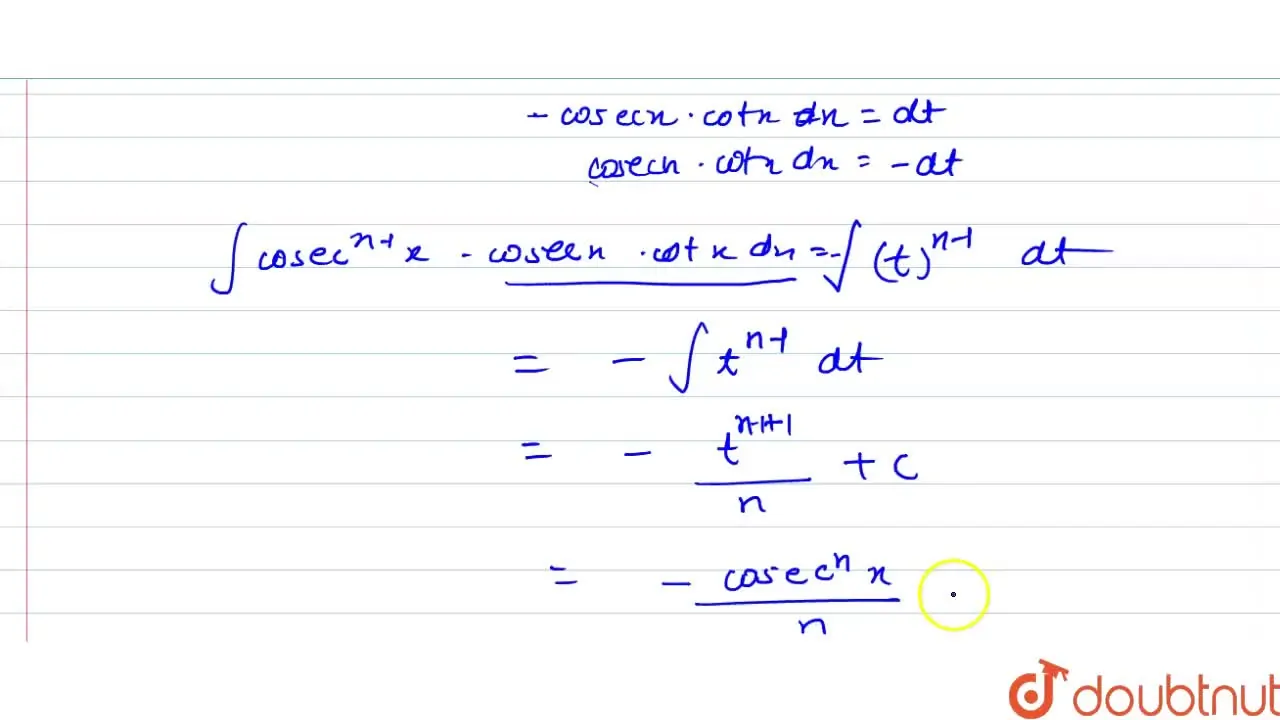
यहां cot x का घात धन विषम पूर्णांक है इसलिए z=cosecx रखें
माना की z=cosecnxcotxdx=∫cosecn−1xcosecxcotxdx
=∫zn−1(−dz)=−∫z?n−1dz
=−z2n+c=−cosecnxn+c.
|
Updated on:21/07/2023
Related Playlists
- BOOK - KC SINHACHAPTER - अनिश्चित समाकल EXERCISE - अभ्यास 19.149videos
अनिश्चित समाकल
- BOOK - KC SINHACHAPTER - अनिश्चित समाकल EXERCISE - अभ्यास 19.262videos
अनिश्चित समाकल
- BOOK - KC SINHACHAPTER - QUESTIONS OF J.A.C. 2014EXERCISE - SECTION-D10videos
QUESTIONS OF J.A.C. 2014
- BOOK - KC SINHACHAPTER - अवकल समीकरण EXERCISE - अभ्यास 23.745videos
अवकल समीकरण
Similar Questions
∫Cos2x
03:15View Solution∫dx√x+a−√x+2
01:40View Solutionसिद्ध करें कि 9π8−94sin−113=94sin−12√23
01:02View Solutionवैसे अवकल समीकरणों के हल पर आधारित प्रशन जिनको उपयुक्त प्रतिस्थापन द्वारा dy/dx+Py=Q , के रूप में लाया जा जहाँ P और Q केवल x के फलन या अचर हों: अवकल समीकरणों को हल करें| xdydx−3y=x2
01:19View SolutionEvaluate: ∫cosecxlog(cosecx−cotx)dx
04:08View SolutionEvaluate: ∫cotnxcosec2xdx,n≠−1
02:38View Solutionint(1)/(csc x-cot x)dx=
02:38View Solution∫cosecx(cosecx+cotx)dx=?
03:32View Solution∫cotx(cosecx−cotx)dx=?
07:36View Solution∫secnxtanxdx,n≠0
01:50View Solution∫cotnncosec2xdx,n≠−1
03:22View Solution∫π/20(n√secxn√secx+n√cosecx)dx=
01:37View SolutionWe can derive different reduction formulas by using integration by parts.
If ∫cosecnxdx=−cosecn−2xcotxn−1+A∫cosecn−2xdx, then A is equal to-02:27View Solutionसमाकलन करें
∫cosec x(cosec x - cot x)dx03:38View Solutionसमाकलन करें
∫ cosec x cosec x + cot xdx02:36View Solution