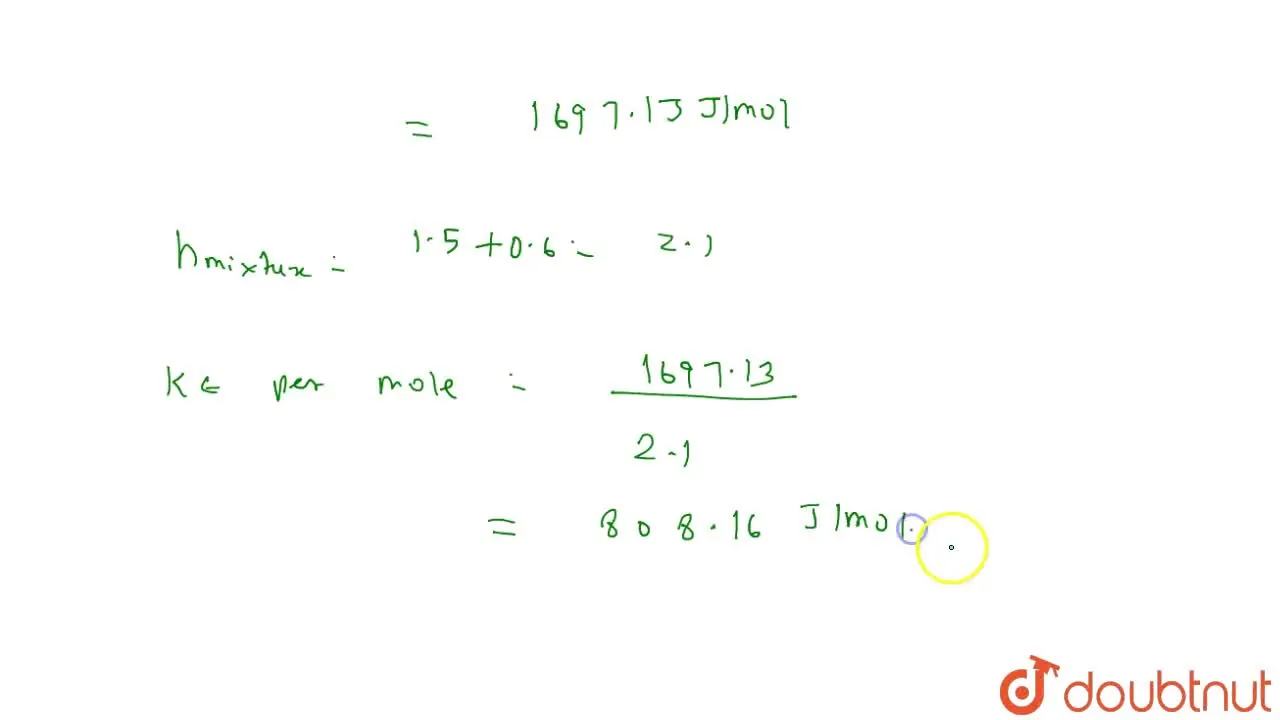6.0g he and 12.0g Ne molecules both having average velocity 4×102ms−1 are mixed Calculate kinetic energy per mo1 of the mixture .
4×102=√8RTπ×4×10−3(Minkg)
∴RT=16×104×π×4×10−38=80π
For Ne
4×102=4×102=√8RTπ×20×10−3(Minkg) ltbr gt∴RT=16×104×π×20×10−38=400π
∵KE=32nRT
∴ Total KE of He=32×64×80π=565.71J/mo1
∴ Total KE of Ne=32×1220×400π=1131.42J/mo1
=1697.13J/mo1
∴ Total KE of mixture =64+1220=1.5+0.6=21
KE / mole of mixture =1697.132.1=808.16 J/mo1 .
Related Playlists
- BOOK - P BAHADURCHAPTER - GASEOUS STATEEXERCISE - Exercise B1videos
GASEOUS STATE
- BOOK - P BAHADURCHAPTER - GASEOUS STATEEXERCISE - Exercise 41videos
GASEOUS STATE
- BOOK - P BAHADURCHAPTER - GASEOUS STATEEXERCISE - Exercise 31videos
GASEOUS STATE
- BOOK - P BAHADURCHAPTER - CHEMICAL EQUILIBRIUMEXERCISE - Exercise134videos
CHEMICAL EQUILIBRIUM
- BOOK - P BAHADURCHAPTER - IONIC EQUILIBRIUMEXERCISE - Exercise85videos
IONIC EQUILIBRIUM
Similar Questions
2 moles of Ne gas and 5 moles of He gas, both samples having average velocity 7×102m/s, are mixed.Find the average translational kinetic energy per mole of the given gas mixture (in joules).Report your answer as 'X' where X=(Average translational KE in joules)×0.002.The reported answer should be upto nearest integer.
View SolutionTwo vessels A and B are identical. A has 1 g hydrogen at 0∘C and B has 1 g oxygen at 0∘C. Vessel A contains x molecules and B contains y molecules. The average kinetic energy per molecules in A is 'n' times the average kinetic energy per molecule in B. The value of 'n' is
View SolutionGiven, Avogadro's number N=6.02×1023 and Boltzmann's constant k=1.38×10−23J/K.
(a) Calculate the average kinetic energy of translation of the molecules of an ideal gas at 0∘Candat100∘C.
(b) Also calculate the corresponding energies per mole of the gas.View SolutionGiven, Avogadro's number N=6.02×1023 and Boltzmann's constant k=1.38×10−23J/K.
(a) Calculate the average kinetic energy of translation of the molecules of an ideal gas at 0∘Candat100∘C.
(b) Also calculate the corresponding energies per mole of the gas.View SolutionThe root mean square speed of 8 g of He is 300 ms−1. Total kinetic energy of He gas is :
View SolutionA body of mass 0.8 kg has intial velocity (3ˆi−4ˆj)ms−1 and final velocity (−6ˆj+2ˆk)ms−1. Find change in kinetic energy of the body?
View SolutionA gas consists of 4 molecules with a velocity of 5ms−1,10 molecules with a velocity of 3ms−1 and 6 molecules with a velocity of 6ms−1. Calculate their average velocity, root mean square velocity and most probable velocity.
View SolutionA particle of mass 0.6g and having charge of 25nC is moving horizontally with a uniform velocity 1.2×104ms−1 in a uniform magnetic field, then the value of the magnetic induction is (g=10ms−2)
View SolutionA beam of helium atoms moves with a velocity of2×104ms−1. Find the wavelength of paritcles constituting with the beam .
Hint : Mass of helium atom =46.022×1023gView SolutionThe energy absorbed by each molecule (A2) of a substance is 4.4×10−19 J and bond energy per molecule is 4.0 x 10-19 J. 4.0×10−19 J . The kinetic energy of the molecule per atom will be
View Solution