A variable triangle ABC is circumscribed about a fixed circle of unit radius. Side BC always touches the circle at D and has fixed direction. If B and C vary in such a way that (BD).(CD)=2, then locus of vertex A will be a straight line
parallel to side BC
right angle to side BC
making and angle π6 with BC
making an angle sin−1(23) with BC
The correct Answer is:A
Step by step video, text & image solution for A variable triangle ABC is circumscribed about a fixed circle of unit radius. Side BC always touches the circle at D and has fixed direction. If B and C vary in such a way that (BD). (CD)=2, then locus of vertex A will be a straight line by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHSCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise (More Than One Correct Option Type Questions)
24 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHSCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise (Statement I And Ii Type Questions)
15 Videos
- PROPERTIES AND SOLUTION OF TRIANGLES BOOK - ARIHANT MATHSCHAPTER - PROPERTIES AND SOLUTION OF TRIANGLES EXERCISE - Exercise For Sesssion 8
5 Videos
- PRODUCT OF VECTORSBOOK - ARIHANT MATHSCHAPTER - PRODUCT OF VECTORSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
52 Videos
- SEQUENCES AND SERIESBOOK - ARIHANT MATHSCHAPTER - SEQUENCES AND SERIESEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
38 Videos
Similar Questions
A variable triangle ABC is circumscribed about a fixed circle of unit radius. Side BC always touches the circle at D and has fixed direction. If B and C vary in such a way that (BD) (CD)=2, then locus of vertex A will be a straight line. parallel to side BC perpendicular to side BC making an angle (π6) with BC making an angle sin−1(23) with BC
View SolutionIn ΔABC, vertex B and C are fixed and vertex A is variable such that 2sin(B−C2)=cos(A2) then locus of vertex A is
View Solution
Knowledge Check
ABC is isosceles triangle where AB = AC which is circumscribed about a circel. If P is the point where the circle touches the side BC, then which of the following is true?
ABP=PCBBP>PCCBP<PCDBP=12PCA and B are two fixed points. The vertex C of triangle ABC moves such that cotA+cotB= constant. The locus of C is a straight line
Aperpendicular to ABBparallel to ABCinclined at an angle (A−B) to ABDNone of theseA and B are two fixed points. The vertex C of a ΔBC moves such that cotA+cotB= constant. Locus of C is a straight line
A⊥ to ABBparallel to ABCInclined at an angle of A−B to ABDnone
ABC is isosceles triangle where AB = AC which is circumscribed about a circel. If P is the point where the circle touches the side BC, then which of the following is true?
View SolutionBase BC of triangle ABC is fixed and opposite vertex A moves in such a way that tan.B2tan.C2 is constant. Prove that locus of vertex A is ellipse.
View SolutionVertex A of a variable triangle ABC, inscribed in a circle of radius R, is a fixed point. If the angles subtended by the side BC at orthocentre (H), circumcentre (O) and incentre (I) are equal than identify the locus of orthocentre of triangle ABC.
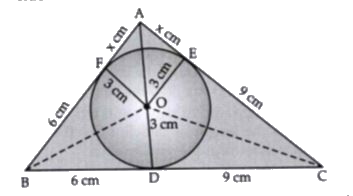
View SolutionIn figure , a triangle ABC is drawn to circumscribe a circle of radius 3 cm , such that segments BD and DC are of length 6 cm and 9 cm .If the area of △ABC=54cm2, then find the lengths of the side AB and AC.
View SolutionThe in circle of ΔABC touches the sides BC, CA and AB at D, E and F respectively. Then AF + BD + CE is equal to :
View Solution