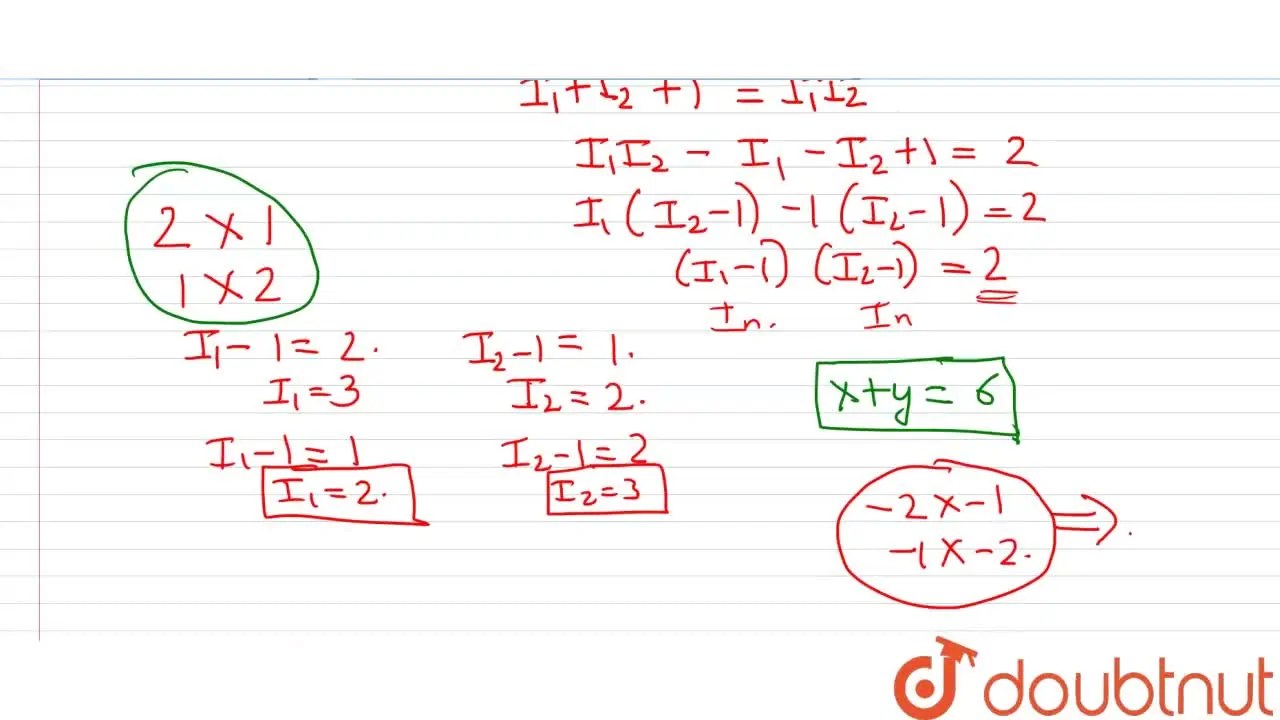
Consider the equation x+y-[x][y]=0, where [*] is the greatest integer

The solution set of the equation ${[x]}^{2} + [x + 1] - 3 = 0,$ where $[.]$ represents greatest integeral function is :
A $[- 1, o) \cup [1, 2)$
B $[- 2, - 1) \cup [1, 2]$
C $[1, 2]$
D $[- 3, - 2) \cup [2, 3)$
Area bounded by the relation [2x]+[y]=5, x,y $>$ 0 is (where $[\cdot]$ represent greatest integer function)
A2
B3
C4
D5
Number of solutions of the equation $x^{2} - 2 = [sin x]$ , where [.] denotes the greatest integer function, is
A3
B4
C2
D1

Consider the equation x+y-[x][y]=0, where $[\cdot]$ is the greatest integer function.
Equation of one of the lines on which the non-integral solution of given equation lies, is