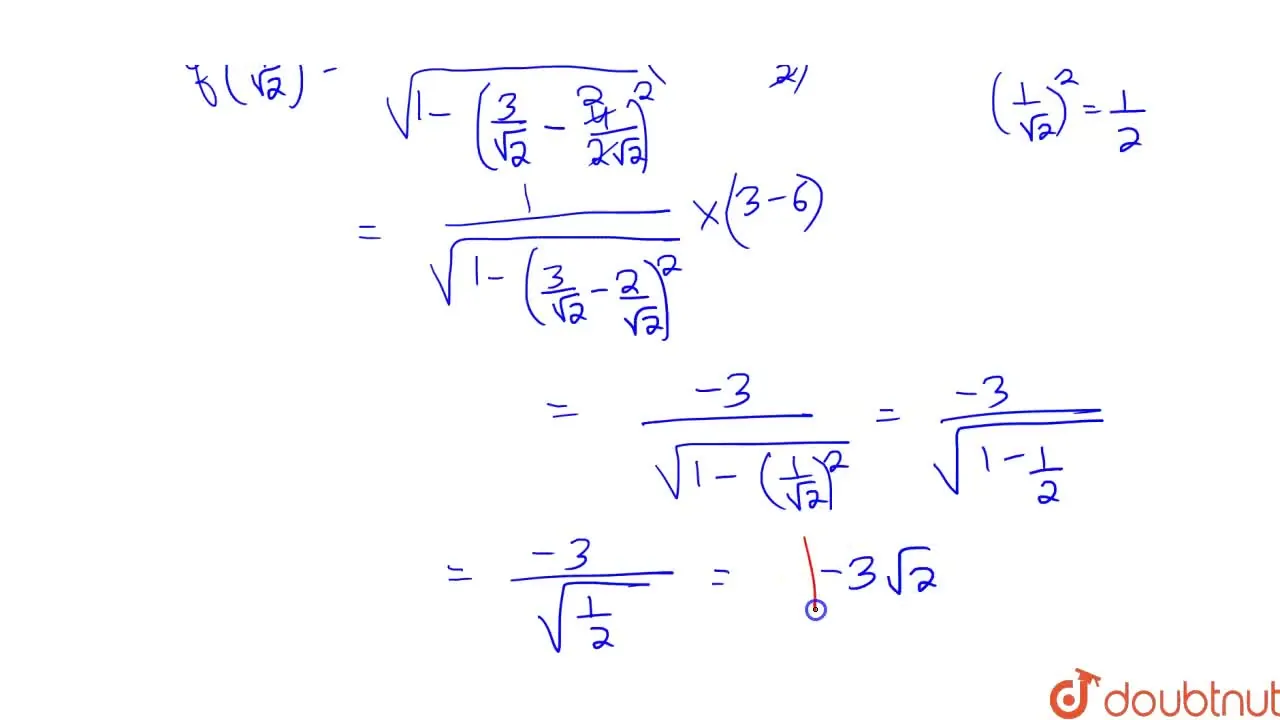If f(x)=sin−1(3x−4x3). Then answer the following
The value of f'(1√2) , is
-3
3
−3√2
3√2
The correct Answer is:C
Step by step video, text & image solution for If f(x)=sin^(-1)(3x-4x^(3)). Then answer the following The value of f'((1)/(sqrt2)) , is by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Related Playlists
- BOOK - ARIHANT MATHSCHAPTER - DIFFERENTIATION EXERCISE - Exercise (Subjective Type Questions)15videos
DIFFERENTIATION
- BOOK - ARIHANT MATHSCHAPTER - DIFFERENTIATION EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)8videos
DIFFERENTIATION
- BOOK - ARIHANT MATHSCHAPTER - DIFFERENTIATION EXERCISE - Exercise (Statement I And Ii Type Questions)10videos
DIFFERENTIATION
- BOOK - ARIHANT MATHSCHAPTER - DIFFERENTIAL EQUATION EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)26videos
DIFFERENTIAL EQUATION
- BOOK - ARIHANT MATHSCHAPTER - DY / DX AS A RATE MEASURER AND TANGENTS, NORMALS EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)7videos
DY / DX AS A RATE MEASURER AND TANGENTS, NORMALS
Similar Questions
If f(x)=sin−1(3x−4x3). Then answer the following
The value of f'(0), isView SolutionIf f'(x)=1x+x2 and f(1)=43 then find the value of f(x)
View SolutionIf f(x)=(x−1)4(x−2)3(x−3)2 then the value of f'(1)+f''(2)+f''(3) is:
View Solutionif f(x)=sin(π3[x]−x2) then the value of f(√π3) is
View Solutionf(x)=sin−1(3−2x5)+√3−x.Find the domain of f(x).
View SolutionIf f(x)=x3+1x3−4(x2+1x2)+13 then the value of f(2+√3)=
View SolutionIf f(x)=3x2+4x−1, then find the approximate value of f(3.1).
View SolutionIf f(x)=(x−1)4(x−2)3(x−3)2(x−4), then the value of f'''(1)+f''(2)+f'(3)+f'(4) equals
View SolutionSTATEMENT-1: The values of f(x)=3sin(√π216−x2 lie in the interval [0,3√3]. and STATEMENT-2 The domain of definition of the function f(x)=3sin(√π216−x2 is [−π4,π4] .
View SolutionThe domain of f(x)=√sin−1(3x−4x3)+√cos−1x is equal to
View Solution