Let f(x) = [ n + p sin x], , p a prime number and [x] = the greatest integer less than or equal to x. The number of points at which f(x) is not not differentiable is :
The correct Answer is:
Step by step video, text & image solution for Let f(x) = [ n + p sin x], x in (0,pi), n in Z, p a prime number and [x] = the greatest integer less than or equal to x. The number of points at which f(x) is not not differentiable is : by Maths experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
- CONTINUITY AND DIFFERENTIABILITYBOOK - ARIHANT MATHSCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise For Session 1
5 Videos
- CONTINUITY AND DIFFERENTIABILITYBOOK - ARIHANT MATHSCHAPTER - CONTINUITY AND DIFFERENTIABILITYEXERCISE - Exercise For Session 2
4 Videos
- COMPLEX NUMBERSBOOK - ARIHANT MATHSCHAPTER - COMPLEX NUMBERSEXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
43 Videos
- COORDINATE SYSTEM AND COORDINATES BOOK - ARIHANT MATHSCHAPTER - COORDINATE SYSTEM AND COORDINATES EXERCISE - Exercise (Questions Asked In Previous 13 Years Exam)
7 Videos
Similar Questions
Knowledge Check
Let |x| be the greatest integer less than or equal to x, Then f(x)=xcos(π(x+[x]) is continous at
Ax=−1Bx=0Cx=2Dx−1Let [x] denotes the greatest integer less than or equal to x. If f(x)=[xsinπx], then f(x) is
Acontinuous at x = 0Bcontinuous in (−1,0)Cdifferentiable at x = 1Ddifferentiable in (−1,1)Let [x] denotes the greatest integer less than or equal to x. If f(x)=[xsinπx], then f(x) is
Acontinuous at x=0,Bcontinuous in (-1,0),Cdifferentiable at x = 1Ddifferentiable in (-1,1),
If f(x)=|x−1|−[x] (where [x] is greatest integer less than or equal to x) then.
View SolutionIf f(x)=|x−1|−[x], where [x] is the greatest integer less than or equal to x, then
View SolutionLet f(x)=[cosx+sinx], 0<x<2π, where [x] denotes the greatest integer less than or equal to x. The number of points of discontinuity of f(x) is
View SolutionLet [x] denotes the greatest integer less than or equal to x. If f(x)=[xsinπx], then f(x) is
View SolutionLet [x] be the greatest integer less than or equal to x, for a real number x. Then the following sum
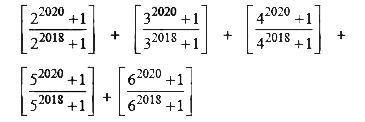
is-
View Solution
