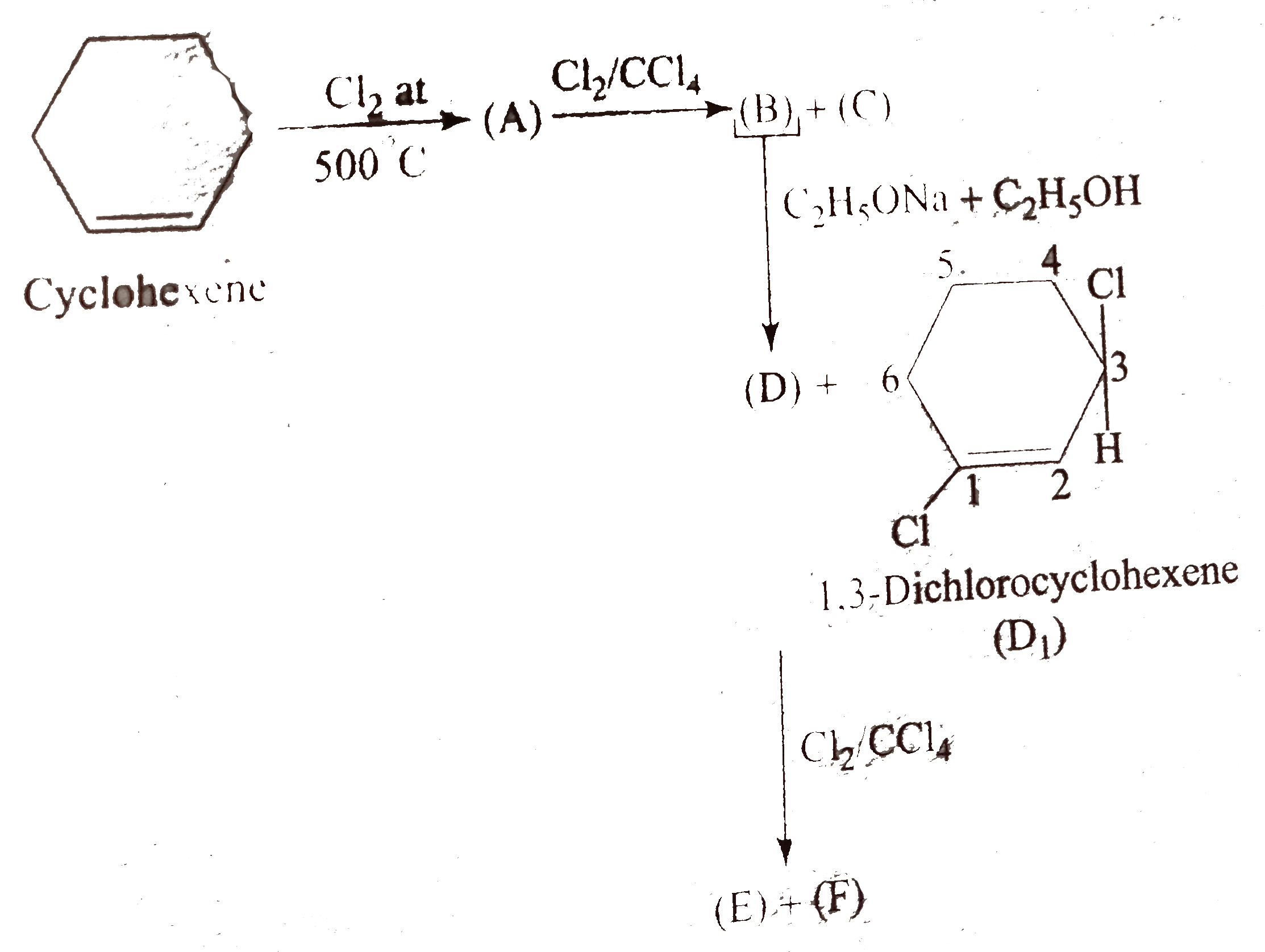
(D) is :



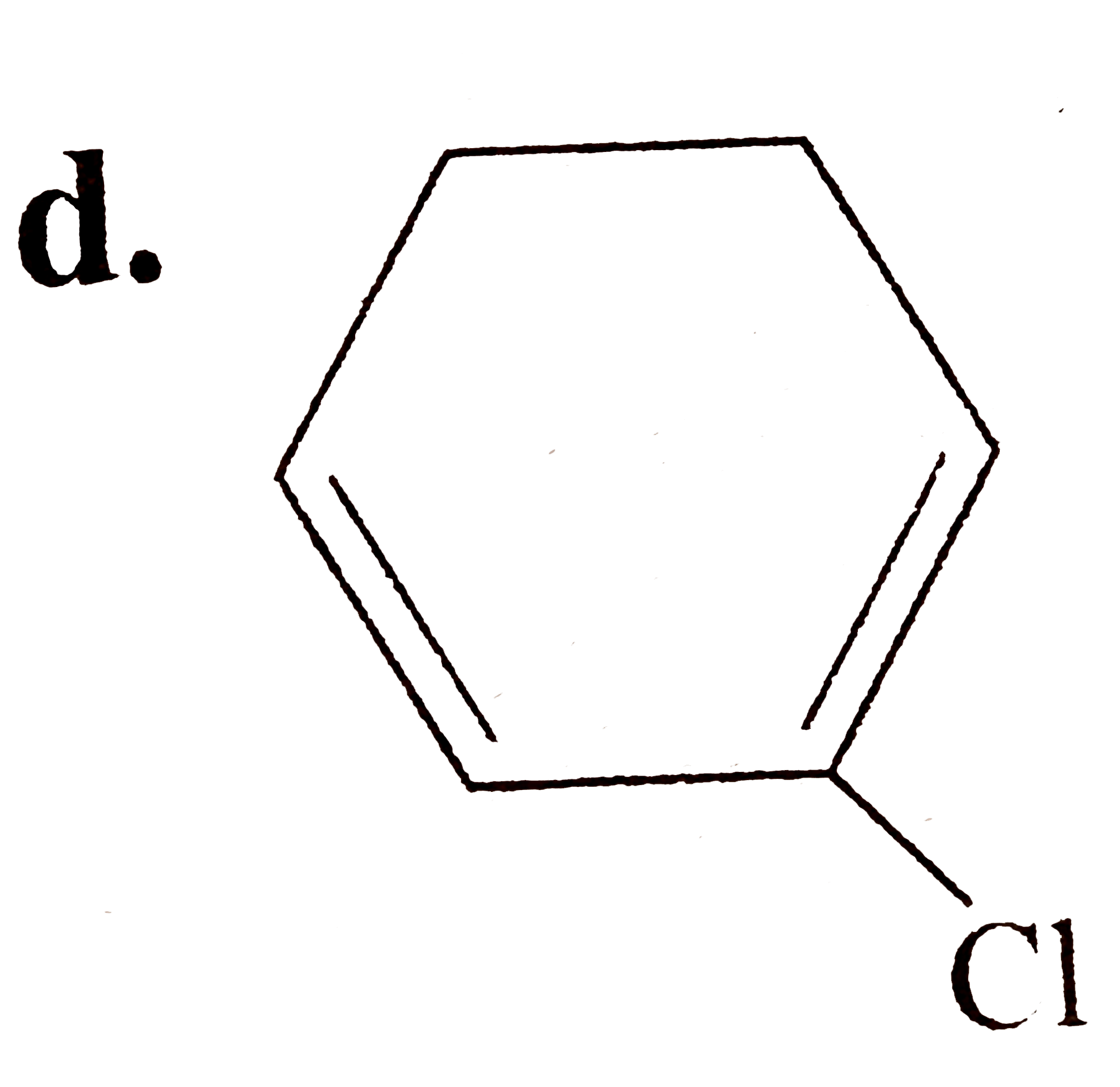
The correct Answer is:b.
Anti− elimination of H and Cl.
Topper's Solved these Questions
- ALKENES AND ALKADIENESBOOK - CENGAGE CHEMISTRYCHAPTER - ALKENES AND ALKADIENESEXERCISE - Multiple Correct Answer Type
41 Videos
- ALKENES AND ALKADIENESBOOK - CENGAGE CHEMISTRYCHAPTER - ALKENES AND ALKADIENESEXERCISE - Single Correct Answer Type
62 Videos
- ALKENES AND ALKADIENESBOOK - CENGAGE CHEMISTRYCHAPTER - ALKENES AND ALKADIENESEXERCISE - EXERCISES
29 Videos
- ALKANES AND CYCLOALKANESBOOK - CENGAGE CHEMISTRYCHAPTER - ALKANES AND CYCLOALKANESEXERCISE - Archives
13 Videos
- ALKYNESBOOK - CENGAGE CHEMISTRYCHAPTER - ALKYNESEXERCISE - Exercises (Archives - Analytical and Desriptive Type)
4 Videos
Similar Questions
If D1andD2 are two 3×3 diagonal matrices, then which of the following is/are true? D1andD2 is a diagonal matrix b. D1D2=D2D1 c. D12+D22 is a diagonal matrix d. none of these
View SolutionIfD=diag[d1,d2,dn] , then prove that f(D)=diag[f(d1),f(d2),,f(dn)],wheref(x) is a polynomial with scalar coefficient.
View Solution
Knowledge Check
If D=diag[d1,d2,…………..,dn] where di≠0∀i=1,2,3,………..n then D−1 is equal to
ADiagonal matrixBInCdiag(d−11,d−12,…..d−1n)DNoneIf D=diag[d1,d2,d3,…,dn], where di≠0,Aai=1,2….,n,then D−1 is equal to
AOBInCdiag[d−11,d−12,…..,d−1n]DNone of the aboveIf D=diag(d1,d2,d3,…,dn) where d≠0 for all I=1,2,…,n, then D−1is equal to
ADBdiag(d−11d−12,...,d−1n)CInDnone of these
If D1andD2 are diagonal matices of order 3×3 then (A) Dn1 is a diagonal matrix (B) D1D2=D2D1 (C) D21+D22 is diagonal matrix (D) D1D2 is a diagonal matix
View SolutionFind the inverse of each of the matrices given below :
Let D=diag[d1,d2,d3] where none of d1,d2,d3 is ), prove that
D−1=diag[d−11,d−12,d−13].
View Solution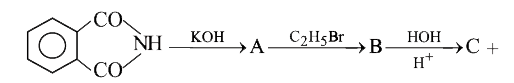
D,C and D in the sequence are :
View SolutionThe vaue of (ds+1÷d⋅ds) would be
View SolutionIf A=diag[d1,d2,d3] then An is equal to
View Solution
