A solid sphere having volume V and density ρ floats at the interface of two immiscible liquids of densityes ρ1 and ρ2 respectively. If ρ1<ρ<ρ2, then the ratio of volume of the parts of the sphere in upper and lower liquid is
(rho-rho_(1))/(rho_(2)-rho)
ρ2−ρρ−ρ1
(rho+rho_(1))/(rho+rho_(2))
(rho+rho_(2))/(rho+rho_(1))
The correct Answer is:B
To solve the problem of a solid sphere floating at the interface of two immiscible liquids, we will follow these steps:
Step 1: Understand the Problem
We have a solid sphere with volume V and density ρ floating at the interface of two immiscible liquids with densities ρ1 (upper liquid) and ρ2 (lower liquid). Given that ρ1<ρ<ρ2, we need to find the ratio of the volumes of the parts of the sphere submerged in the upper and lower liquids.
Step 2: Define Variables
Let:
- V1 = Volume of the sphere submerged in the upper liquid (density ρ1)
- V2 = Volume of the sphere submerged in the lower liquid (density ρ2)
Step 3: Apply Archimedes' Principle
According to Archimedes' principle, the buoyant force (upthrust) acting on the sphere is equal to the weight of the liquid displaced by the submerged parts of the sphere. Therefore, we can write:
U1+U2=mg
Where:
- U1=V1⋅ρ1⋅g (upthrust from the upper liquid)
- U2=V2⋅ρ2⋅g (upthrust from the lower liquid)
- mg=V⋅ρ⋅g (weight of the sphere)
Step 4: Set Up the Equation
Substituting the expressions for U1 and U2 into the equation gives:
V1⋅ρ1⋅g+V2⋅ρ2⋅g=V⋅ρ⋅g
We can cancel g from all terms (since g≠0):
V1⋅ρ1+V2⋅ρ2=V⋅ρ
Step 5: Relate Volumes
Since the total volume of the sphere is the sum of the submerged volumes:
V=V1+V2
Step 6: Solve for the Ratios
From the equations we have:
1. V1⋅ρ1+V2⋅ρ2=V⋅ρ
2. V=V1+V2
We can express V2 in terms of V1:
V2=V−V1
Substituting V2 into the first equation:
V1⋅ρ1+(V−V1)⋅ρ2=V⋅ρ
Expanding this gives:
V1⋅ρ1+V⋅ρ2−V1⋅ρ2=V⋅ρ
Rearranging terms:
V1(ρ1−ρ2)=V⋅ρ−V⋅ρ2
Factoring out V:
V1(ρ1−ρ2)=V(ρ−ρ2)
Thus,
V1=V(ρ−ρ2)(ρ1−ρ2)
Now substituting V1 back into the equation for V2:
V2=V−V1=V−V(ρ−ρ2)(ρ1−ρ2)
Step 7: Find the Ratio
Now we can find the ratio V1V2:
V1V2=V(ρ−ρ2)(ρ1−ρ2)V−V(ρ−ρ2)(ρ1−ρ2)
This simplifies to:
V1V2=ρ2−ρρ−ρ1
Final Answer
Thus, the ratio of the volume of the parts of the sphere in the upper and lower liquids is:
V1V2=ρ2−ρρ−ρ1
We have a solid sphere with volume V and density ρ floating at the interface of two immiscible liquids with densities ρ1 (upper liquid) and ρ2 (lower liquid). Given that ρ1<ρ<ρ2, we need to find the ratio of the volumes of the parts of the sphere submerged in the upper and lower liquids.
Let:
- V1 = Volume of the sphere submerged in the upper liquid (density ρ1)
- V2 = Volume of the sphere submerged in the lower liquid (density ρ2)
According to Archimedes' principle, the buoyant force (upthrust) acting on the sphere is equal to the weight of the liquid displaced by the submerged parts of the sphere. Therefore, we can write:
- U1=V1⋅ρ1⋅g (upthrust from the upper liquid)
- U2=V2⋅ρ2⋅g (upthrust from the lower liquid)
- mg=V⋅ρ⋅g (weight of the sphere)
Substituting the expressions for U1 and U2 into the equation gives:
Since the total volume of the sphere is the sum of the submerged volumes:
From the equations we have:
2. V=V1+V2
Now we can find the ratio V1V2:
Thus, the ratio of the volume of the parts of the sphere in the upper and lower liquids is:
Topper's Solved these Questions
- FLUID MECHANICSBook:A2ZChapter:FLUID MECHANICSExercise:Chapter Test
Explore 29 Videos
- FLUID MECHANICSBook:A2ZChapter:FLUID MECHANICSExercise:NEET Questions
Explore 7 Videos
- GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONBook:A2ZChapter:GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONExercise:Chapter Test
Explore 30 Videos
Similar Questions
V1 and V2 are the velocities of sound at the same temperature in two monoatomic gases of densities ρ1 and ρ2 respectively.If ρ1ρ2=14 then the ratio of velocities V1 and V2 is
View SolutionThe light cone is in equilibrium under the action of hydrostatic forces of two liquids of densities ρ1 and ρ2. Find ρ1/ρ2. Find ρ1 and ρ2.
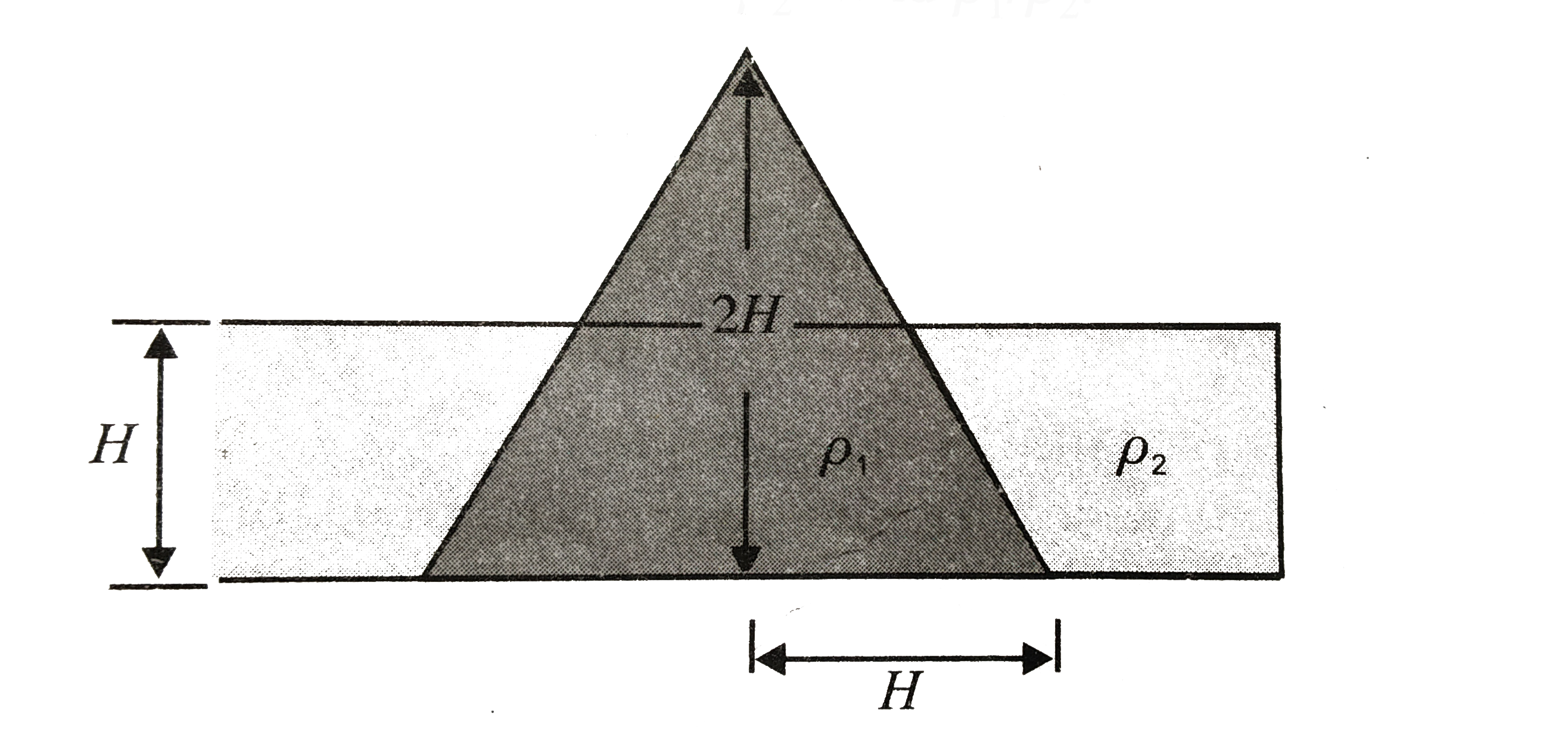
View Solution
Knowledge Check
A solid uniform ball having volume V and density ρ floats at the interface of two unmixable liquids as shown in the figure. The densities of the upper and the lower liquids are ρ1 and ρ2 respectively, such that ρ1<ρ<ρ2 . What fraction of the volume of the ball will be in the lower liquid ?
 Aρ−ρ2ρ1−ρ2Bρρ1−ρ2Cρ1−ρρ1−ρ2Dρ1−ρ2ρ2
Aρ−ρ2ρ1−ρ2Bρρ1−ρ2Cρ1−ρρ1−ρ2Dρ1−ρ2ρ2A solid uniform ball having volume V and density ρ floats at the interface of two unmixible liquids as shown in Fig. 7(CF).7. The densities of the upper and lower liquids are ρ1 and ρ2 respectively, such that ρ1<ρ<ρ2. What fractio9n of the volume of the ball will be in the lower liquid.
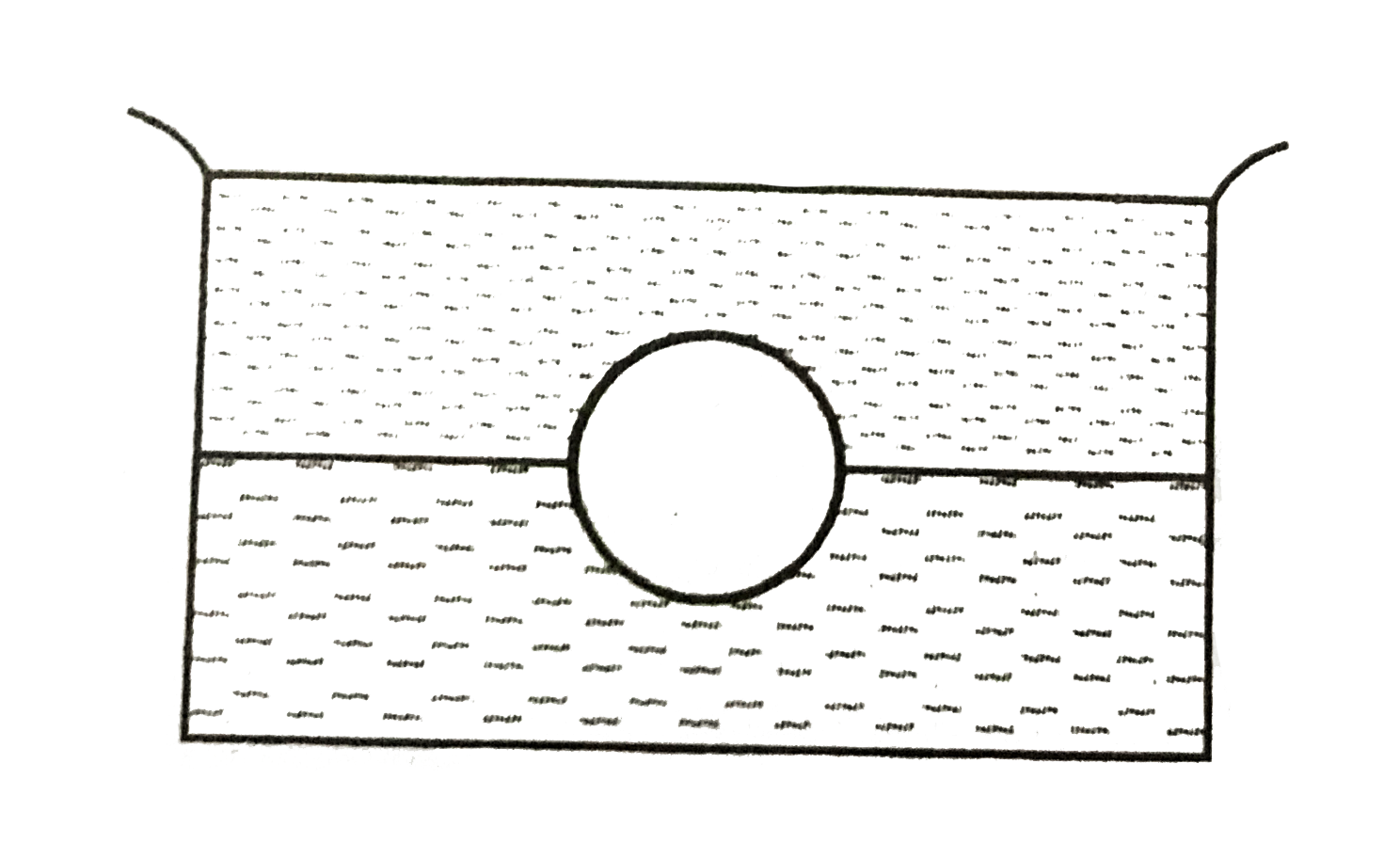 Aρ−ρ2ρ1−ρ2Bρ1ρ1−ρ2Cρ1−ρρ1−ρ2Dρ1−ρ2ρ2
Aρ−ρ2ρ1−ρ2Bρ1ρ1−ρ2Cρ1−ρρ1−ρ2Dρ1−ρ2ρ2A solid sphere of radius r is floating at the interface of two immiscible liquids of densities ρ1 and ρ2(ρ2>ρ1), half of its volume lying in each. The height of the upper liquid column from the interface of the two liquids is h. The force exerted on the sphere by the upper liquid is (atmospheric pressure =p0 and acceleration due to gravity is g):
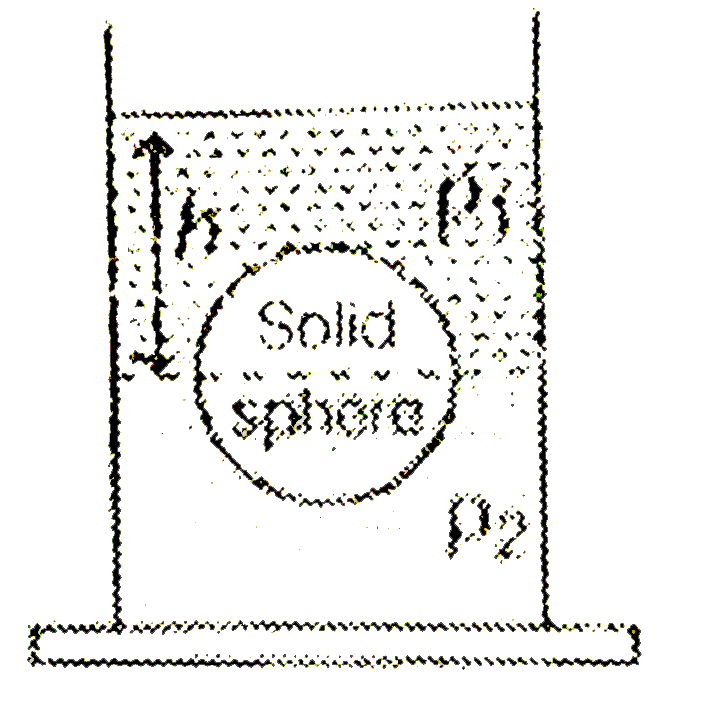 Ap0πr2+(h−23r)πr2ρ1gB(h−23r)πr2ρ1gC23πr2ρ1gDp0×πr2
Ap0πr2+(h−23r)πr2ρ1gB(h−23r)πr2ρ1gC23πr2ρ1gDp0×πr2
If two liquids of same mass but densities ρ1 and ρ2 respectively are mixed, then the density of the mixture is:
View SolutionIf two liquids of same masses but densities ρ1andρ2 respectively are mixed, then the density of mixture is given by
View SolutionIf two liquids of same masses but densities ρ1andρ2, respectively are mixed, then the density of mixture is given by
View SolutionTwo disc has same mass rotates about the same axis with their densities ρ1 and ρ2 respectively such that (ρ1>ρ2), then the relation between I1 and I2 will be
View SolutionThe velocities of sound at same temperature in two monoatomic gases of density ρ1 and ρ2 are v1 and v2 repectively , if ρ1ρ2=4, then the value of v1v2 will be
View Solution