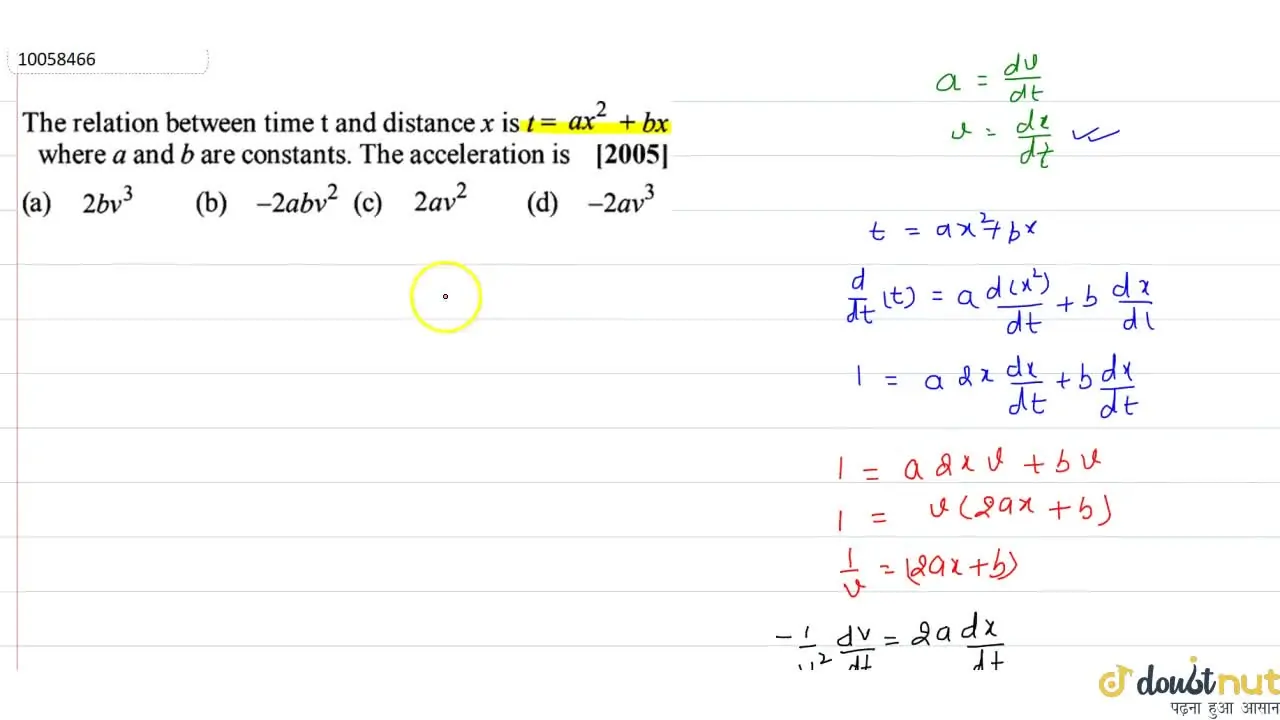
The relation between time $t$ and distance $x$ is $t = a x^{2} + b x$ where $a and$ b are constants. The acceleration is

Video Solution

Text Solution

Verified by Experts

Here, $t = a x^{2} + b x$
Differentiatng both the dises w.r.t. time (t0, we get $1 = 2 a x \frac{d x}{d t} + b \frac{d x}{d t} = (2 a x + b) \frac{d x}{d t}$ ltbRgt or $\frac{d x}{d t} = \frac{1}{(2 a x + b)}$
velocity , $v =) d x \frac{)}{d t} = \frac{1}{(2 a x + b)} = (2 a x + b))^{1}$
Acceleration $A = \frac{d v}{d t} = \frac{d}{d t} {[2 a x + b)}^{- 1}]$
$= - {(2 a x + b)}^{- 2} \times 2 a \frac{d x}{d t}$
$= \frac{- 2 a}{{(2 a x + b)}^{2}} \times \frac{1}{(2 a x + b)}$
$= \frac{- 2 a}{{((2 a x + b))}^{3}}$
$= - 2 a v^{3}$ [:. 1/((2 ax+b))=v].

|

Updated on:21/07/2023

Class 11 PHYSICS MOTION IN A STRAIGHT LINE

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
The relation between time t and displacement x is $t = α x^{2} + β x,$ where $α and β$ are constants. The retardation is
A $2 α v^{3}$
B $2 β v^{3}$
C $2 α β v^{3}$
D $2 β^{2} v^{3}$
Question 2 - Select One
The relation between time $t$ and distance $x$ is $t = a x^{2} + b x$ where $a and$ b are constants. The acceleration is
A $2 b v^{3}$
B $2 a v^{3}$
C $- 2 a v^{2}$
D $- 2 a v^{3}$
Question 3 - Select One
The relation between time $t$ and distance $x$ of a particle is given by $t = A x^{2} + B x$ , where $A$ and $B$ are constants. Then the
$(A)$ velocity is given by $v = 2 A x + B$
$(B)$ velocity is given by $v = {(2 A x + B)}^{- 1}$
$(C)$ retardation is given by $2 A v^{2}$
$(D)$ retardation is given by $2 B v^{2}$
Select correct statement $: -$
AOnly $C$
BOnly $D$
COnly $A$ & $D$
DOnly $B$ & $C$

PRADEEP-KINEMATICS-2 Problems for practice

Home
Ask Doubt
Profile