Two particles are moving with constant speed such that they are always at a constant distance apart and their velocities are always equal and opposite. After what time will they return to their initial positions ?
Since, the particles while moving with constant speed (v) are always at a constant distance (d), they must be at the two ends of the diameter of a circular path. The diameter of this circular path . Each particle will return to its initial position after describing a circular path
( :. 2 r =d) Time interval after which each particle will return to its intial position
=distance travelled//speed =pi d//v.
Topper's Solved these Questions
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 2 Short answer
26 Videos
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 3 Short answer
37 Videos
- KINEMATICSBOOK - PRADEEPCHAPTER - KINEMATICSEXERCISE - 6 Very short
4 Videos
- GRAVIATIONBOOK - PRADEEPCHAPTER - GRAVIATIONEXERCISE - Assertion-Reason Type Questions
19 Videos
- LAWS OF MOTIONBOOK - PRADEEPCHAPTER - LAWS OF MOTIONEXERCISE - Assertion- Reason Type Questions
17 Videos
Similar Questions
If a particle is moving with a constant velocity, what is its acceleration?
View SolutionA circle of radius unity is centered at thet origin. Two particles tart moving at the same time from the point (1,0) and move around the circle in opposite direction. One of the particle moves anticlockwise with constant speed v and the other moves clockwise with constant speed 3v. After leaving (1,0), the two particles meet first at a point P, and continue until they meet next at point Q. The coordinates of the point Q are
View Solution
Knowledge Check
If a particle moves with a constant speed, the distance-time graph is
Astraight lineBcircleCstairlike lineDpolygonIf a particle moves with a constant speed, the distance-time graph is
Astraight lineBcurved lineChorizontal line to time axisDparallel line to velocity axisA particle is moving with a constant speed v in a circle. What is the magnitude of average velocity after half rotation?
A2vBv(vπ)Cv2Dv2π
A particle is moving with constant speed v along x - axis in positive direction. Find the angular velocity of the particle about the point (0, b), when position of the particle is (a, 0).
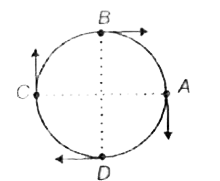
View SolutionFour particles A, B, C and D are moving with constant speed v each at the instant shown relative velocity of A with respect to B, C and D are in directions
View SolutionA particle is moving with constant speed v in xy plane as shown in figure. The magnitude of its angular velocity about point O is

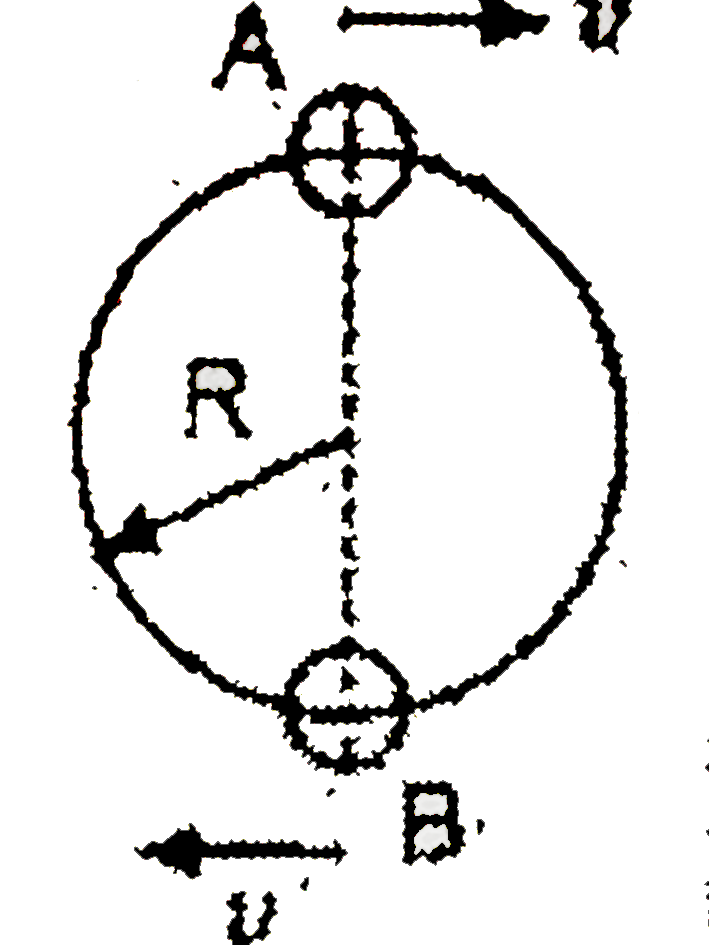
View SolutionTwo bodies are moving with constant speed v clockwise and are initially diagonally opposite. The particle B now acheives a tangential acceleration of a m/s2. Then :
View SolutionA particle moves with constant speed v along a circular path of radius r and completes the circle in time T. The acceleration of the particle is
View Solution
