the gauge pressure is both tyres of a bicycle is 690 k Pa. If the bicycle and the rider have a combined mass of 90 kg. what is the area of contact of each tyre with the ground? (assume that each tyre supports half the total weight of the bicycle.)
As F=12(90)(9.8)=441N,P=690×103Pa,A=FP=441N690×103Nm2=6.39×10−4m2
Topper's Solved these Questions
- FLOTATIONBOOK - PRADEEPCHAPTER - FLOTATIONEXERCISE - Oral Testing
21 Videos
- FLOTATIONBOOK - PRADEEPCHAPTER - FLOTATIONEXERCISE - Quiz Testing:Between Two Groups
9 Videos
- FLOTATIONBOOK - PRADEEPCHAPTER - FLOTATIONEXERCISE - PROBLEMS FOR PRACTICE
18 Videos
- FORCES AND LAWS OF MOTIONBOOK - PRADEEPCHAPTER - FORCES AND LAWS OF MOTIONEXERCISE - MOCK TEST
36 Videos
Similar Questions
The gauge prerssure in both the tyres of a bicycle is 7.0×105Pa If the bicycle and the person riding it have a combined mass of 100 kg. find the area of contact of each tyre with ground.Use g=10ms−2
View SolutionForce exerted by a truck is 500000N and the pressure on the ground is 2500000Pa. Calculate the area of contact of tyres with ground?
View Solution
Knowledge Check
The drwing shows a bicycle wheel of radius r, resting against a small step whose height is h=r/5. A clockwise torque increases, there comes a time when the wheel just begins to rise up and loses contact with the ground. Let this torque be T. What is the magniitude of the horizontal component of the acceleration of the centre of the wheel when a torque of 2T is applied? Assume that the wheel doesn't slip at the edge of the step when this torque is applied (ignore the mass of the spokes).
 A4.8m/s2B3m/s2C2.4m/s2D1.5m/s2
A4.8m/s2B3m/s2C2.4m/s2D1.5m/s2A simplified model of a bicycle of mass M has two tires that each comes into contact with the ground at a point. The wheel base of this bicycle is JV, and the centre of mass C of the bicycle is located mid way between the tires and a height h above the ground.The bicycleis moving to the right,but slowing down at a constant acceleration a. Air resistance may be ignored. Assuming that the coefficient of sliding friction between each tyre and the ground is μ and that both tyres are skidding (sliding without rotating). Express your answer in terms of w,h,M and g .
What is the maximum value of a so that both tyres remain in contact with the ground ?
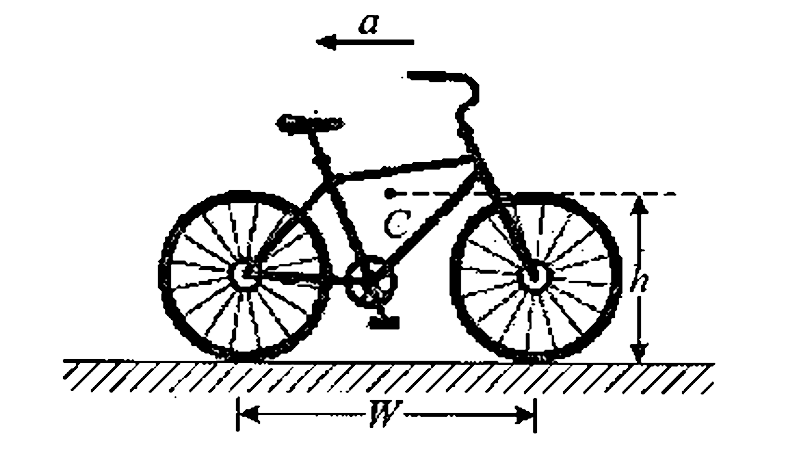 AWghBWg2hChg2WDh2Wg
AWghBWg2hChg2WDh2Wg
The weight of a rider, driving scooter is assumed to be evenly distributed on both the tyres.The area of contact of each type with the ground is 10cm2. If the pressure inside the tyres is 3 bar, find the mass of the rider (g=10ms−2)
View SolutionThe pressure inside a cycle tyre is 3.2×105dyne cm−2 . Area of contact of tyre with the ground is 2cm2 when the rider is on the seat. Find the weight of the rider, assuming the weight to be evenly distributed on both tyres of the cycle .
View SolutionThe menual of a car instructs the owner to inflate the tyres to pressure of 200 kPa. (a) What is the recommended gauge pressure ? (b) What is the recommended absolute pressure ? (c ) If after the required inflation of the tyres, the car is driven to a mountain peak. where the atmospheric pressrre is 10 % below that at sea level, what will be the tyre gauge read ? Atmospehric pressure =1.01×105Pa.
View SolutionA track consists of two circular pars ABC andCDE of equal rdius 100 m and joined smoothly as shown in figure.Each part sutends a right ngle at its centre. A cycle weighing 100 kg together with rider travels at a constant speed of 18 km/h on the track. A. Findteh nromal contct force by tehroad on the cycle whenit is at B and at D. b.Findteh force of friction exerted by the track on the tyres when the cycle is at B,C and D. c. Find the normal force between teh road and teh cycle just before and just after the cycle crosses C. d. What should be the minimum friction coefficient between the road and the tyre, which will ensure that teh cyclist can move with constant speed? Take g=10ms2

View SolutionConsider a vertical tube open at both ends. The tube consistss of two parts, each of different cross sections and each part having a piston which can move smoothly in respective tubes. The two piston which can move smoothly in respective tube wire. The piston are joined together by an inextensible wire. The combined mass of the two piston is 5kgand area of cross section of the upper piston is 10cm2 greater than that of the lower piston. Amount of gas enclosed by the pistons is 1mol. When the gas is heated slowly, pistons move by 50cm. Find the rise in the temperature of the gas in the form X/RK, where R is universal gas constant. Use g=10m/s2 and outside pressure =105N/m2).

View SolutionConsider a vertical tube open at bothh ends. The cube consists of two parts, each of different cross-sections and each parth having a piston which can move smoothly in respective tubes. The two pistons are joined together by an inextensible wire. The combined mass of the two piston is 5 kg and area of cross-section of the upper piston is 10cm2 greater than that of the lower piston. Amount of gas enclosed by the pistons is one mole. When the gas is heated slowly, pistons move by 50cm. Find rise in the temperature of the gas, in the form 25X/RK where R is universal gas constant. Use g=10m/s2 and outside pressure =105N/m2).
Fill value of X in the answer sheet.

View Solution
