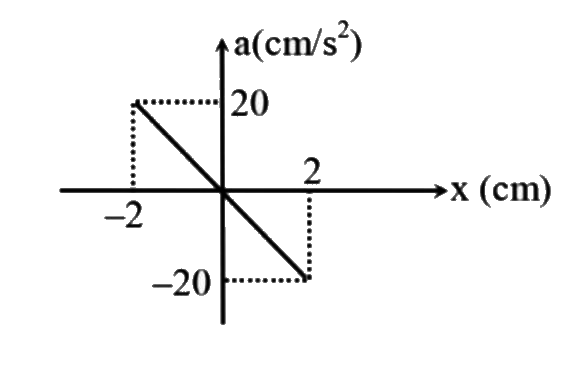
The acceleration displacement graph of a particle executing simple harmonic motion is shown in figure. The time period of simple harmonic motion is
4π√3s
2π√3s
The given graph doesn't represent simple harmonic motion
information is insufficient
The correct Answer is:A
To find the time period of a particle executing simple harmonic motion (SHM) from the acceleration-displacement graph, we can follow these steps:
1. Understand the Relationship:
In SHM, the acceleration a is related to the displacement x by the equation:
a=−ω2x
This indicates that the acceleration is directly proportional to the displacement but in the opposite direction.
2. Identify the Graph Type:
The graph of acceleration versus displacement is a straight line with a negative slope. This can be expressed in the form y=mx+c, where y is acceleration a and x is displacement x.
3. Determine the Slope:
The slope of the line m can be defined as:
m=dydx
Since the graph shows a negative slope, we can denote it as:
m=−ω2
4. Calculate the Slope from the Graph:
If the angle θ made with the horizontal is given (for example, 37∘), we can find the slope using:
m=−tan(θ)
For θ=37∘:
tan(37∘)=34
Therefore, the slope m becomes:
m=−34
5. Relate the Slope to Angular Frequency:
From the relationship m=−ω2, we have:
−ω2=−34
Thus, we can write:
ω2=34
6. Find Angular Frequency:
Taking the square root gives:
ω=√34=√32
7. Relate Angular Frequency to Time Period:
The angular frequency ω is related to the time period T by the equation:
ω=2πT
Therefore, we can rearrange this to find T:
T=2πω
8. Substitute the Value of ω:
Substituting ω=√32:
T=2π√32=4π√3
9. Final Answer:
The time period of the simple harmonic motion is:
T=4π√3
In SHM, the acceleration a is related to the displacement x by the equation:
a=−ω2x
This indicates that the acceleration is directly proportional to the displacement but in the opposite direction.
The graph of acceleration versus displacement is a straight line with a negative slope. This can be expressed in the form y=mx+c, where y is acceleration a and x is displacement x.
The slope of the line m can be defined as:
m=dydx
Since the graph shows a negative slope, we can denote it as:
m=−ω2
If the angle θ made with the horizontal is given (for example, 37∘), we can find the slope using:
m=−tan(θ)
For θ=37∘:
tan(37∘)=34
Therefore, the slope m becomes:
m=−34
From the relationship m=−ω2, we have:
−ω2=−34
Thus, we can write:
ω2=34
Taking the square root gives:
ω=√34=√32
The angular frequency ω is related to the time period T by the equation:
ω=2πT
Therefore, we can rearrange this to find T:
T=2πω
Substituting ω=√32:
T=2π√32=4π√3
The time period of the simple harmonic motion is:
T=4π√3
Topper's Solved these Questions
- OSCILLATION AND SIMPLE HARMONIC MOTIONBOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Velocity , Acceleration And Energy Of Simple Harmonic Motion
40 Videos
- OSCILLATION AND SIMPLE HARMONIC MOTIONBOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Spring Particle System
24 Videos
- NEWTONS LAWS OF MOTIONBOOK - A2ZCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Chapter Test
30 Videos
- PROPERTIES OF MATTERBOOK - A2ZCHAPTER - PROPERTIES OF MATTEREXERCISE - Chapter Test
29 Videos
Similar Questions
Knowledge Check
The velocity of a particle executing simple harmonic motion is
Aω2√A2+x2Bω√A2−x2Cω√A2+x2Dω2√A2−x2While a particle executes linear simple harmonic motion
Aits linear velocity and acceleration pass through their maximum and minimum values once in each oscillation.BIts linear velocity and acceleration pass through their maximum and minimum values twice in each oscillation.Cits linear velocity and acceleration pass through their maximum and minimum values four times in each oscillation.Dits linear velocity and acceleration always attain their peak values simlataneaously.The phase of a particle executing simple harmonic motion is π2 when it has
AMaximum velocityBMaximum accelerationCMaximum energyDMaximum displacement
The acceleration-displacement (a−X) graph of a particle executing simple harmonic motion is shown in the figure. Find the frequency of oscillation.

View SolutionThe plot of velocity (v) versus displacement (x) of a particle executing simple harmonic motion is shown in figure. The time period of oscillation of particle is :-

View SolutionThe displacement of a particle in simple harmonic motion in one time period is
View SolutionIf a body is executing simple harmonic motion, then
View SolutionThe displacement - time (x−t) graph of a particle executing simple harmonic motion is shown in figure. The correct variation of net force F action on the particle as a function of time is

View Solution