A boby is moving in a room with a velocity of 20m/s perpendicular to the two walls separated by 5 meters .There is no friction and the collision with the walls are elastic.
Not periodic
Periodic but not simple harmonic
Priodic and simple harmonic
Periodic with variable time period
The correct Answer is:B
Body collides elastically with wolls of room So, will be no loss energy and it will remain colliding with wolts of room, so its motion will be periodic .Then is no change in energy of the body hence there is no acceleration so it motion to not SHM
Topper's Solved these Questions
- OSCILLATION AND SIMPLE HARMONIC MOTIONBOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Velocity , Acceleration And Energy Of Simple Harmonic Motion
40 Videos
- OSCILLATION AND SIMPLE HARMONIC MOTIONBOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Spring Particle System
24 Videos
- NEWTONS LAWS OF MOTIONBOOK - A2ZCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Chapter Test
30 Videos
- PROPERTIES OF MATTERBOOK - A2ZCHAPTER - PROPERTIES OF MATTEREXERCISE - Chapter Test
29 Videos
Similar Questions
0A body of mass 20 kg moving with a velocity of 3 m/s, rebounds on a wall with same velocity. The impulse on the body is -
View SolutionA sphere of mass m slides with velocity v on as frictionless surface towards a smooth inclined wall as shown in figure. If the collision with the wall is perfectly elastic find a. the impulse imparted by the wall on the sphere b the impulse imparted by the floor on the sphere.
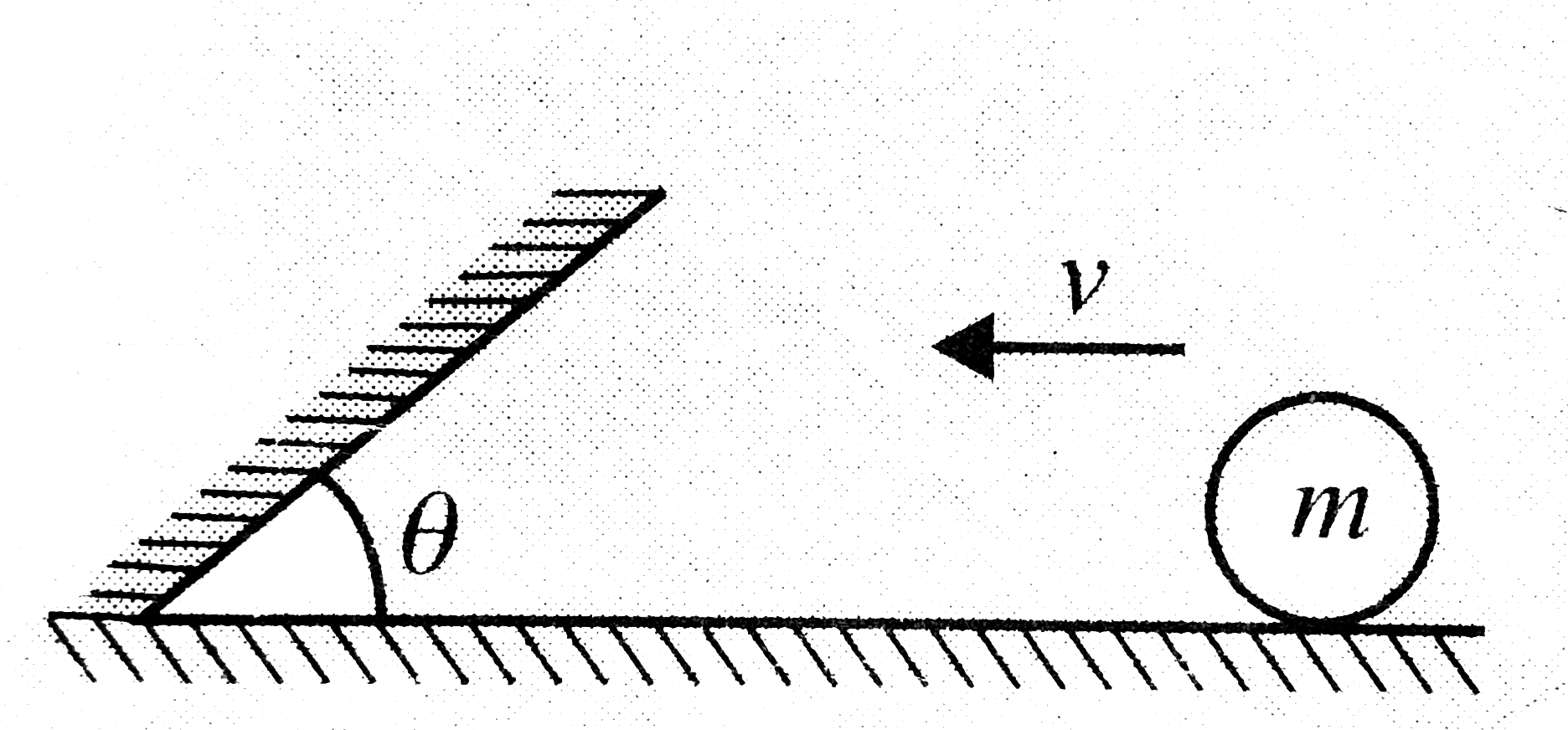
View Solution
Knowledge Check
A boby is moving in a room with a velocity of 20m/s perpendicular to the two walls separated by 5 meters .There is no friction and the collisionnn with the walls are elastic.
ANot periodicBPeriodic but not smple harmonicCPeriodic and simple harmonicDPeriodic with variable time periodA vessel contains 6×1026 molecules m−3. Mass of each molecule is 6×10−27kg. Assume that, on an average, one-sixth of the molecules move with a velocity103 m/s perpendicularly towards each wall. If the collisions with the walls are perfectly elastic, then which of the following is correct?
AChange in momentum of each molecule is 12×10−24 kg m/s in each collisionBThe number of molecules hitting normally to1 m2 of the wall per second is 1029CTotal change in momentum of all molecules per second is1031 SI unitsDThe number of molecules hitting one square metre of the surface is 6×1029A parallel beam of particles of mass m moving with velocity v impinges on a wall at an angle θ to its normal. The number of particles per unit volume in the beam is n. If the collision of particles with the wall is elastic, then the pressure exerted by this beam on the wall is
A2mnv2cosθB2mnv2cos2θC2mnvcosθD2mnvcos2θ
Two particles A and B separated by 10 m at time t=0 are moving uniformly. A is moving along line AB at a constant velocity of 4m/s and B is moving perpendicular to the velocity of A at a constant velocity of 5m/s. After what time the two particles will be nearest to each other?

View SolutionA particle of mass m moving on a smooth surface with velocity 10m/s strikes another particle of mass 2m moving with 5m/s in the same direction . If the collision is elastic and head - on , find velocities of particles after the collision.
View SolutionA ball of mass 10 kg is moving with a velocity of 10 m / s . It strikes another ball of mass 5 kg which is moving in the same direction with a velocity of 4 m / s . If the collision is elastic, their velocities after the collision will be, respectively
View SolutionA wall moving with velocity 2cms−1 towards the ball and ball is moving towards the wall with a velocity 10cms−1. It hits the wall normally and makes elastic collision with wall. The velocity of ball after collision with wall in cms−1.
View SolutionA bead of mass m and diameter d is sliding back and forth with velocity v on a wire held between two right walls of length L. Assume that th ecollisions with the wall are perfectly elastic and ther is no friction. The average force that the bouncing bead exerts on the one of the walls is :_
View Solution
