Question

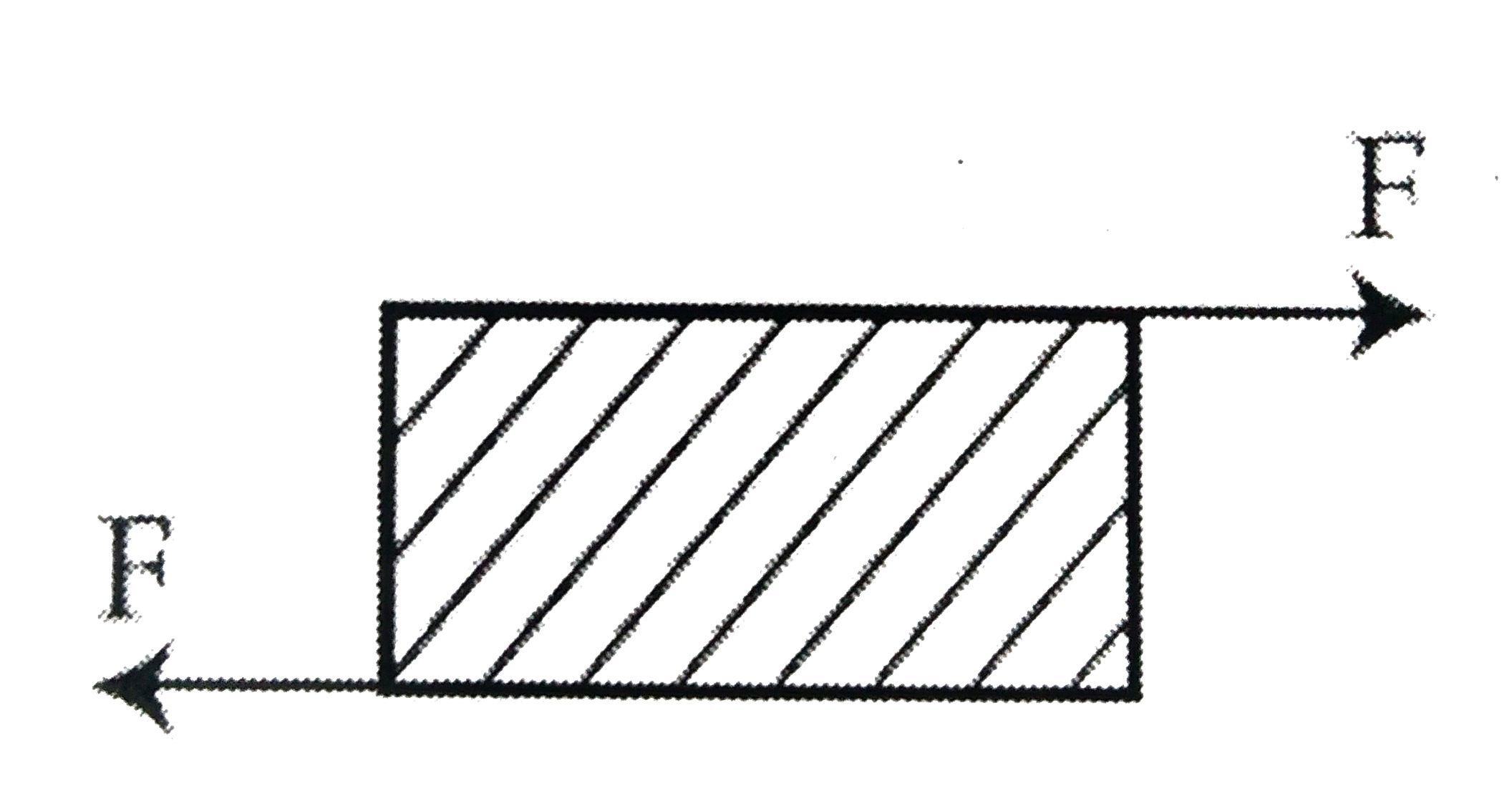
Two parallel and opposite forces each 5000 N are applied tangentially to the upper and lower faces of a cubical metal block of side 25 cm. the angle of shear is (The shear modulus of the metal is 80 Gpa)

Options

A. `10^-4rad`
B. `10^-5rad`
C. `10^-6rad`
D. `10^-7rad`

Answer

C

Explanation

To solve the problem, we will follow these steps:

Step 1: Understand the Given Information
We have:
- Two parallel and opposite forces, \( F = 5000 \, \text{N} \)
- Side of the cubical block, \( L = 25 \, \text{cm} = 0.25 \, \text{m} \)
- Shear modulus of the metal, \( G = 80 \, \text{GPa} = 80 \times 10^9 \, \text{Pa} \)

Step 2: Calculate the Area of the Block
The area \( A \) on which the forces are acting is given by:
\( A = L^2 = (0.25 \, \text{m})^2 = 0.0625 \, \text{m}^2 \)

Step 3: Calculate the Shear Stress
Shear stress \( \tau \) is defined as the force per unit area:
\( \tau = \frac{F}{A} = \frac{5000 \, \text{N}}{0.0625 \, \text{m}^2} = 80000 \, \text{Pa} = 80 \, \text{kPa} \)

Step 4: Relate Shear Stress to Shear Strain
The shear modulus \( G \) is defined as:
\( G = \frac{\tau}{\phi} \)
where \( \phi \) is the angle of shear (in radians).

Step 5: Rearrange to Find the Shear Angle
Rearranging the equation gives:
\( \phi = \frac{\tau}{G} \)

Step 6: Substitute the Values
Substituting the values we have:
\( \phi = \frac{80000 \, \text{Pa}}{80 \times 10^9 \, \text{Pa}} = \frac{80000}{80 \times 10^9} = \frac{1}{10^6} = 10^{-6} \, \text{radians} \)

Step 7: Conclusion
The angle of shear \( \phi \) is:
\( \phi = 10^{-6} \, \text{radians} \)