A solid sphere is rolling on a frictionless surface, shown in figure with a translational velocity $v m / s$ . If it is to climb the inclined surface then $v$ should be :

A

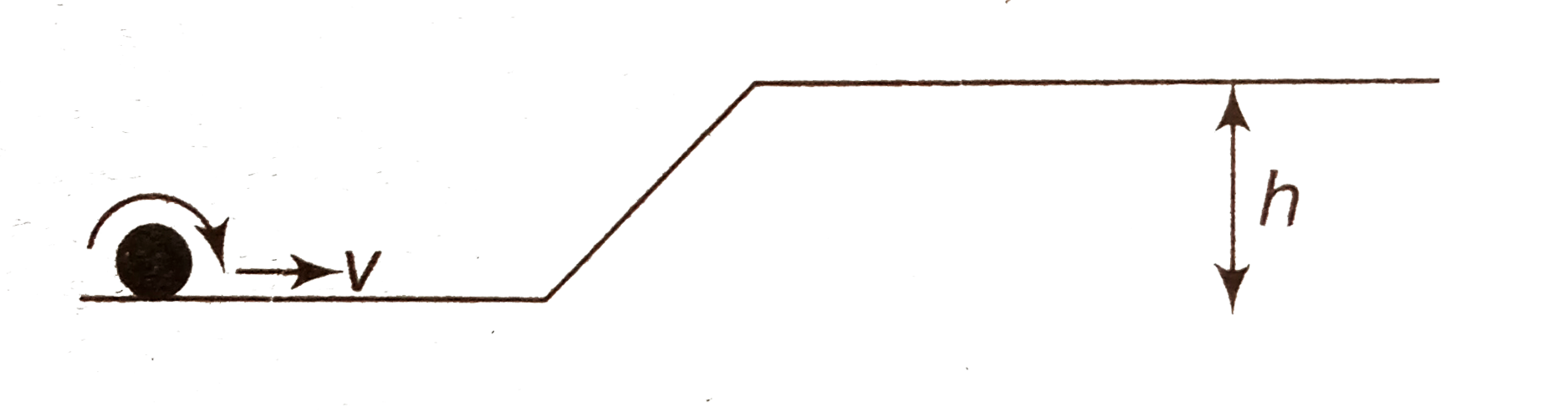
$\geq \sqrt{2 g h}$

B

$2 g h$

C

$\geq \sqrt{\frac{10}{7} g h}$

D

$\frac{10}{7} g h$

Video Solution

Text Solution

Verified by Experts

The correct Answer is:C

( c) From law of conservation of energy can neither be created nor be destroyed but it remains conserved In the given case the sum of kinetic energy of rotation and translation is converted to potential energy.
Also moment of inertia of disc is
$I = \frac{2}{5} M R 62$
$\frac{1}{2} m v_{Translatonal kinetic energy}^{2} + \frac{1}{2} I ω_{R o t a t i o n a l e \neq r g y}^{2} = m g h_{Potential energy}$
$\Rightarrow \frac{1}{2} m v^{2} + \frac{1}{2} (\frac{2}{5} M R^{2}) \frac{v^{2}}{R^{2}} = m g h$
where $v = R ω, ω$ = angular velocity
$\Rightarrow \frac{7}{10} m v^{2} = m g h$
$\Rightarrow v = \sqrt{\frac{10}{7}} g h$
Hence, to climb the inclined surface the velocity should be greater than $\sqrt{\frac{10}{7}} g h$ .

|

Updated on:21/07/2023

Class 11 PHYSICS RIGID BODY DYNAMICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
A solid sphere is rolling on a frictionless surface, shown in figure with a translational velocity $v m / s$ . If is to climb the inclind surface then $v$ should be :

A(1) $\geq \sqrt{\frac{10}{7} g h}$
B $\geq \sqrt{2 g h}$
C $2 g h$
D $\frac{10}{7} g h$
Question 2 - Select One
A solid sphere is rolling down an inclined plane. Then the ratio of its translational kinetic energy to its rotational kinetic energy is
A2.5
B1.5
C1
D0.4
Question 3 - Select One
A sphere is moving on a smooth surface with linear speed $v_{0}$ and angular velocity $ω_{0}$ . It finds a rough inclined surface and it starts climbing up:

Aif $v_{0} > R ω_{0}$ friction force will act downwards
Bif $v_{0} < R ω_{0}$ , frictio force will act upwards
Cif $v_{0} = R ω_{0}$ no friction force will act
Dif all above case friction will act upwards

A2Z-ROTATIONAL DYNAMICS-AIIMS Questions

Home
Ask Doubt
Profile