A plumb bob is hung from the ceiling of a train compartment. The train moves on an inclined track of inclination 30∘ with horizontal. Acceleration of train up the plane is a=g/2 . The angle which the string supporting the bob makes with normal to the ceiling in equilibrium is.
30∘
tan−1(2/(√3)
tan−1(√3/2)
tan−1(2)
The correct Answer is:B
To solve the problem step by step, we will analyze the forces acting on the plumb bob and use the equilibrium conditions to find the angle the string makes with the normal to the ceiling of the train compartment.
Step 1: Understand the setup
- The train is moving up an inclined plane with an inclination of 30∘ and has an acceleration a=g2.
- The plumb bob is hanging from the ceiling of the train compartment, which is also inclined at 30∘.
Step 2: Identify the forces acting on the bob
In the frame of the train compartment:
1. The weight of the bob acts downward: W=mg.
2. The tension T in the string acts along the string.
3. A pseudo force acts on the bob due to the acceleration of the train, directed down the incline: Fpseudo=ma=m(g2).
Step 3: Resolve forces into components
- The weight of the bob can be resolved into two components:
- Perpendicular to the incline: W⊥=mgcos(30∘)
- Parallel to the incline: W∥=mgsin(30∘)
- The pseudo force can also be resolved into components:
- Perpendicular to the incline: Fpseudo⊥=mg2cos(30∘)
- Parallel to the incline: Fpseudo∥=mg2sin(30∘)
Step 4: Write the equilibrium equations
In equilibrium, the sum of forces in both the perpendicular and parallel directions must be zero.
1. Vertical (perpendicular to incline) equilibrium:
Tcos(θ)=mgcos(30∘)+mg2cos(30∘)
Simplifying gives:
Tcos(θ)=3mg2cos(30∘)
2. Horizontal (parallel to incline) equilibrium:
Tsin(θ)=mgsin(30∘)+mg2sin(30∘)
Simplifying gives:
Tsin(θ)=3mg2sin(30∘)
Step 5: Divide the equations to eliminate T
Dividing the vertical equation by the horizontal equation:
Tcos(θ)Tsin(θ)=3mg2cos(30∘)3mg2sin(30∘)
This simplifies to:
cot(θ)=cos(30∘)sin(30∘)
Using the values:
cot(θ)=√3212=√3
Thus:
θ=30∘
Step 6: Find the angle with respect to the normal
The angle that the string makes with the normal to the ceiling is:
ϕ=90∘−θ=90∘−30∘=60∘
Final Answer
The angle which the string supporting the bob makes with the normal to the ceiling in equilibrium is 60∘.
---
- The train is moving up an inclined plane with an inclination of 30∘ and has an acceleration a=g2.
- The plumb bob is hanging from the ceiling of the train compartment, which is also inclined at 30∘.
In the frame of the train compartment:
1. The weight of the bob acts downward: W=mg.
2. The tension T in the string acts along the string.
3. A pseudo force acts on the bob due to the acceleration of the train, directed down the incline: Fpseudo=ma=m(g2).
- The weight of the bob can be resolved into two components:
- Perpendicular to the incline: W⊥=mgcos(30∘)
- Parallel to the incline: W∥=mgsin(30∘)
- Perpendicular to the incline: Fpseudo⊥=mg2cos(30∘)
- Parallel to the incline: Fpseudo∥=mg2sin(30∘)
In equilibrium, the sum of forces in both the perpendicular and parallel directions must be zero.
Tcos(θ)=mgcos(30∘)+mg2cos(30∘)
Simplifying gives:
Tcos(θ)=3mg2cos(30∘)
Tsin(θ)=mgsin(30∘)+mg2sin(30∘)
Simplifying gives:
Tsin(θ)=3mg2sin(30∘)
Dividing the vertical equation by the horizontal equation:
Tcos(θ)Tsin(θ)=3mg2cos(30∘)3mg2sin(30∘)
This simplifies to:
cot(θ)=cos(30∘)sin(30∘)
Using the values:
cot(θ)=√3212=√3
Thus:
θ=30∘
The angle that the string makes with the normal to the ceiling is:
ϕ=90∘−θ=90∘−30∘=60∘
The angle which the string supporting the bob makes with the normal to the ceiling in equilibrium is 60∘.
Topper's Solved these Questions
- NEWTONS LAWS OF MOTIONBOOK - A2ZCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - AIIMS Questions
26 Videos
- MOTION IN TWO DIMENSIONBOOK - A2ZCHAPTER - MOTION IN TWO DIMENSIONEXERCISE - Chapter Test
29 Videos
- OSCILLATION AND SIMPLE HARMONIC MOTIONBOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Chapter Test
29 Videos
Similar Questions
A plumb bob of mass 1kg is hung from the ceiling of a train compartment.The train moves on an inclined plane with constant velocity. If the angle of inclined is 30∘.Find the angle made by the string with the normal to the ceiling .Also ,Find the tension in the string (g=10m/s2)
View SolutionA plumb bob is hung from the ceiling of a train compartment. If the train moves with an acceleration a along a straight horizontal track, the stirng suporting the bob makes an angle tan1(ag) with the normkal to the ceiling. Suppose the train moves on an inclined straight track with uniform moves on aninclined straight track with uniform velocity. If the angle of incline is tan−1(ag), the string again makes the same angle with the normal to the ceiling. Can a person sitting inside the compartment tell by lookign at the plumb line whether the train is accelerated on a horizontal straight track or it is going on an incline? If yes, how? If no, suggest a method to do so.
View Solution
Knowledge Check
Aa simple pendulum is suspended from the ceiling of a vehicle which moves down an inclined plane o inclination Θ. The period of oscilation of the pendulum is
A2π√LgcosθB2π√LcosθgC2π√LgsinθD2π√LsinθgA plumb bob is hanging from the ceiling of car. If the car move with acceleration a, the angle made by the string with the vertical is
Atan−1(ag)Btan−1(ga)Ccos−1(ga)Dcos−1(ag)A plumb line is suspended from a ceiling of a car moving with horizontal acceleration of a . What will be the angle of inclination with vertical
Atan−1(a/g)Btan−1(g/a)Ccos−1(a/g)Dcos−1(g/a)
A plumb line is suspended from a celling of a car moving with horizontal acceleration of a. What will be the angle of inclination with vertical?
View SolutionA simple pendulum is suspended from the ceiling of a train which is moving with an acceleration a .The angular of inclination of the pendulum from the vertical will be
View SolutionA small sphere is suspended by a string from the ceiling of a car. If the car beings to move with a constant acceleration a, the inclination of the string to the vertical is:
View SolutionA particle is projected with a certain velocity at an angle α above the horizontal from the foot of an inclined plane of inclination 30∘. If the particle strikes the plane normally then α is equal to
View SolutionA pendulum of mass m hangs from a support fixed to a trolley. The direction of the string when the trolley rolls up a plane of inclination α with acceleration a0 is
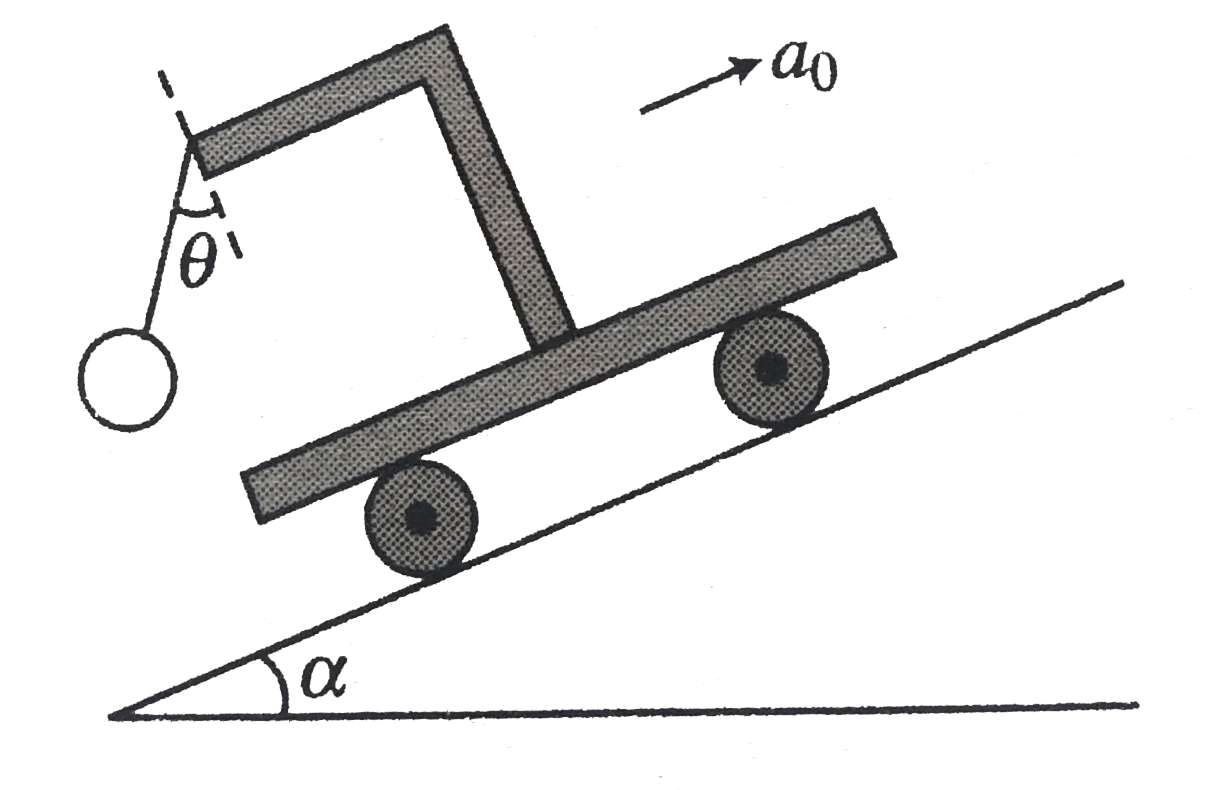
View Solution
