Question

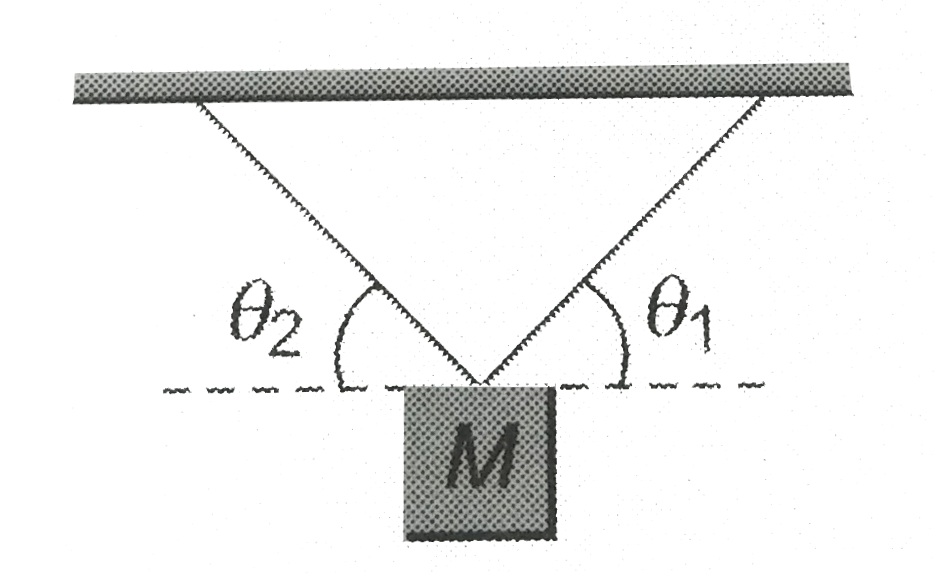
Two point particles with masses $m_1\text{and}{m}_2$ are thrown at angles ${\theta }_1\text{and}{\theta }_2$ with horizontal with speeds $v_1\text{and}{v}_2$ respectively. R, H and T are range, height and total time of flight respectively. Let $v_1{\sin \theta }_1=v_2{\sin \theta }_2$. Then for both particles

Options

A. T,H and R are different
B. H and R will be same but T will be different
C. T and R are same but H will be different
D. T and H are same but R is different.

Answer

D

Explanation

Relations for,
`T=(2u sin theta)/g, R=(u^(2)sin 2 theta)/g and H=(u^(2)sin^(2) theta)/(2g)`
Here, `u_(1)sin theta_(1)=u_(2) sin theta_(2)`
T and H are same but will not be same.