In a direct impact loss in kinetic energy is given by
ΔK=M1M22(M1+M2)(V1−V2)2(1−k2)
with usual notations (except k) The quantity k will have dimensional formula
[M0L2T−2]
[MLT−1]
[M0L0T0]
[M0T−1]
The correct Answer is:c
It is clear that
[1−k2]=M0L0T0
Hence [k]=M0L0T0
Actually k is the coefficient of restitution
Topper's Solved these Questions
- UNIT, DIMENSION AND ERROR ANALYSISBOOK - A2ZCHAPTER - UNIT, DIMENSION AND ERROR ANALYSISEXERCISE - Assertion Reasoning
16 Videos
- UNIT, DIMENSION AND ERROR ANALYSISBOOK - A2ZCHAPTER - UNIT, DIMENSION AND ERROR ANALYSISEXERCISE - NEET Questions
23 Videos
- UNIT, DIMENSION AND ERROR ANALYSISBOOK - A2ZCHAPTER - UNIT, DIMENSION AND ERROR ANALYSISEXERCISE - Erros Of Measurement
31 Videos
- THERMAL PROPERTIES OF MATTERBOOK - A2ZCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Chapter Test
30 Videos
- VECTORSBOOK - A2ZCHAPTER - VECTORSEXERCISE - Chapter Test
29 Videos
Similar Questions
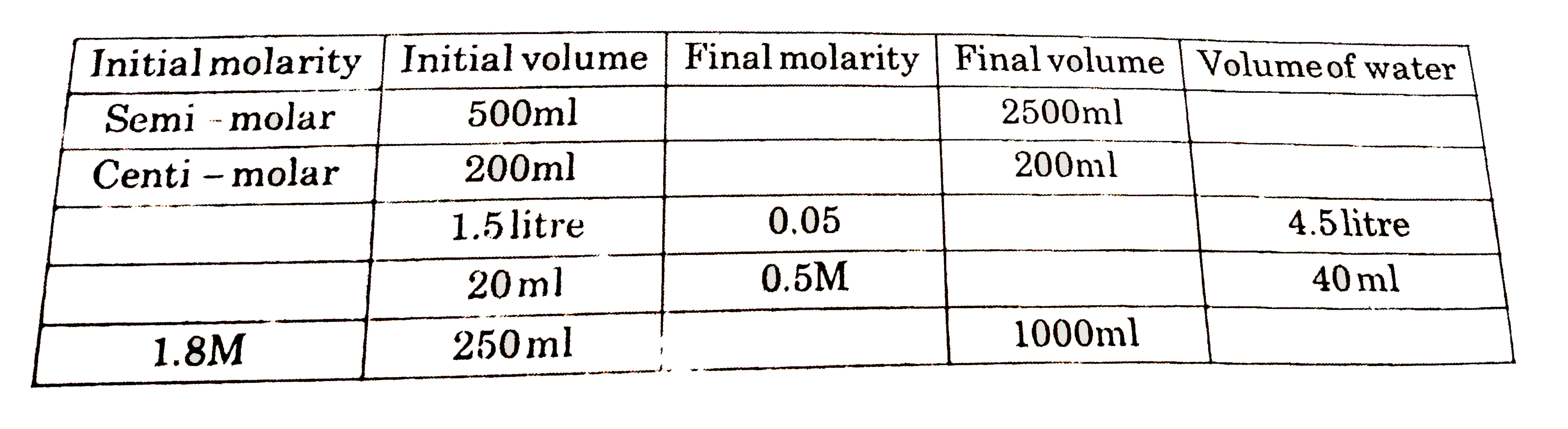
Use of dilution formula (M1V1=M2V2)
View SolutionTwo particles having masses m1andm2 are moving with velocities →V1and→V2 respectively. →V0 is velocity of centre of mass of the system.
(a) Prove that the kinetic energy of the system in a reference frame attached to the centre of mass of the system is KEcm=12μV2rel. Where μ=m1m2m1+m2 and Vrel is the relative speed of the two particles.
(b) Prove that the kinetic energy of the system in ground frame is given by
KE=KEcm+12(m1+m2)V20
(c) If the two particles collide head on find the minimum kinetic energy that the system has during collision.
View Solution
Knowledge Check
The kinetic energy of a moving body is given by k=2v2,k being in joules and v in m/s. It's momentum when travelling with a velocity of 2m/s will be (in kgms−1)
A16B4C8D2According to Maxwell−distribution law, the probability function representing the ratio of molecules at a particular velocity to the total number of molecules is given by
f(v)=k1√(m2πKT2)4πv2e−mv22KT
Where m is the mass of the molecule, v is the velocity of the molecule, T is the temperature k and k1 are constant. The dimensional formulae of k1 isAL2T−2BL1T−1K−3/2CL1T−1K+3/2DL2T−1K+3/2Two bodies P and Q of masses m1 and m2 (m2>m1) are moving with velocity v1 and v2 force exerted by P on Q during the collision is
Agreater that the force exerted by Q on PBless than the force exerted by Q on PCsame as the force exerted by Q on PDsam as the force exerted by Q on P but opposite in direction
If v=(3ˆi+2ˆj+6k) m/s and m=27kg then find kinetic energy (i.e.12mv2)
View Solution[k(k+1)2]2+(k+1)3=[(k+1)(k+2)2+1]2
View SolutionThe gravitational force between two point masses m1 and m2 at separation r is given by F=km1m2r2
The constant k doesn't
View SolutionFor a particle executing S.H.M., the kinetic energy K is given K=K0cos2ωt. The maximum value of potential energy is:
View SolutionM is a metal that forms an oxide M2O,12M2O→M+14O2ΔH=120 K. Cal. When a sample of metal M reacts with one mole of oxygen what will be the ΔH in that case
View Solution
