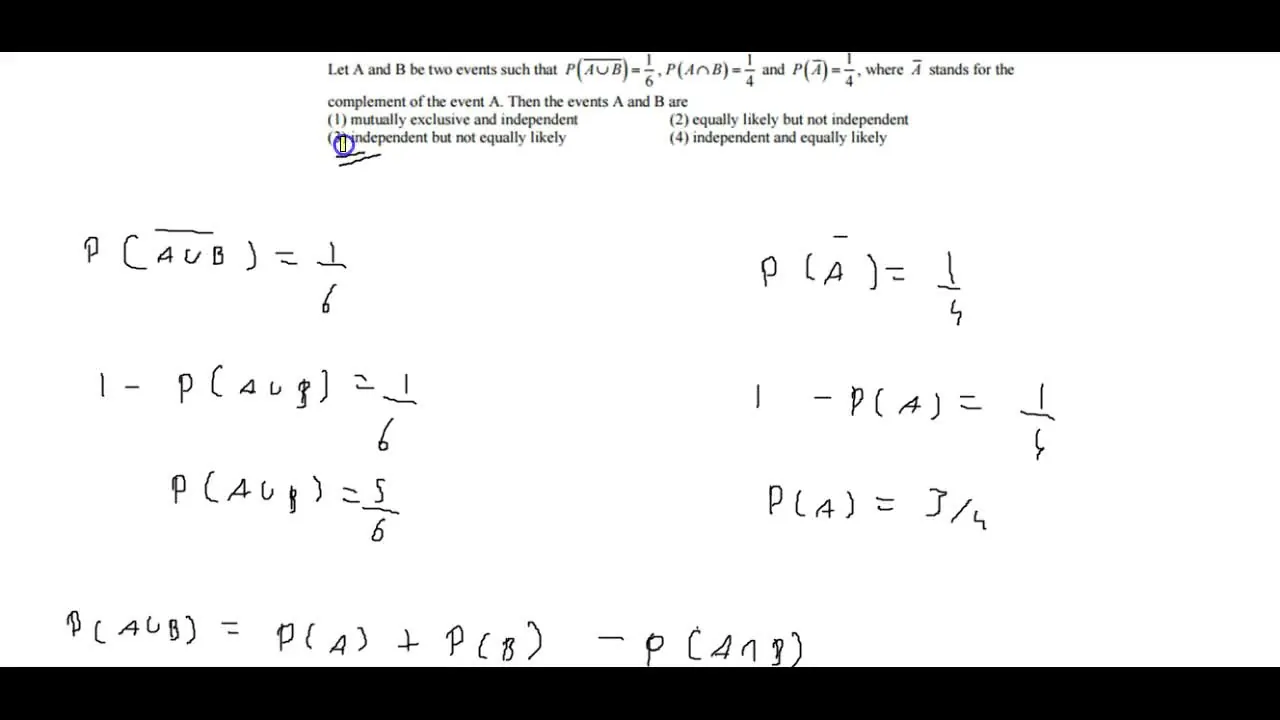
Let A and B be two events such that p(¯¯¯A∪B)=16,p(A∩B)=14 and p(¯¯¯A)=14 , where ¯¯¯A stands for the complement of the event A. Then the events A and B are (1) mutually exclusive and independent (2) equally likely but not independent (3) independent but not equally likely (4) independent and equally likely
P(¯¯¯¯¯¯¯¯¯¯A∪B=16
1−P(A∪B)=16
P(A∪B)=56
P(¯¯¯A)=14
1−P(A)=14
P(A)=34
P(A∪B)=P(A)+P(B)−P(A∩B)
56=34+P(B)−14
P(B)=56−12=26=13
P(A∩B)=P(A)P(B)
=34×13=14
A & B are independent events
P(A)=34
P(B)=13
P(A)≠P(B)
option 3 is correct
Related Playlists
Similar Questions
Let A and B be two events such that P(¯¯¯¯¯¯¯¯¯¯A∪B)=16,P(A∩B)=14andP(¯¯¯A)=14,where¯¯¯A stands for the complement of the event A. Then the events A and B are
View SolutionLet A and B be two events such that P(¯¯¯¯¯¯¯¯¯¯A∪B)=16,P(A∩B)=14andP(¯¯¯A)=14,where¯¯¯A stands for the complement of the event A. Then the events A and B are
View SolutionLet A and B be two events such that P¯¯¯¯¯¯¯¯¯¯¯¯¯(A∪B)=16,P(A∩B)=14andP(¯¯¯A)=14 where ¯¯¯A stands for complement of event A. Then, events A and B are
View SolutionLet A and B be two events such that
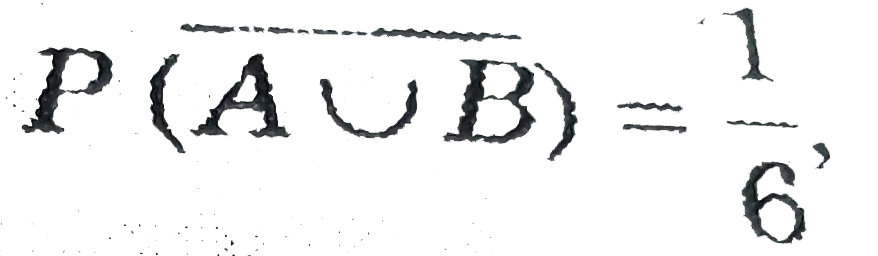 ,P(A cap B)=(1)/(4)andPoverset(-)(A)=(1)/(4),whereoverset(-)A stands for the complement of the event A. Then, the events A and B are View Solution
,P(A cap B)=(1)/(4)andPoverset(-)(A)=(1)/(4),whereoverset(-)A stands for the complement of the event A. Then, the events A and B are View SolutionLet A and B be two events such that P¯¯¯¯¯¯¯¯¯¯¯¯¯(A∪B)=16,P(A∩B)=14andP(¯¯¯A)=14,where ¯¯¯A stands for complement of event A. then , events A and B are
View SolutionIf A and B are any two events such that P(A∪B)=34,
P(A∩B)=14andP(¯¯¯A)=23, where ¯¯¯A stands for the complementary event of A, then what is P(B) ?View SolutionLet A and B be independent events such that P(A) =0.6 and P(B ) =0.4.
find P(A∩B)View SolutionLet A and B be independent events such that P(A) =0.6 and P(B ) =0.4.
find P(A∩B)View SolutionLet A and B be independent events such that P(A) =0.6 and P(B ) =0.4.
find P(A∩¯¯¯B)View SolutionLet A and B be events such that P(¯¯¯A)=45,P(B)=13,P(AB)=16 then
View Solution