
Two ports, A and B, on a north-south line ar separated by a river of width D. The river flows east with speed u. A boat crosses the river starting from port A. The speed of the boat relative to the river is v. Assume v=2u
Q. Suppose the boat wants to cross the river from A to the other side in the shortest possible time. Then what should be the direction of the velocity of boat relative to river?
30∘ west of north
30∘ east of north
60∘ west of north
along north
The correct Answer is:B
To cross the river the fastest, we need to maximize the y-component of the total velocity is VBcosθ So θ=0. The boat should head straight to the north.
Take positive x-axis along east and positive y-axis along north.
Topper's Solved these Questions
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICSCHAPTER - CENGAGE PHYSICS DPPEXERCISE - single correct type
14 Videos
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICSCHAPTER - CENGAGE PHYSICS DPPEXERCISE - subjective type
51 Videos
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICSCHAPTER - CENGAGE PHYSICS DPPEXERCISE - Fill in the blanks type
26 Videos
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICSCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Integer
5 Videos
- CENTRE OF MASS CONVERSATION OF MOMENTUM AND COLLISIONBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASS CONVERSATION OF MOMENTUM AND COLLISIONEXERCISE - Question Bank
38 Videos
Similar Questions
A boatman can row with a speed of 10kmh−1 in still water. River flows at 6kmh−1 . If he crosses the river from one bank to the other along the shortest possible path, time taken to cross that river of width 1 km is
View SolutionThe speed of boat is 5kmh−1 in still water. It crosses a river of width 1 km along the shortest possible path in 15 min. Then, velocity of river will be
View Solution
Knowledge Check

Two ports, A and B, on a north-south line ar separated by a river of width D. The river flows east with speed u. A boat crosses the river starting from port A. The speed of the boat relative to the river is v. Assume v=2u
Q. The boat crosses the river from A to the other side in shortest possible time, then how far is the boat from the port B after crossing the riverAD√2B√2DC2DDD2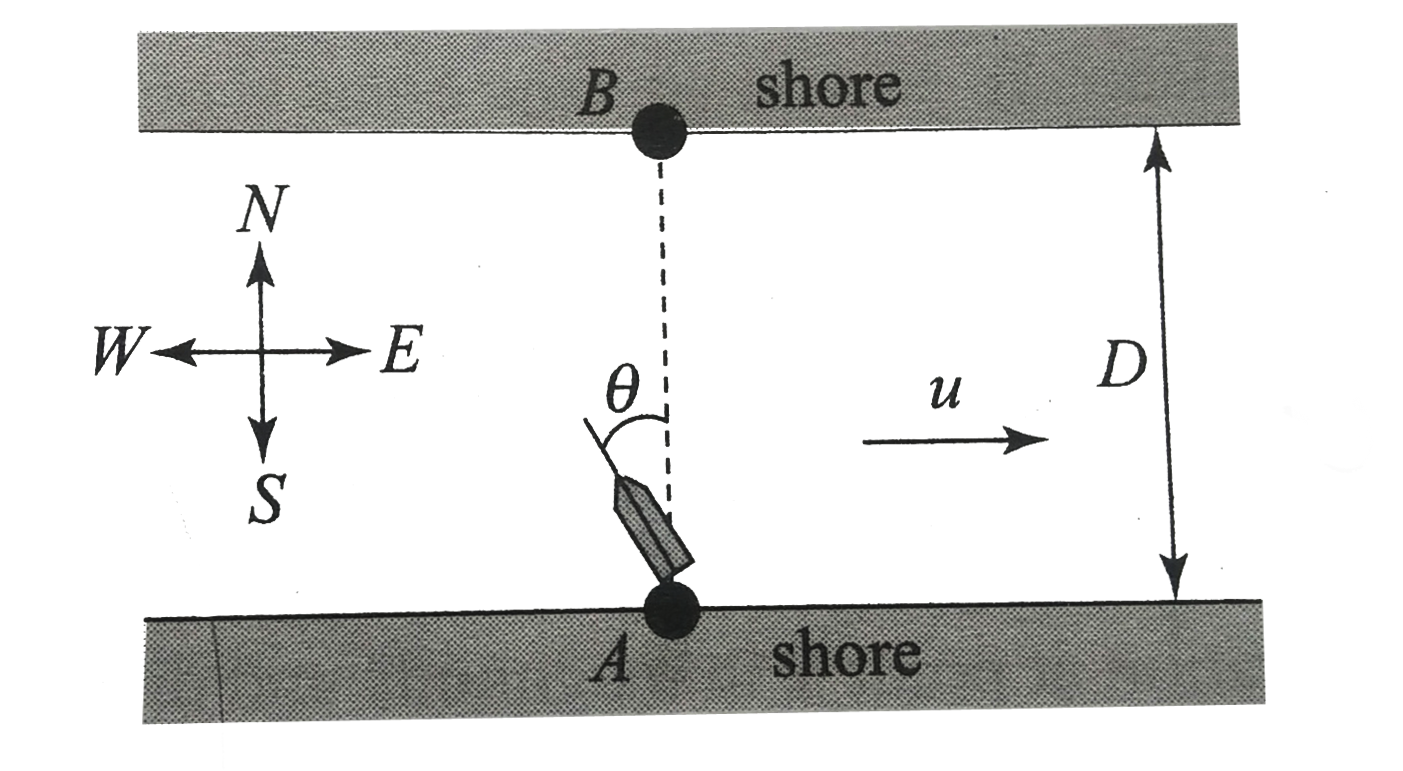
Two ports, A and B, on a north-south line ar separated by a river of width D. The river flows east with speed u. A boat crosses the river starting from port A. The speed of the boat relative to the river is v. Assume v=2u
Q. What is the direction of the velocity of boat relative to river θ, sothat it crosses directly on a line from A to B?A30∘ west of northB30∘ east of northC60∘ west of northD60∘ east of northA river is flowing from north to south at a speed of 0.3 kph. A man on the west bank of the river, capable of swimming 1 kph in still water, wants to swim across the river in the shortest time. He should in a direction:
ADue eastB30∘ north of eastC30∘ weat of northD60∘ north of east
A boat which has a speed of 6km/h in still water crosses a river of width 1 km along the shortest possible path in 20 min. the velocity of the river water in km/h is
View SolutionThe speed of boat is 5kmh−1 in still water. It crosses a river of width 1 km along the shortest possible path in 15 min. Then, velocity of river will be
View SolutionThe speed of boat is 5kmh−1 in still water. It crosses a river of width 1 km along the shortest possible path in 15 min. Then, velocity of river will be
View SolutionA boat crosses a river of width 1 km by shortest path in 15 min. if the speed of boat in still water is 5 kmh−1, then what is the speed of the river?
View SolutionA man can swim with a speed v relative to water . There is a river of width d which is flowing at a speed u . A is the point on river bank from where he starts and B is point directly opposite to A on the other side of the river .
View Solution
