In the climax of a movie, the hero jumps from a helicopter and the villain chasing the hero also jumps from the same level . After sometime when they come at same horizontal level, the villain fires bullet horizontally towards the hero, Both were falling with constant acceleration 2(m)/(s^2), because of parachute, Assuming the hero to be within the range of bullet, which of the following is correct.
bullet will hit the hero
bullet will pass above the hero
bullet will pass below the hero
bullet will definitely hit the hero, if both were falling with constant acceleration g instead of 2ms2
The correct Answer is:C
To solve the problem, we need to analyze the motion of both the hero and the villain, as well as the bullet fired by the villain.
1. Understanding the Scenario:
- Both the hero and the villain jump from the same height and fall under the influence of gravity with a downward acceleration of 2m/s2 due to their parachutes.
- The villain fires a bullet horizontally when he reaches the same horizontal level as the hero.
2. Vertical Motion of the Hero and Villain:
- The vertical motion of both the hero and the villain can be described by the equation of motion:
h=12at2
where h is the distance fallen, a=2m/s2, and t is the time of fall.
3. Vertical Motion of the Bullet:
- The bullet, once fired, will also experience a downward acceleration due to gravity, which is approximately 9.8m/s2.
- The vertical motion of the bullet can also be described by the same equation of motion:
hb=12gt2b
where g≈9.8m/s2 and tb is the time taken for the bullet to fall.
4. Comparing the Vertical Distances:
- At the moment the villain fires the bullet, both the hero and the villain have fallen the same vertical distance h after time t.
- The bullet will start falling from the same height as the hero but will accelerate downwards faster due to the greater gravitational acceleration.
5. Conclusion:
- Since the bullet falls faster than the hero (because 9.8m/s2>2m/s2), it will pass below the hero as both continue to fall.
- Therefore, the correct answer is that the bullet will pass below the hero.
Final Answer:
The bullet will pass below the hero.
- Both the hero and the villain jump from the same height and fall under the influence of gravity with a downward acceleration of 2m/s2 due to their parachutes.
- The villain fires a bullet horizontally when he reaches the same horizontal level as the hero.
- The vertical motion of both the hero and the villain can be described by the equation of motion:
h=12at2
where h is the distance fallen, a=2m/s2, and t is the time of fall.
- The bullet, once fired, will also experience a downward acceleration due to gravity, which is approximately 9.8m/s2.
- The vertical motion of the bullet can also be described by the same equation of motion:
hb=12gt2b
where g≈9.8m/s2 and tb is the time taken for the bullet to fall.
- At the moment the villain fires the bullet, both the hero and the villain have fallen the same vertical distance h after time t.
- The bullet will start falling from the same height as the hero but will accelerate downwards faster due to the greater gravitational acceleration.
- Since the bullet falls faster than the hero (because 9.8m/s2>2m/s2), it will pass below the hero as both continue to fall.
- Therefore, the correct answer is that the bullet will pass below the hero.
The bullet will pass below the hero.
Topper's Solved these Questions
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICSCHAPTER - CENGAGE PHYSICS DPPEXERCISE - Fill in the blanks type
26 Videos
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICSCHAPTER - CENGAGE PHYSICS DPPEXERCISE - Comprehension Type
31 Videos
- CENGAGE PHYSICS DPPBOOK - CENGAGE PHYSICSCHAPTER - CENGAGE PHYSICS DPPEXERCISE - Multiple Correct Answer Type
54 Videos
- CAPACITOR AND CAPACITANCEBOOK - CENGAGE PHYSICSCHAPTER - CAPACITOR AND CAPACITANCEEXERCISE - Integer
5 Videos
- CENTRE OF MASS CONVERSATION OF MOMENTUM AND COLLISIONBOOK - CENGAGE PHYSICSCHAPTER - CENTRE OF MASS CONVERSATION OF MOMENTUM AND COLLISIONEXERCISE - Question Bank
38 Videos
Similar Questions
A mass M=1 kg lies on a smooth horizontal base of a rough inclined plane at an angle 37∘ with the horizontal as shown in figure-4.81. A bullet of mass m=0.1 kg is fired horizontally with a velocity u=110m/s and gets embeded in it almost immediately. The impluse imparted carries the combined mass up the incline and finally lands on the horizontal level with the horizontal base.
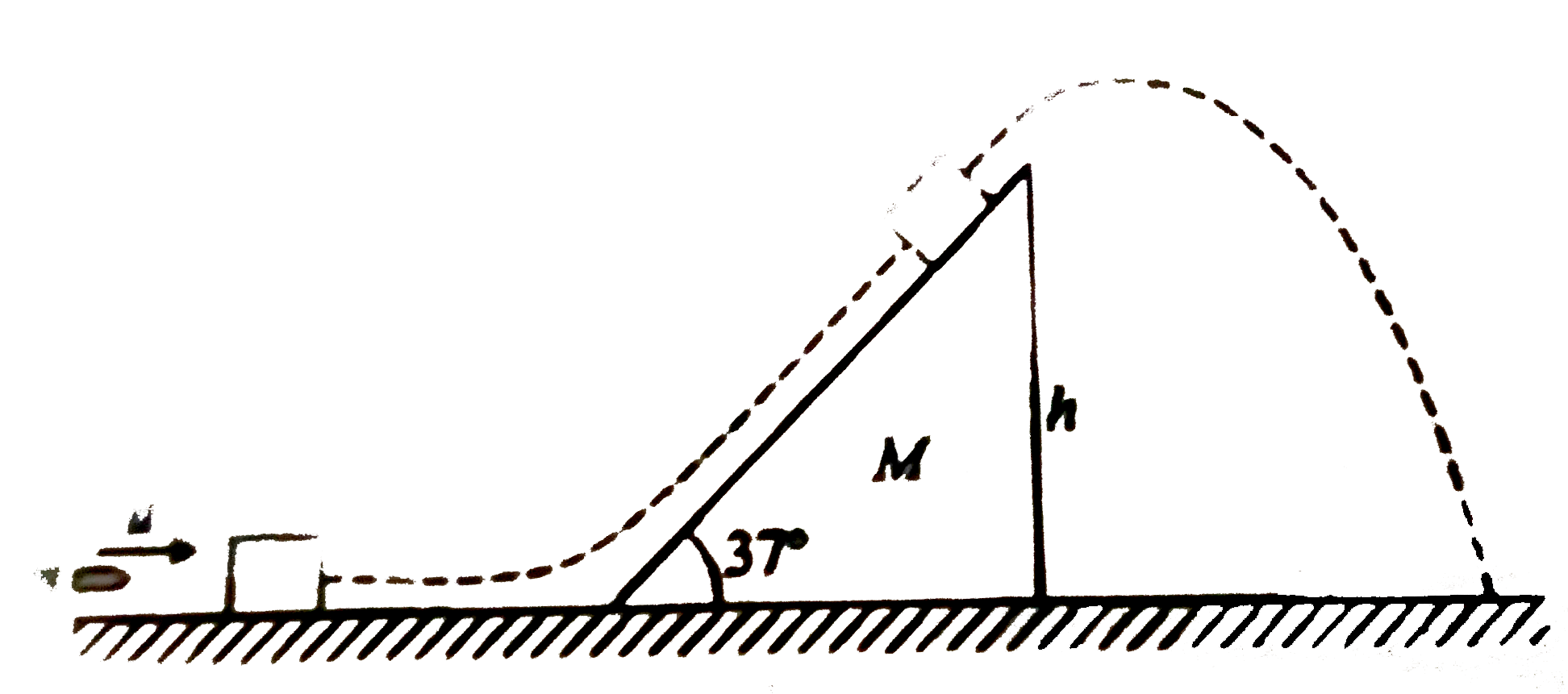
If the length of the incline is I=1.8 m and the incline offers a coefficient of kinetic friction 0.5 to the sliding of the mass, find the horizontal distance from the base of the incline to the point of landing of the combined mass. Assume the contact of the incline with the horizontal plane is smooth and mass is not jerked when starts up on incline plane.
View SolutionTwo particles were projected one by one with the same initial velocity from the same point on level ground. They follow the same parabolic trajectory and are found to be in the same horizontal level, separated by a distance of 1m,2s after the second partice was projected. Assume that the horizontal component of their velocities is 0.5ms−1. Find
(a) the horizontal range of the parabolic path.
(b) the maximum height for the parabolic path.
View Solution
Knowledge Check
A boy and a block, both of same mass, are suspended at the same horizontal level, from each end of a light string that moves over a frictionless pulley as shown. The boy start moving upward with an acceleration 2.5m/s2 relative the rope. If the block is to travel a total distance 10m before reaching at the pulley, the time taken by the block in doing so is equal to:
 A√8sB4sC10√8sD8s
A√8sB4sC10√8sD8sTwo particles, one of mass m and the other of mass 2m, are projected horizontally towards each other from the same level above the ground with velocities 10 m/s and 5 m/s, respectively. They collide in air and stick to each other. The distance of the combined mass from point A is
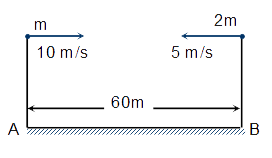 A40 mB20 mC30 mDzero
A40 mB20 mC30 mDzeroA man is standing on a road and observes that rain is failing at angle 45∘ with the vertical. The man starts running on the road with constant acceleration 0.5m/s2. After a certain time from the start of the motion, it appears to him that rain is still falling at angle 45∘ with the vertical, with speed 2√2m/s. Motion of the man is in the same vertical plane in which the rain is falling. Then which of the following staement(s) are true.
Ait is not possibleBSpeed of the rain relative to the ground is 2m/sCSpeed of the man when he finds rain to be falling at angle 45∘ with the vertical, is 4m/s.DThe man has travelled a distance 16cm on the road by the time he again finds rain to be falling at angle 45∘
A bullet fired from a gun with a velocity of 140ms−1 strikes the ground at the same level as the gun at a distance of 1 km. Find the angle of inclination with the horizontal at which the bullet is fixed. Take g=9.8ms−2.
View SolutionA particle is projected from point A at an angle of 53^(@) with horizontal.At the same time wedge starts from rest and moves with constant acceleration a as shown. The value of a for which the particle strikes the plane perpendicular to it at P is (tan53^(@)=4/3 g=10m/s^(2))
View SolutionTwo persons, A of mass 60kg and B of mass 40kg, are standing on a horizontal platform of mass 50kg. The platform is supported on wheels on a horizontal frictionless surface and is initially at rest. Consider the following situations.
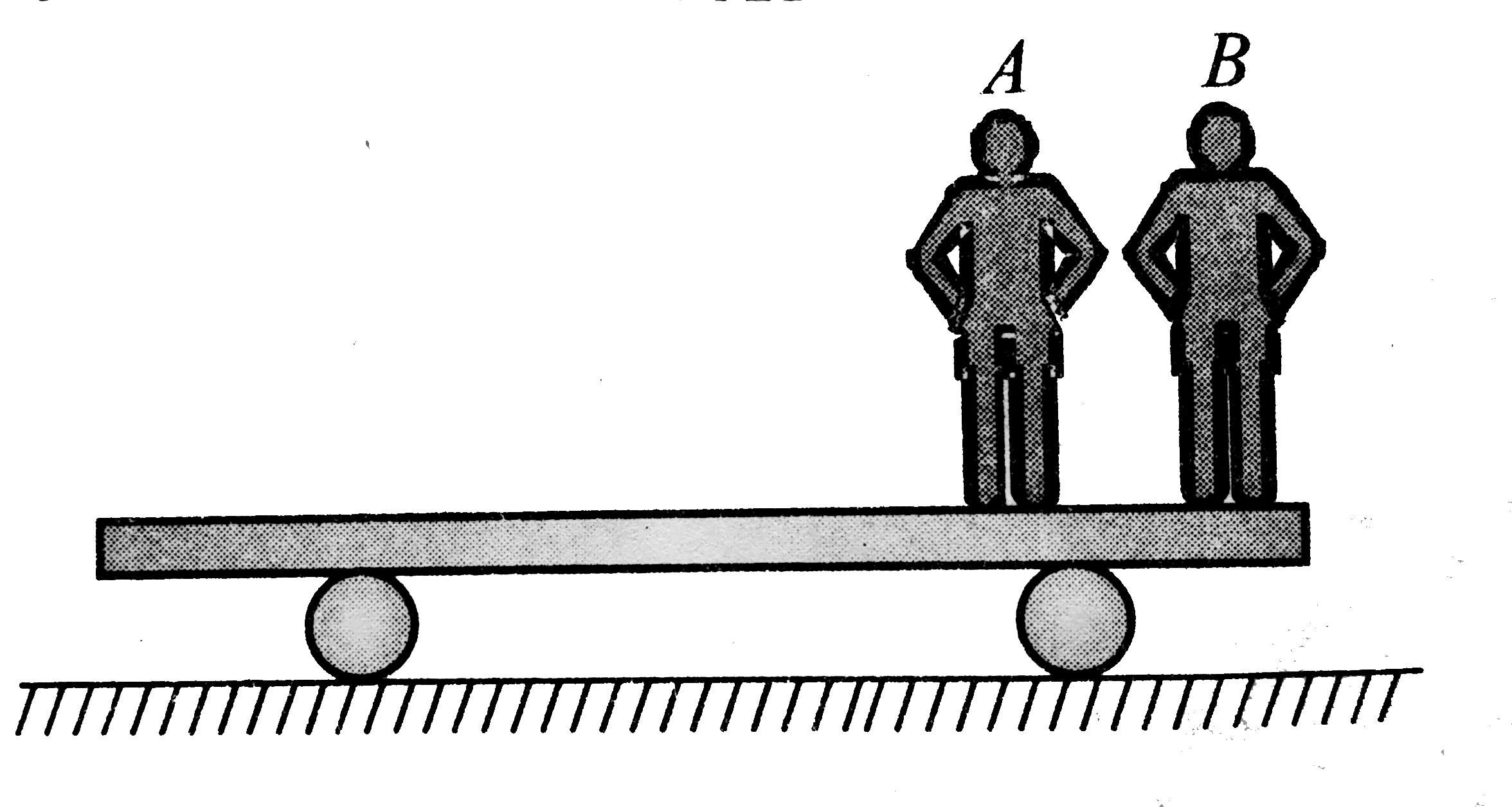
(i) Both A and B jump from the platform simultaneously and in the same horizontal direction.
(ii) A jumps first in a horizontal direction and after a few seconds B also jumps in the same direction. In both the situations above, just after the jump, the person (A or B) moves away from the platform with a speed of 3m/s relative to the platform and along the horizontal.
Final speed of the platform in situation (i), i.e., just after both A and B have jumped will be a.
View SolutionTwo persons, A of mass 60kg and B of mass 40kg, are standing on a horizontal platform of mass 50kg. The platform is supported on wheels on a horizontal frictionless surface and is initially at rest. Consider the following situations.
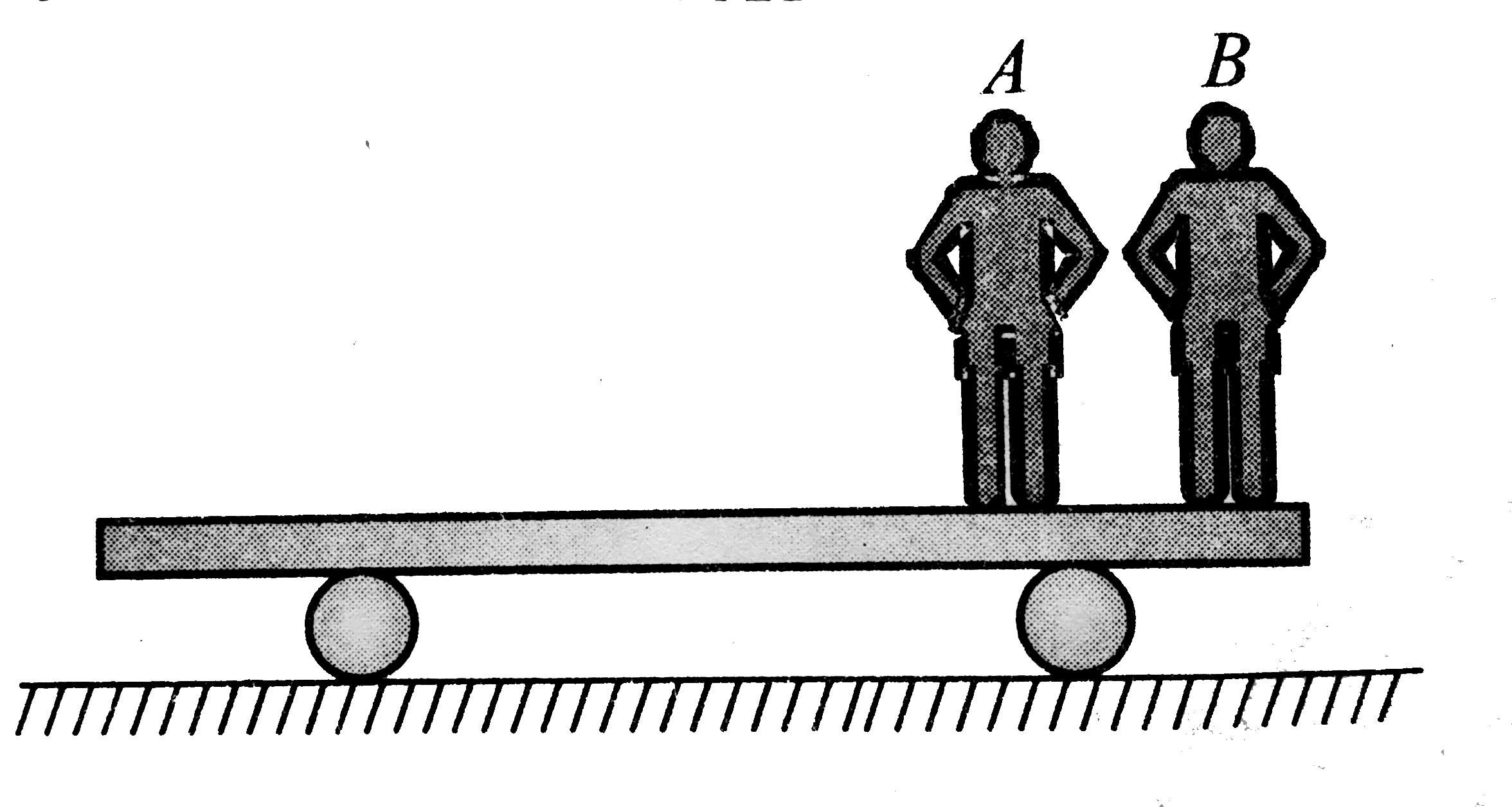
(i) Both A and B jump from the platform simultaneously and in the same horizontal direction.
(ii) A jumps first in a horizontal direction and after a few seconds B also jumps in the same direction. In both the situations above, just after the jump, the person (A or B) moves away from the platform with a speed of 3m/s relative to the platform and along the horizontal.
Final speed of the platform in situation (ii), i.e., just after B has jumped, will be nearly
View SolutionTwo persons, A of mass 60kg and B of mass 40kg, are standing on a horizontal platform of mass 50kg. The platform is supported on wheels on a horizontal frictionless surface and is initially at rest. Consider the following situations.
(i) Both A and B jump from the platform simultaneously and in the same horizontal direction.
(ii) A jumps first in a horizontal direction and after a few seconds B also jumps in the same direction. In both the situations above, just after the jump, the person (A or B) moves away from the platform with a speed of 3m/s relative to the platform and along the horizontal.
In situation (i), just after both A and B jump from the platform, velocity of centre of mass of the system (A,B and the platform) is
View Solution
