In U.C.M., when time interval δt→0 , the angle between change in velocity (δv) and linear velocity ( v) will be
0∘
90∘
180∘
45∘
The correct Answer is:B
The direction of change in velocity (δv) is given by
ϕ=180∘θ2=90∘−θ2 …….(i)
This can be shown garphically as

For small time interval, i.e, δt→0, then angle between V1 and V2 is very small i.e., θ=0∘
So , from Eq.(i) , we get
ϕ=90∘ .
Topper's Solved these Questions
Similar Questions
A particle is travelling on a curved path. In an interval Δt its speed changed from v to 2v. However, the change in magnitude of its velocity was found to be ∣∣∣−−→ΔV∣∣∣=√5v. What can you say about the direction of velocity at the beginning and at the end of the interval (Δt)?
View SolutionA certain constant force starts acting on a body moving at a constant velocity v. After a time interval Δt, the velocity of the body is reduced by half, and after the same time interval, the velocity is again reduced by half. Determine the velocity vf of the body after a time interval 3Δt from the moment when the constant force starts acting.
View Solution
Knowledge Check
Relation between Linear velocity (v) and Angular velocity (ω)
Av=rωBω=vωCr=vωDv=ωrΔV measured between B and C is
AρIπα−ρIπ(α−b)BρIα−ρI(α+b)CρI2πα−ρI2π(α−b)DρI2π(α−b)Delta V measured between B and C is
AρIπα−ρIπ(α+b)BρIπα−ρI(α+b)CρI2πα−ρI2π(α+b)DρI2π(alpah−b)
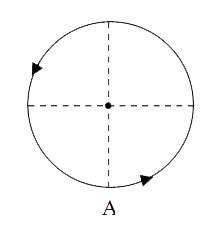
A particle is describing uniform circular motion in the anti-clockwise sense such that its time period of revolution is T. At t = 0 the particle is observed to be at A. If θ1 be the angle
between acceleration vector at t=T4 and average velocity vector in the time interval 0 to T/4 and θ2 be the angle between acceleration vector at t=T4 and the vector representing change in velocity in the time interval 0 to T/4, then :
View SolutionAt the start of a motion along a line the initial velocity is u and acceleration is at. The final velocity v after time t, is
View SolutionConsider a particle moving along x-axis. Its distance from origin O is described by the co-ordinate x which varies with time. At a time t1, the particle is at point P, where its co-ordinate is x1 and at time t2, the particle is at point Q, where its co-ordinate is x2. The displacement during the time interval from t1 to t2 is the vector from P to Q, the x-component of this vector is (x2−x1) and all other components are zero.
It is convenient to represent the quantity x2−x1 the change in x by means of a notation Δ, thus Δx=x2−x1 and Δt=t2−t1.
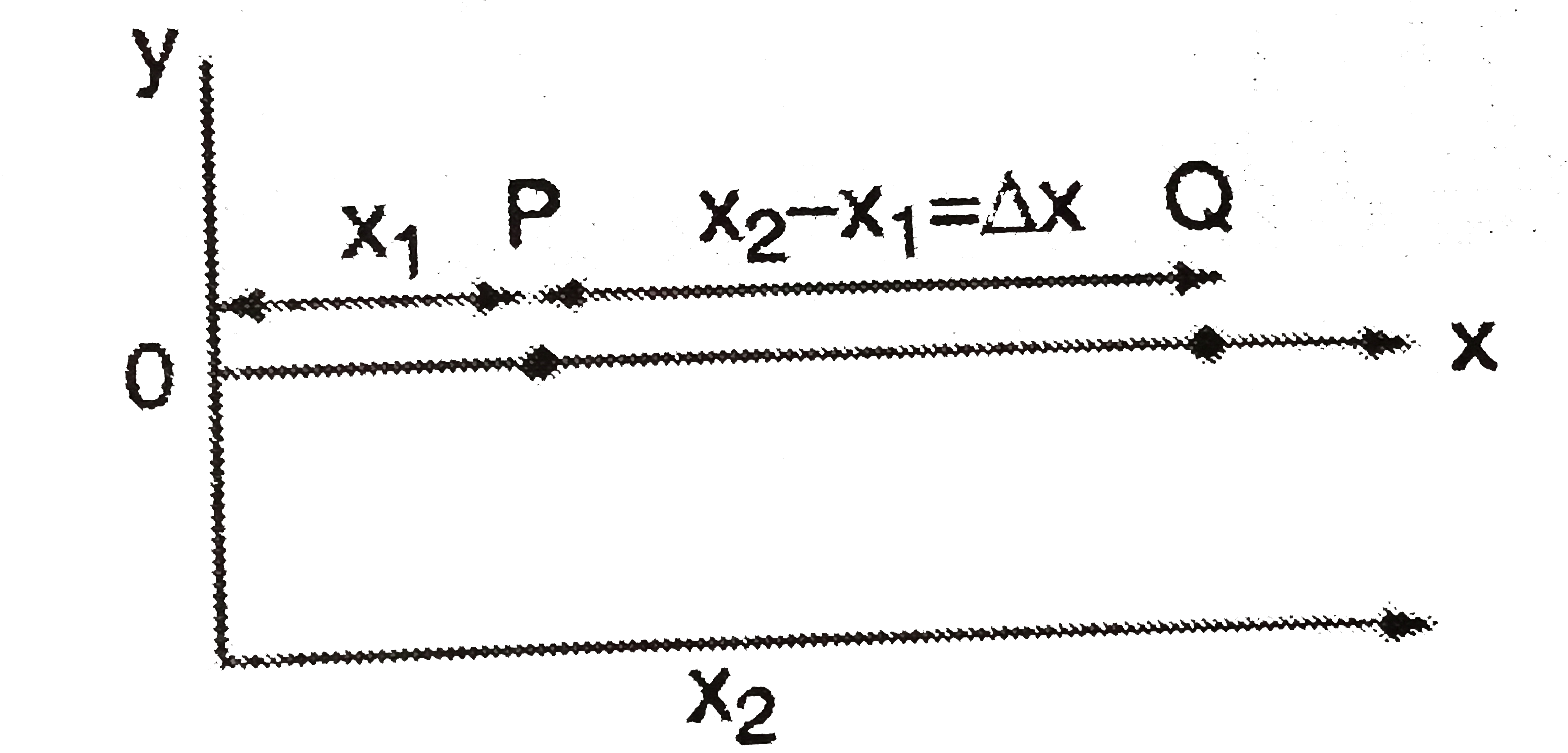
The average velocity ¯¯¯V=x2−x1t2−t1=ΔxΔt
The resistive force suffered by a motor boat is proportional to v2, where v is instantaneous velocity. The engine was shut down when the velocity of the boat was v0. Find the average velocity at any time t.
View SolutionAn electron is launched with velocity →v in a uniform magnetic field →B. The angle θ between →vand→B lies between 0 and π2. Its velocity vector →v returns to its initial value in a time interval of
View SolutionThe distribution of the molecular velocities of gas molecules at any temperature T is shown below. (The plot below is known as Maxwell's distribution of molecular speeds.)

where
v is molecular velocity
n is number of molecules having velocity v
Let us define ΔNv, which is equal to the number of molecules between the velocity range v and v+Δv, given by
ΔNv=4πNa3e−bv2v2Δv
where
N is total number of molecules
a=√M02πRT and b=M02RT
R is universal gas constant
T is temperature of the gas
M0 is molecular weight of the gas
Answer the following question:
If PPc=Pr, TTc=Tr, and VmVm,c=Vr where
View Solution
