The equiconvex lens has focal length f. If is cut perpendicular to the principal axis passin through optical centre, then focal length of each half is
f2
2f
3f2
f
The correct Answer is:B
As, for equiconvex lens R1=R2=R ( say )
So, 1f=(μreal −1)2R … ( i)
Now, if lens is cut along the line perpendicular to the principal axis as shown in the figure,

The new cut part of the lens has, R1=RandR2=∞
Again by using the lens Maker's formula, focal length of the new part of the lens,
1f'=(μreal−1)[1R−(−1∞)]
⇒1f=(μreal −1)[1R] ...(ii)
So, from the Eqs. (i ) and (ii) , we get
f'=2f
Topper's Solved these Questions
Similar Questions
Knowledge Check
If a equiconvex lens of focal length f is cut into two halves by a plane perpendicular to the principal axis, then
AThe focal length of each half becomesf2BThe focal length of each half becomes 2fCThe focal length of each half remains fDThe focal length of each half becomes f4If a symmetrical convex lens of focal length 'f is cut into two parts along the principal axis as shown in the figure, the focal length of each part will be:
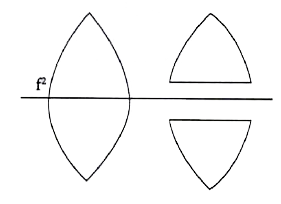 Af2Bf4CfD∞
Af2Bf4CfD∞
The focal length of the convex lens isA+10 cmB+20cmC−10cmD−20cm
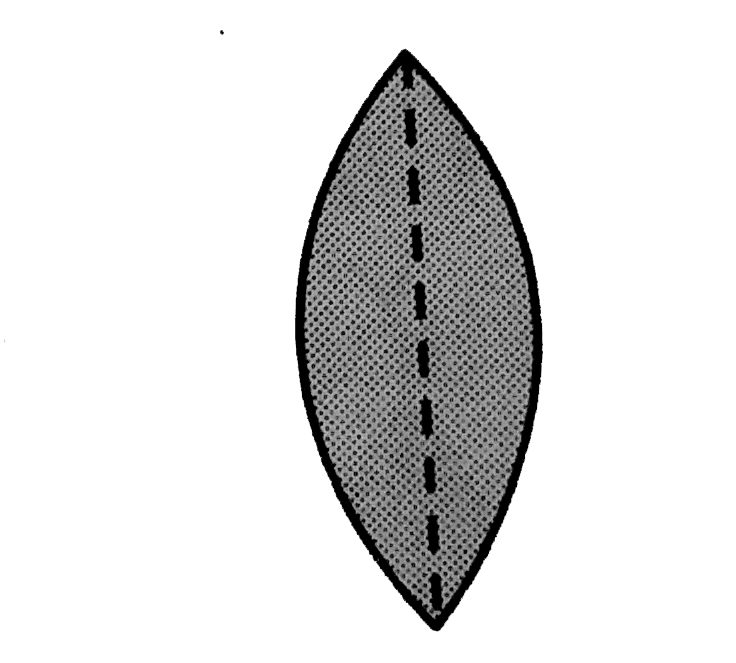
A convex lens has a focal length f. It is cut into two parts along the dotted line as shown in figure. The focal length of each part will be
View SolutionAn equiconvex lens of glass of focal length 0.1 metre is cut along a plane perpendicular to principle axis into two equal parts. The ratio of focal length of new lenses formed is:
View SolutionAn equiconvex lens of glass of focal length 0.1 meter is cut along a plane perpendicular to principle axis into two equal parts. The ratio of focal length of new lenses forms is
View SolutionA convex lens has a focal length f. It is cut into two parts along the dotted line as shown in figure. The focal length of each part will be

View SolutionA symmetrical biconvex lens of focal length f is cut into four identical pieces along its principal axis and to the perpendicular to principal axis. The focal length of one of four pieces is
View Solution
