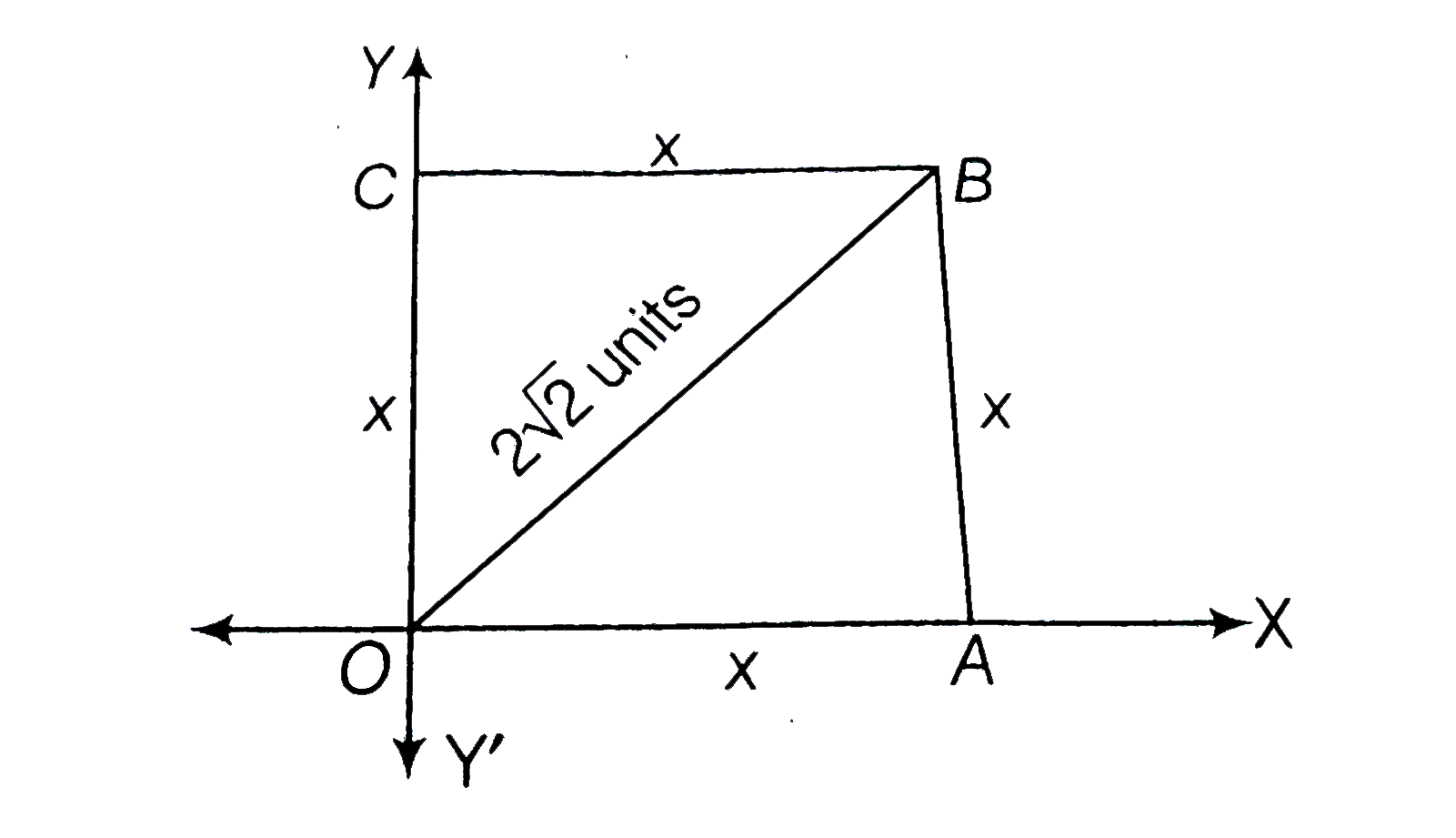
Write down 2×2 matrix A which corresponds to a counterclockwise rotation of 60∘ about tha origin. In the diagram OB of 2√2 units in lenth. The square is rotated counterclockwise about O through 60∘ find the coordiates of the vertices of the square after rotating.
the matrix desribes a rotation through an angle 60∘ in counterclockwise direction is
[cos60∘,−sin60∘sin60∘,cos60∘]=⎡⎢⎣12−√32−√3212⎤⎥⎦=12[1−√3√31]
Since, each side of the square be x,
then x2+x2=(2√2)2
⇒ 2x2=8⇒x2=4
∴ x=2units
therefore the coordinates of the vertices O,A,B and C are (0,0),(2,0),
(2,2)and(0,2), respectively. Let after rotation A map into A,B map into B,C map into C but the O map into itself.
If coordinates of A'B' and C' are (x',y),(x'',y'')and(x''',y'''), respectively.
∴ [x'y']=12[1−√3√31][20]=12[22√3]=[1√3]
∴ x'=1,y'=√3⇒A(2,0)→A'(1,√3)
and [x''y'']=12[1−√3√31][22]=12[2−2√32√3+2]=[1−√3√3+1]
∴ x''=1−√3,y''=√3+1
⇒ B(2,2)→B'(1−√3,√3+1)
[x''y'']=12[1−√3√31][02]=12[−2√32]=[−√31]
∴ x'''=−√3,y'''=1
⇒ C(0,2)→C'(−√3,1)
Similar Questions
Knowledge Check
The complex number z = 1 + i is rotated through an angle 3π/2 anticlockwise direction about the origin and stretched by additional √2 units, then the new complex number is
A2 (1 + i)B2 (1 - i)C√2(1−i)D−√2(1+i)If the pair of straight lines ax2+2hxy+by2=0 is rotated about the origin through 90∘, then their equations in the new position are givne by
Aax2−2hxy+by2=0Bax2−2hxy−by2=0Cbx2−2hxy+ay2=0Dbx2+2hxy+ay2=0the matrix S is rotation through an angle 45∘ and G is th reflection about the line y=2x, then (SG)2 is equal to
A7IB5IC3IDI
A line through the point A(2,0) which makes an angle of 30∘ with the positive direction of x−axis is rotated about A in anticlockwise direction through an angle 15∘. Find the equation of the straight line in the new position.
View SolutionThe point represented by the complex number 2−i is rotated about origin through on angle π2 the clockwise direction, the new position of the point is
View SolutionA line through the point A(2,0) which makes an angle of 300 with the positive direction of x-axis is rotated about A in clockwise direction through an angle 150. Find the equation of the straight line in the new position.
View Solutionif the axes are rotated through 60 in the anticlockwise sense,find the transformed form of the equation x2−y2=a2,
View SolutionThe complex number z=1+i is rotated through an angle 3π2 in anti-clockwise direction about the origin and stretched by additional √2 unit, then the new complex number is:
View Solution