Question

The area of the plane region bounded by the curves $x+2y^2=0$
and $x+3y^2=1$
is equal to
(1) $\frac{5}{3}$

(2) $\frac{1}{3}$

(3) $\frac{2}{3}$

(4) $\frac{4}{3}$

Options

A. `(4)/(3)` sq units
B. `(5)/(3)` sq unit
C. `(1)/(3)` sq unit
D. `(2)/(3)` sq unit

Explanation

On solving the given curves, we get
`y=pm1 and x=-2`
`therefore` Intersection points are (-2,1) and (-2,-1)
`therefore` Required area `=|int_(-1)^(1)(1-3y^(2)-2y^(2))dy|`

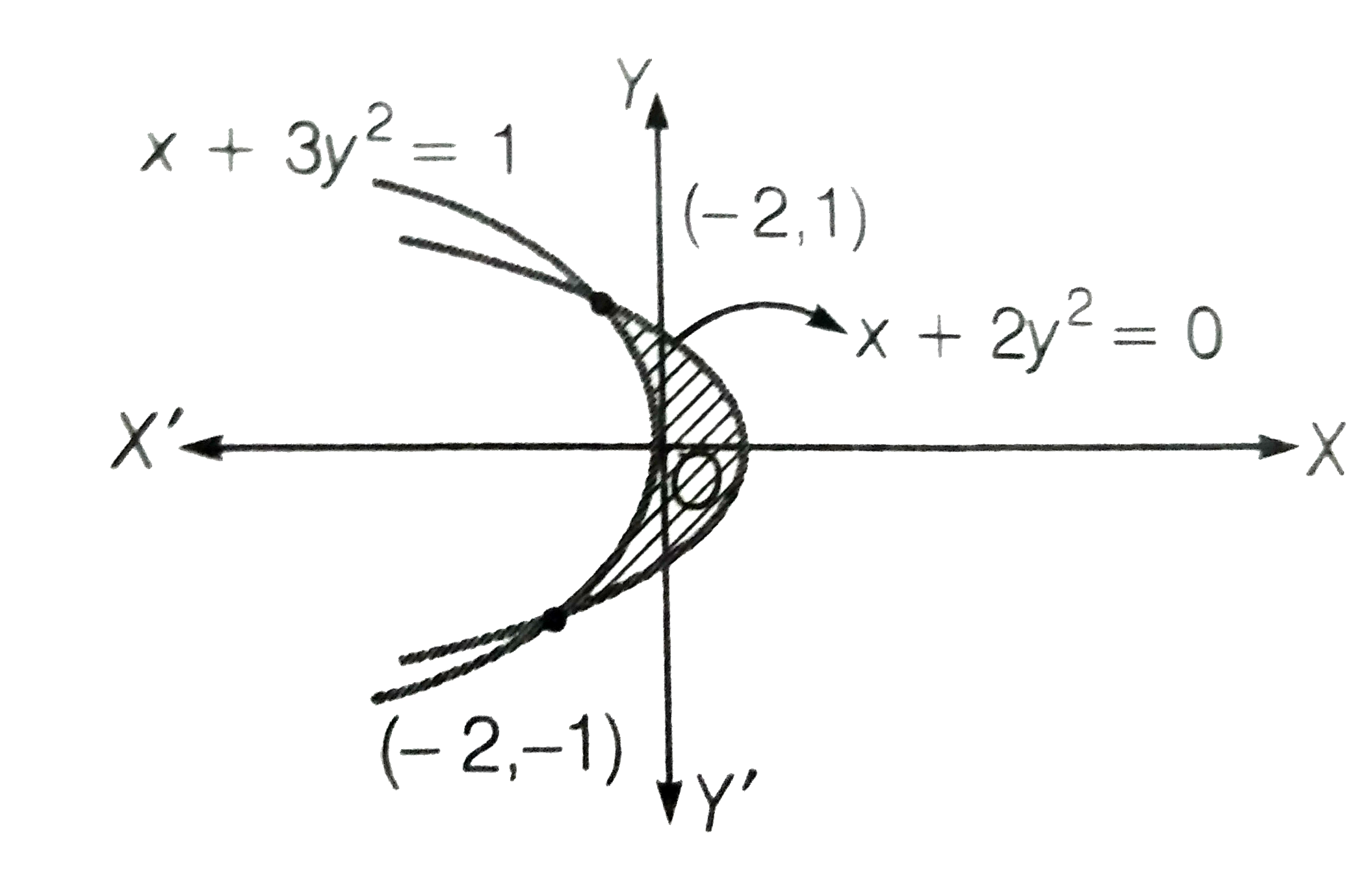
`=|2int_(0)^(1)(1-y^(2))dy|=|2[y-(y^(3))/(3)]_(0)^(1)|=(4)/(3)` sq units