Question

Five sinusoidal waves have the same frequency $500Hz$ but their amplitudes are in the ratio $2:1/2:1/2:1:1$ and their phase angles $0,\pi /6,\pi /3,\pi /2\text{and}\pi$, respectively . The phase angle of resultant wave obtained by the superposition of these five waves is

Options

A. `30^(@)`
B. `45^(@)`
C. `60^(@)`
D. `90^(@)`

Answer

B

Explanation

`y _(1) = 2 A sin omega t`
`y_(2) = (A)/(2) sin ( omega t + (pi)/(6))`
`y_(3) = (A)/(2) sin ( omega t + (pi)/( 3))`
`y _(4) = A sin ( omega t (pi)/(2))`
`y_(5) = A sin ( omega t + pi)`
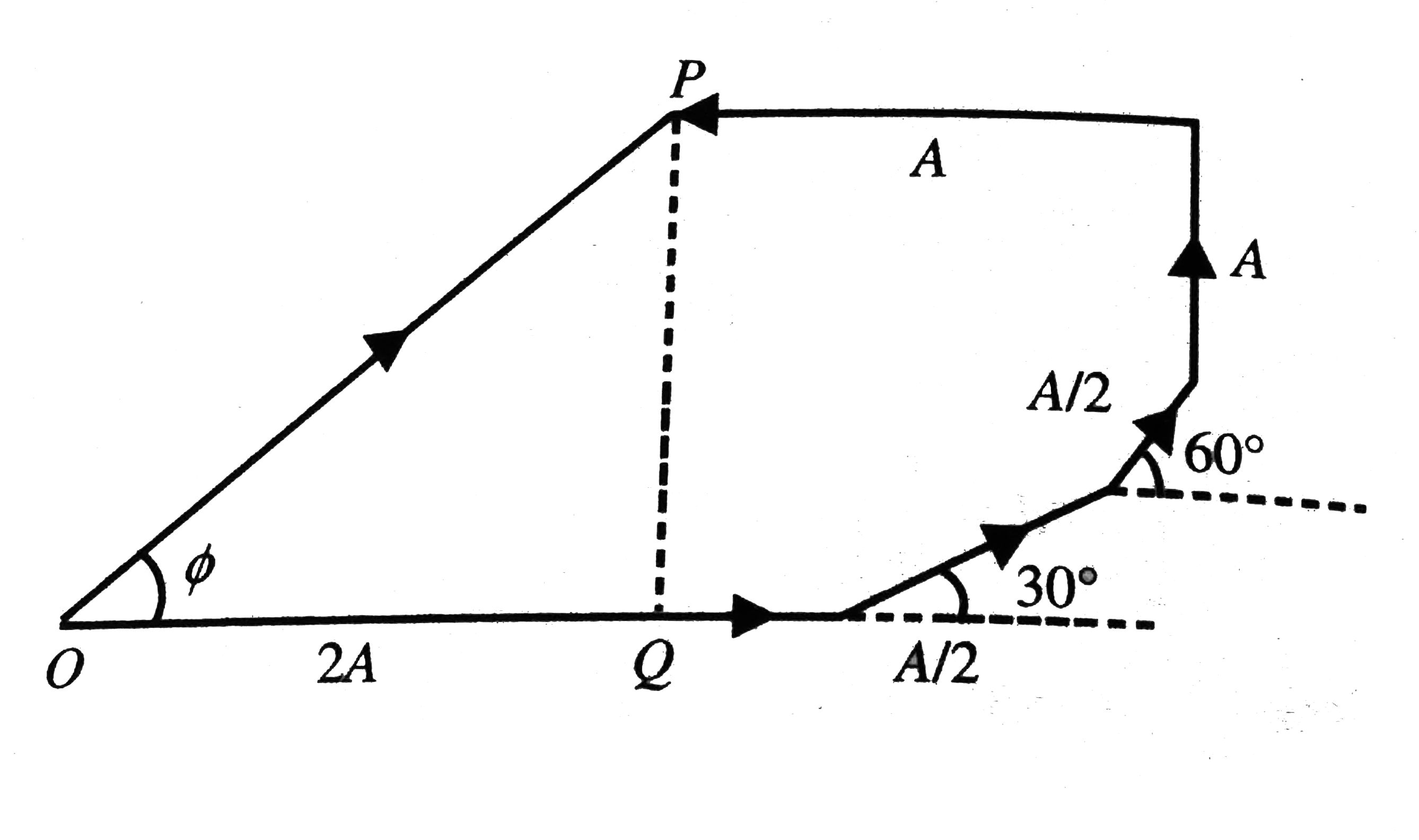
By phaser diagram ,
`tan phi = (PQ)/(OQ) = 1`
`phi = 45^(@)`
Alternatively : ` y = 2 A sin omega t + (A)/(2) ( sin omega cos 30^(@) + cos omega t sin 30^(@)) + (A)/(2) ( sin omega t cos 60^(@) + cos omega t sin 60^(@)) + A cos omega t - A sin omega t`
` = A' cos phi sin omega t + A' sin phi cos omega t`
where `A' cos phi = [ A + (A)/(4) ( sqrt (3) + 1) ]`
where `A' sin phi = [ A + (A)/(4) ( sqrt (3) + 1) ]`
`tan phi = 1`
`phi = 45 ^(@)`