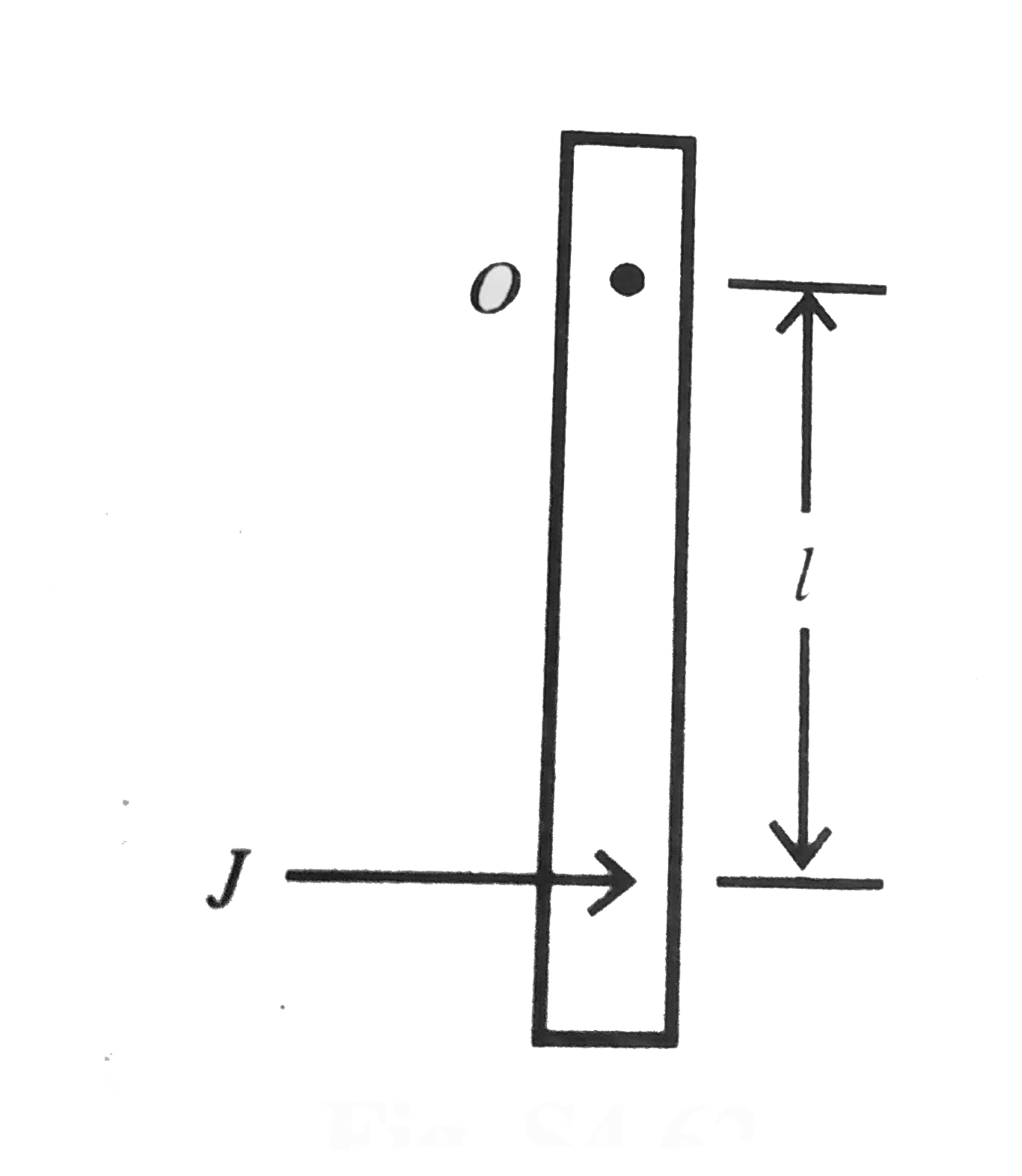
A block of mass m is suspended from one end of a light spring as shown. The origin O is considered at distance equal to natural length of the spring from the ceiling and vertical downwards direction as positive y-axis. When the system is in equilibrium a bullet of mass $\frac{m}{3}$ moving in vertical up wards direction with velocity $v_{0}$ strikes the block and embeds into it. As a result, the block (with bullet embedded into it) moves up and start oscillating. Based on the given information, answer the following question:
Q. The time taken by the block bullet system to move from $y = \frac{m g}{k}$ (initial equilibrium position) to $y = 0$ (natural length of spring) is (A represents the amplitude of motion)

$\sqrt{\frac{4 m}{3 k}} [{cos}^{- 1} (\frac{m g}{3 k A}) - {cos}^{- 1} (\frac{4 m g}{3 k A})]$

$\sqrt{\frac{3 k}{4 m}} [{cos}^{- 1} (\frac{m g}{3 k A}) - {cos}^{- 1} (\frac{4 m g}{3 k A})]$

$\sqrt{\frac{4 m}{6 k}} [{sin}^{- 1} (\frac{4 m g}{3 k A}) - {sin}^{- 1} (\frac{m g}{3 k A})]$

none of these

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

Initially in equilibrium let the elongation in spring be $y_{0}$ then $m g = k y_{0}$
$y_{0} = \frac{m g}{k}$ As the bullet strikes the block with velocity with velocity $v_{0}$ and gets embed ded into it, the velocity of the combined mass can be computed by ising the principle of moment conservation.
$\frac{m}{3} v_{0} = \frac{4 m}{3} v \Rightarrow v = \frac{v_{0}}{4}$
Let new mean position is at distance y from the origin, then
$k y = \frac{4 m}{3} g \Rightarrow y = \frac{4 m g}{3 k}$
Now, the block executes SHM about mean position defined by $y = \frac{4 m g}{3 k}$ with time period $T = 2 π \sqrt{\frac{4 m}{3 k}}$ . At $t = 0$ , the combined mass is at a displacement of $(y - y_{0})$ from mean position and is moving with velocity v, then by using $v = ω \sqrt{A^{2} - x^{2}}$ , we can find the amplitude of motion,
${(\frac{v_{0}}{4})}^{2} = \frac{3 k}{4 m} [A^{2} - {(y - y_{0})}_{0}^{2}] = \frac{3 k}{4 m} [A^{2} - {(\frac{m g}{3 k})}^{2}]$
$\Rightarrow A = \sqrt{\frac{m v_{0}^{2}}{12 k} + {(\frac{m g}{3 k})}^{2}}$
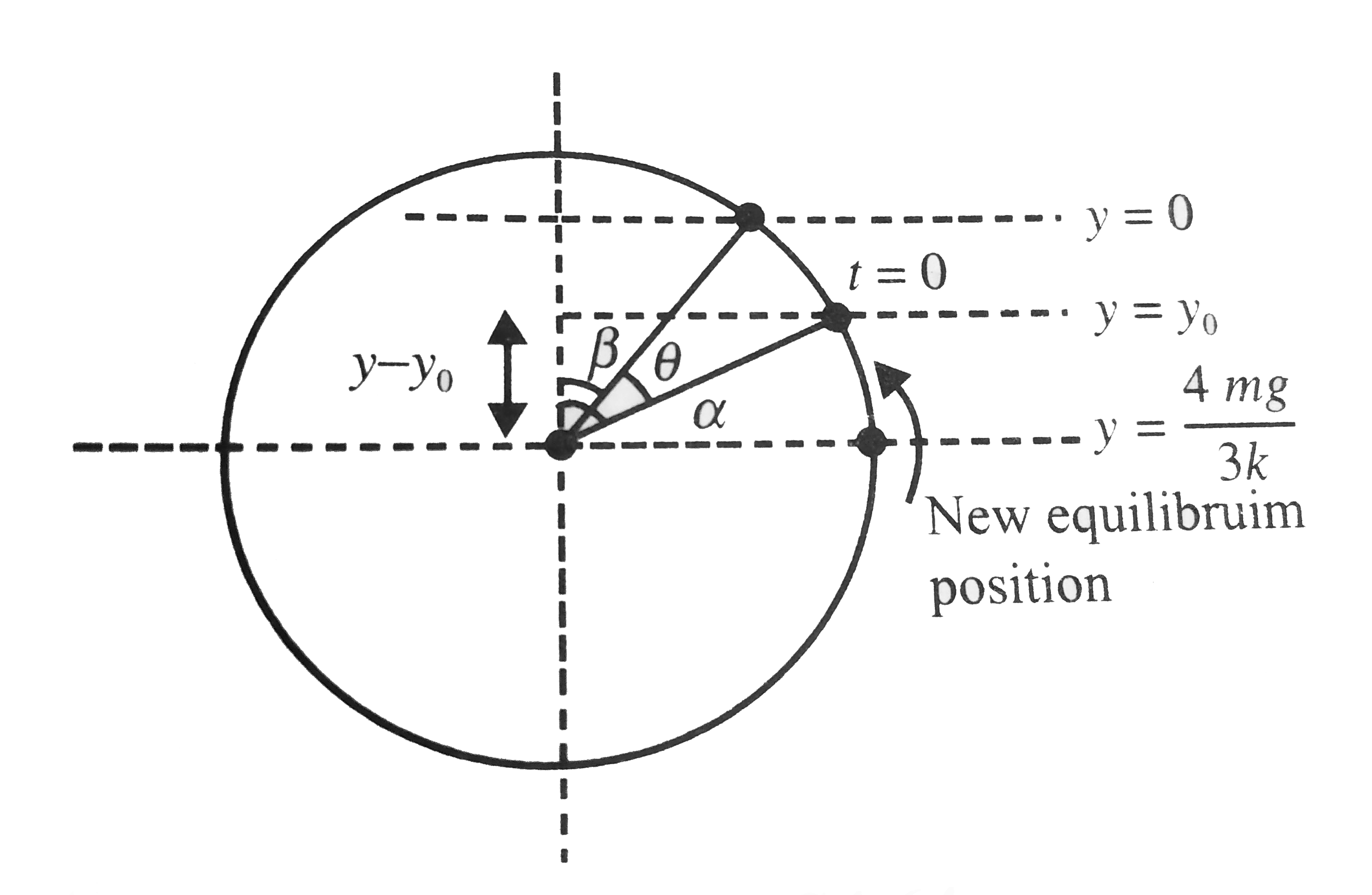
To compute the time taken by the combined mass from $y = \frac{m g}{k}$ to $y = 0$ , we can either go for equation method of circular motion projection method.
Required time, $t = \frac{θ}{ω} = \frac{α - β}{ω}$
$cos α = \frac{y - y_{0}}{A} = ⎛ ⎜ ⎝ \frac{\frac{4 m g}{3 k} - \frac{4 m g}{k}}{A} ⎞ ⎟ ⎠ = \frac{m g}{3 k A}$
$cos β = \frac{y}{A} = \frac{4 m g}{3 k A}$
So, $t = \frac{{cos}^{- 1} (\frac{m g}{3 k A}) - {cos}^{- 1} (\frac{4 m g}{3 k A})}{ω}$
$= \sqrt{\frac{4 m}{3 k}} [{cos}^{- 1} (\frac{m g}{3 k A}) - {cos}^{- 1} (\frac{4 m g}{3 k A})]$

Updated on:21/07/2023

Class 11 PHYSICS SIMPLE HARMONIC MOTION

Topper's Solved these Questions

Knowledge Check

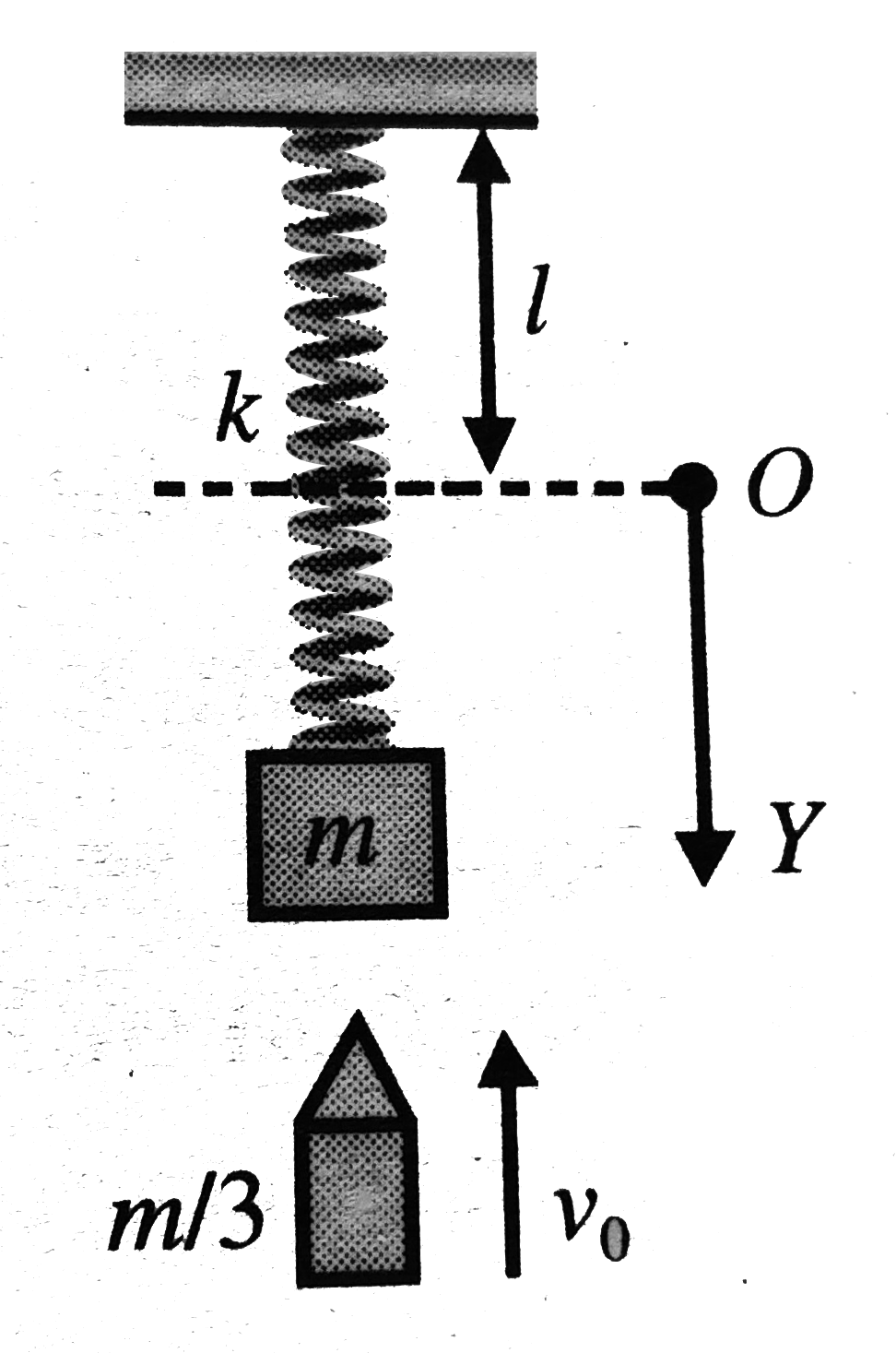
A block of mass m is suspended from one end of a light spring as shown. The origin O is considered at distance equal to natural length of the spring from the ceiling and vertical downwards direction as positive y-axis. When the system is in equilibrium a bullet of mass $\frac{m}{3}$ moving in vertical up wards direction with velocity $v_{0}$ strikes the block and embeds into it. As a result, the block (with bullet embedded into it) moves up and start oscillating. Based on the given information, answer the following question:
Q. The amplitude of oscillation would be
A $\sqrt{{(\frac{4 m g}{3 k})}^{2} + \frac{m v_{0}^{2}}{12 k}}$
B $\sqrt{\frac{m v_{0}^{2}}{12 k} + {(\frac{m g}{3 k})}^{2}}$
C $\sqrt{\frac{m v_{0}^{2}}{6 k} + {(\frac{m g}{k})}^{2}}$
D $\sqrt{\frac{m v_{0}^{2}}{6 k} + {(\frac{4 m g}{3 k})}^{2}}$
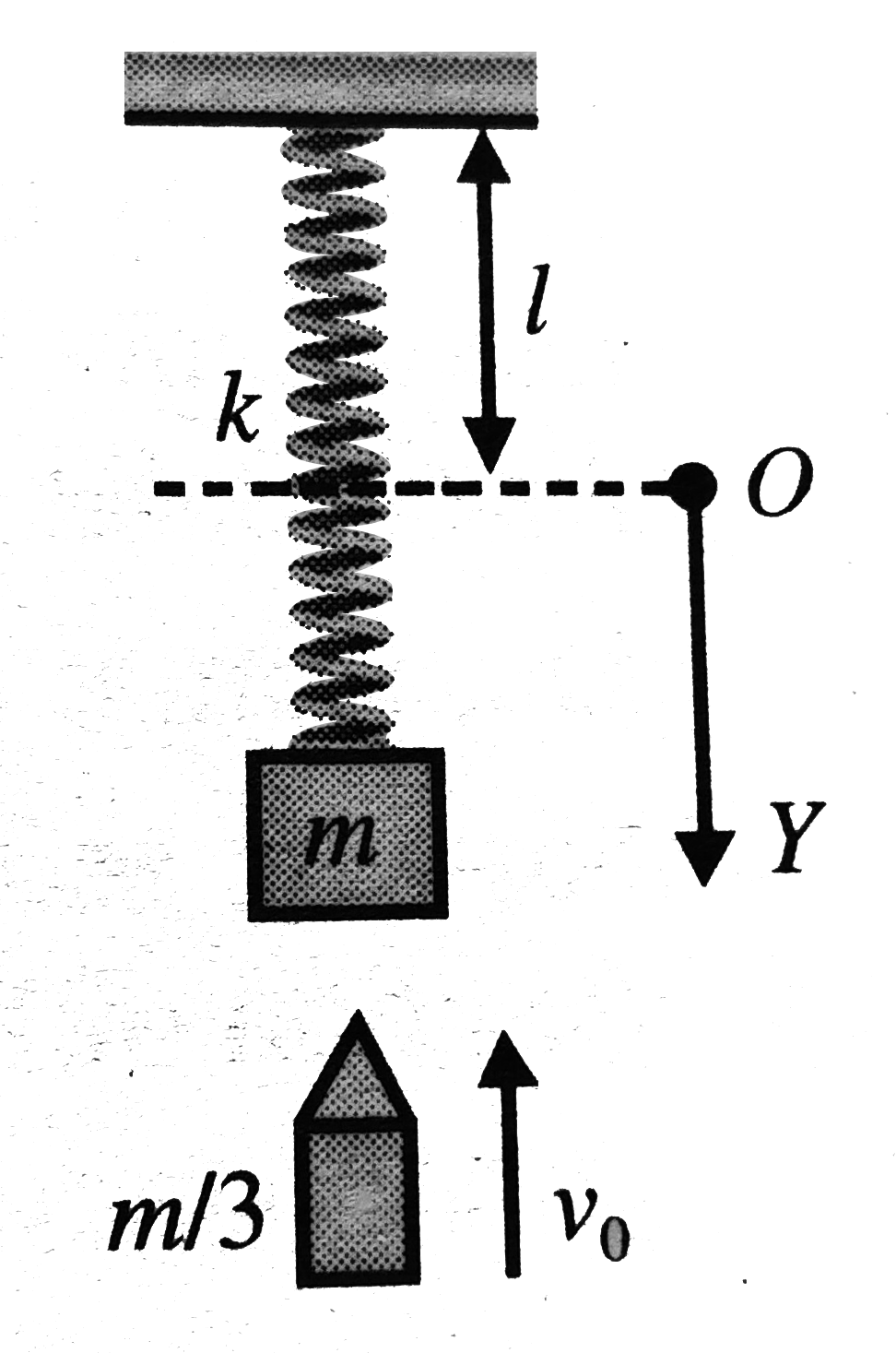
A block of mass m is suspended from one end of a light spring as shown. The origin O is considered at distance equal to natural length of the spring from the ceiling and vertical downwards direction as positive y-axis. When the system is in equilibrium a bullet of mass $\frac{m}{3}$ moving in vertical up wards direction with velocity $v_{0}$ strikes the block and embeds into it. As a result, the block (with bullet embedded into it) moves up and start oscillating. Based on the given information, answer the following question:
Q. Mark out the correct statements (s).
Athe block bullet system performs SHM about $y = \frac{m g}{k}$ .
BThe block bullet system performs oscillatory motion but not SHM about $y = \frac{m g}{k}$ .
CThe block bullet system performs SHM about $y = \frac{4 m g}{3 k}$ .
DThe block bullet system performs oscillatory motion but not SHM about $y = \frac{4 m g}{3 k}$ .
A block of mass m is suspended by different springs of force constant shown in figure.

A $T_{1} = T_{2} = T_{4}$
B $T_{1} = T_{2} and T_{3} = T_{4}$
C $T_{1} = T_{2} = T_{3}$
D $T_{1} = T_{3}$ and $T_{2} = T_{4}$