The coefficient of apparent expansion of mercury in a glass vessel is and in a steel vessel is . If for steel is , then that of glass is
The correct Answer is:A
To solve the problem, we need to find the coefficient of linear expansion (α) for glass given the apparent expansion coefficients for mercury in glass and steel vessels, and the coefficient of linear expansion for steel.
1. Understanding the Apparent Expansion:
The apparent coefficient of expansion (γ) of a liquid in a vessel is given by the formula:
γ=γliquid−γvessel
where:
- γliquid is the volumetric expansion coefficient of the liquid.
- γvessel is the volumetric expansion coefficient of the vessel.
2. Given Values:
- Apparent expansion of mercury in glass: γapparent, glass=153×10−6°C−1
- Apparent expansion of mercury in steel: γapparent, steel=144×10−6°C−1
- Coefficient of volumetric expansion for steel: αsteel=12×10−6°C−1
3. Relating Volumetric Expansion to Linear Expansion:
The volumetric expansion coefficient (γ) is related to the linear expansion coefficient (α) by:
γ=3α
Thus, for steel:
γsteel=3αsteel=3×(12×10−6)=36×10−6°C−1
4. Setting Up the Equations:
For the glass vessel:
γmercury=γapparent, glass+γglass
For the steel vessel:
γmercury=γapparent, steel+γsteel
5. Equating the Two Expressions:
Since both expressions equal γmercury, we can set them equal to each other:
γapparent, glass+γglass=γapparent, steel+γsteel
6. Substituting Known Values:
Substitute the known values into the equation:
153×10−6+γglass=144×10−6+36×10−6
7. Simplifying the Equation:
Simplifying the right side:
144×10−6+36×10−6=180×10−6
Now we have:
153×10−6+γglass=180×10−6
8. Solving for γglass:
Rearranging gives:
γglass=180×10−6−153×10−6=27×10−6
9. Finding αglass:
Since γglass=3αglass:
3αglass=27×10−6
Therefore:
αglass=27×10−63=9×10−6°C−1
Final Answer:
The coefficient of linear expansion for glass is:
αglass=9×10−6°C−1
The apparent coefficient of expansion (γ) of a liquid in a vessel is given by the formula:
γ=γliquid−γvessel
where:
- γliquid is the volumetric expansion coefficient of the liquid.
- γvessel is the volumetric expansion coefficient of the vessel.
- Apparent expansion of mercury in glass: γapparent, glass=153×10−6°C−1
- Apparent expansion of mercury in steel: γapparent, steel=144×10−6°C−1
- Coefficient of volumetric expansion for steel: αsteel=12×10−6°C−1
The volumetric expansion coefficient (γ) is related to the linear expansion coefficient (α) by:
γ=3α
Thus, for steel:
γsteel=3αsteel=3×(12×10−6)=36×10−6°C−1
For the glass vessel:
γmercury=γapparent, glass+γglass
For the steel vessel:
γmercury=γapparent, steel+γsteel
Since both expressions equal γmercury, we can set them equal to each other:
γapparent, glass+γglass=γapparent, steel+γsteel
Substitute the known values into the equation:
153×10−6+γglass=144×10−6+36×10−6
Simplifying the right side:
144×10−6+36×10−6=180×10−6
Now we have:
153×10−6+γglass=180×10−6
Rearranging gives:
γglass=180×10−6−153×10−6=27×10−6
Since γglass=3αglass:
3αglass=27×10−6
Therefore:
αglass=27×10−63=9×10−6°C−1
The coefficient of linear expansion for glass is:
αglass=9×10−6°C−1
Topper's Solved these Questions
- CALORIMETRYBook:CENGAGE PHYSICSChapter:CALORIMETRYExercise:Multiple Correct
Explore 25 Videos
- CALORIMETRYBook:CENGAGE PHYSICSChapter:CALORIMETRYExercise:Comprehension
Explore 30 Videos
- CALORIMETRYBook:CENGAGE PHYSICSChapter:CALORIMETRYExercise:Subjective
Explore 25 Videos
- BASIC MATHEMATICSBook:CENGAGE PHYSICSChapter:BASIC MATHEMATICSExercise:Exercise 2.6
Explore 20 Videos
- CENTRE OF MASSBook:CENGAGE PHYSICSChapter:CENTRE OF MASSExercise:INTEGER_TYPE
Explore 1 Video
Similar Questions
A non-isotropic solid metal cube has coefficients of linear expansion as 5×105/∘C along the x - axis and 5×10−6/∘C along the y - axis and 15×106/∘C along Z axis . If the coefficient of volume expansion of the solid is C×10−6/∘C then the value of C is ____.
View SolutionA steel wire AB of length 85cm at 10∘C is fixed rigidly at points A and B in an aluminium frame as shown. If the temperature of the system is raised to 110∘C , what extra stress will be produced in the wire relative to aluminium frame. Assume that coefficient of linear expansion for aluminium and steel are 23×10−6/∘C and 11×10−6/∘C respectively and Young's moduls for steel is 2×1011 pa.
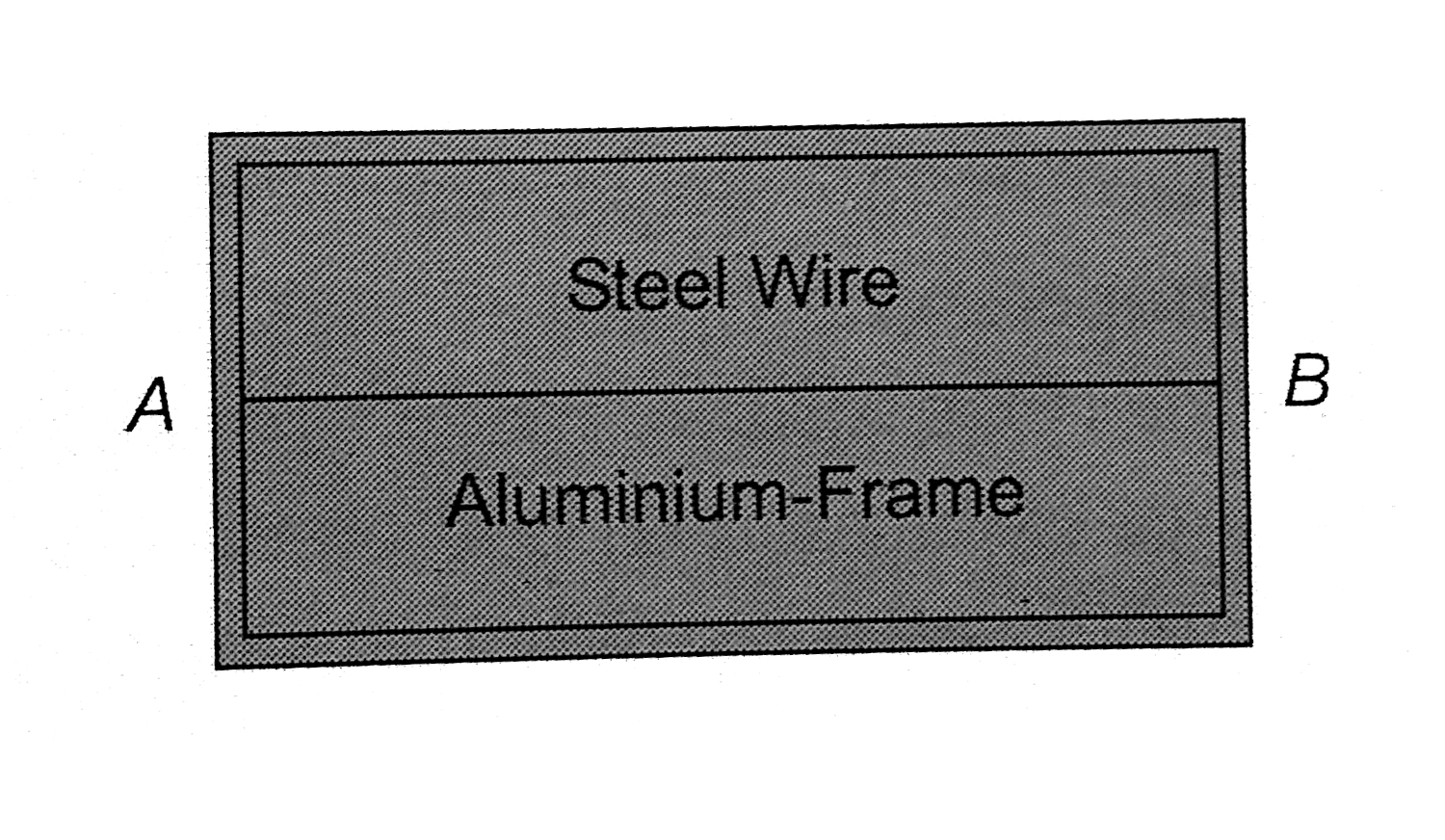
View Solution
Knowledge Check
The coefficient of apparent expansion of mercury in a glass vessel is 153×10−6/∘C and in a steel vessel is 144×10−6/∘C. If α for steel is 12×10−6/∘C then, that of glass is
A9×10−6/∘CB6×10−6/∘CC36×10−6/∘CD27×10−6/∘CThe coefficient of linear expansion of a metal rod is 12×10−6/0C, its value in per ^(0)F
A203×10−6/0FB154×10−6/0FC21.6×10−6/0FD12×10−6/0FA flask of volume 103 cc is completely filled with mercury at 0∘C The coefficient of cubical expansion of mercury is 180×10−6/∘C and heat of glass is 40×10−6/∘C. If the flask in now placed in boiling water at 100∘C how much mercury will overflow?
A7 ccB14 ccC21 ccD28cc
A steel rod of length 5m is fixed between two support. The coefficient of linear expansion of steel is 12.5×10−6/∘C. Calculate the stress (in 108N/m2) in the rod for an increase in temperature of 40∘C. Young's modulus for steel is 2×1011Nm−2
View SolutionWhen a liquid is heated in a glass vessel, its coefficient of apparent expension is 1.03×10−3/∘C. When the same liquid is heated in a copper vessel, its coefficient of apparent expansion is 1.006×10−3/∘C. If the coefficient of linear expension of copper is 17×10−6/∘C, then the coefficient of linear expansion of glass
View SolutionCoefficient of apparent expansions of mercury is 0.18×10−3/0C. If the density of mercury at 00C is 13.6g/cc its density at 473K will be
View SolutionA glass flask of volume 1 litre is fully filled with mercury at 0∘C. Both the flask and mercury are now heated to 100∘C. If the coefficient of volume expansion of mercury is 1.82×10−4/∘C, volume coefficient of linear expansion of glass is 10×10−6/∘C, the amount of mercury which overflows is
View SolutionThe coefficient of real expansion of liquid is 7×10−4/0C. The co efficient of linear ex/pansion of the vessel is 1×10−5/0C. The coefficient of apparent expansion of the liquid is
View Solution