A copper and a tungsten plate having a thickness σ=2mm each are riveted together so that at 0∘C they form a flat bimetallic plate. Find the average radius of curvature of this plate at T=200∘C. The coefficients of linear expansion for copper and tungsten are
αcu=1.7×10−5/K and αW=0.4×10−5/K, respectively.
0.67m
0.45m
0.79m
0.77m
The correct Answer is:D
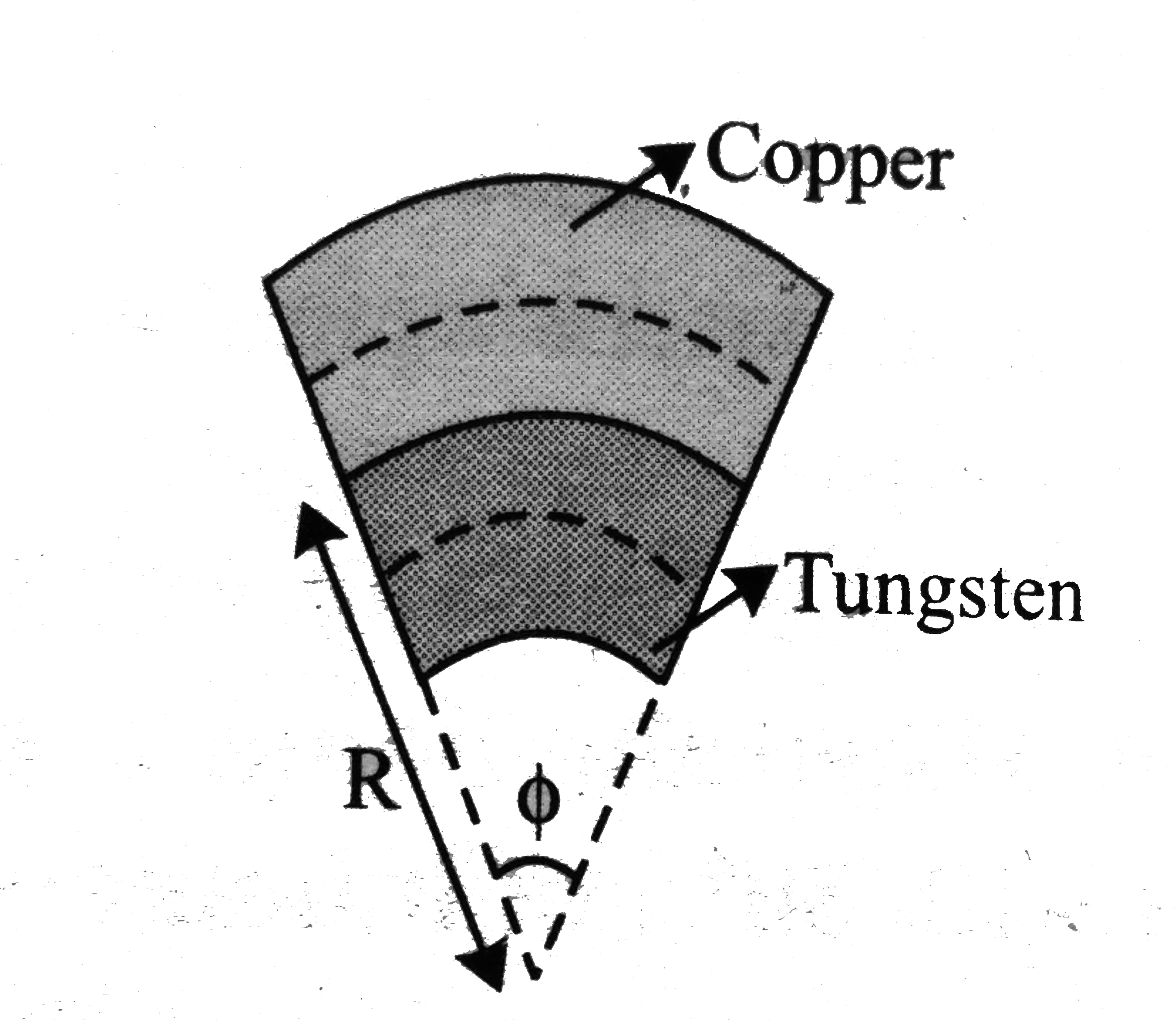
The average length of copper plate at a temperature T=200∘C is lC=l0(1+αcT),
where l0 is the length of copper plate at 0∘C. The length of the tungsten plate is lt=l0(1+αtT)
We shall assume that the edges of plates are not displaced during deformation and that an increase in the plate thickness duw to heating can be neglected.
From Fig we have
lC=ϕ(R+δ/2)⇒lt=ϕ(R−δ/2)
Consequently,
ϕ(R+δ/2)=l0(1+αCT) ..(i)
ϕ(R−δ/2)=l0(1+αtT) ..(ii)
To eliminate the unknown quantities ϕ and l0, we divide Eq. (i) by Eq. (ii) term wise.
⇒(R+δ/2)(R−δ/2)=(1+αcT)(1+αtT)
⇒R=δ[2+(αc+αt)T](αc−αt)T
⇒R=δ(αc−αt)T
neglecting (αC+αt) in numerator. Sustituting the values in above relation we get R=0.769m.
Topper's Solved these Questions
- CALORIMETRYBook:CENGAGE PHYSICSChapter:CALORIMETRYExercise:Exercise 1.1
Explore 23 Videos
- CALORIMETRYBook:CENGAGE PHYSICSChapter:CALORIMETRYExercise:Exercise 1.2
Explore 22 Videos
- BASIC MATHEMATICSBook:CENGAGE PHYSICSChapter:BASIC MATHEMATICSExercise:Exercise 2.6
Explore 20 Videos
- CENTRE OF MASSBook:CENGAGE PHYSICSChapter:CENTRE OF MASSExercise:INTEGER_TYPE
Explore 1 Video
Similar Questions
An iron rod and a copper rod lie side by side. As the temperature is changed, the difference in the lengths of the rods remains constant at a value of 10cm . Find the lengths at 0∘C . Coefficient of linear expansion of iron and copper are 1.1×10−5/∘C and 1.7×10−5/∘C respectively.
View SolutionA copper bar is 80 cm long at 15∘C. What is the increase in length, when heated to 35∘C? The coefficient of linear expansion for copper is 1.7×10−5.∘C−1
View Solution
Knowledge Check
A copper and a tungsten plate having a thickness σ=2mm each are riveted together so that at 0∘C they form a flat bimetallic plate. Find the average radius of curvature of this plate at T=200∘C. The coefficients of linear expansion for copper and tungsten are
αcu=1.7×10−5/K and αW=0.4×10−5/K, respectively.A0.67mB0.45mC0.79mD0.77mA copper and a tungsten plate having a thickness d each are riveted together so that at 0∘C they form a flat bimetallic plate. Find the average radius of the curvature of this plate at temperature T. the coefficient of linear expansion for copper and tungsten are αCuandαW.
Aδ[1+(αc+αt)T][2(αc−αt)T]Bδ[1+(αc+αt)T][(αc−αt)T]Cδ[2+(αc+αt)T][(αc−αt)T]Dδ[2+(αc+αt)T][2(αc−αt)T]Two identical thin metal strips one of aluminium and the other of iron are riveted together to form a bimetallic strip. The temperature is raised by 50∘C. If the central planes of the two strips are separated by 2nm and the coefficients of thermal expansion of aluminium and iron are respectively 30×10−6/∘C and 10×10−6/∘C the average radius of curvature of the bimetallic strip is about
A50 cmB100 cmC150 cmD200 cm
A bimetallic strip of thickness 2cm consists of zinc and silver rivetted together. The approximate radium of curvature of the strip when heated through 500C will be : (linear expansivity of zinc and silver are 32×10−6/∘C and 19×10−6/∘C respectively)
View SolutionAn iron of length 50cm is joined at an end to copper rod to length 100cm at 200C. Find the length of the system at 1000C and average coefficient of linear expansion of the systeam.
(⋅iron=12×10−6/0Cand⋅copper=17×10−6/0C.)
View SolutionIn a cylinder whose diameter is exactly 1.0 cm at 30∘C is to be slid into a hole of diameter 0.9997 cm in a steel plate at the same temperature. The minimum required rise in temperature of the plate is (The coefficient of linear expansion of steel = 12×10−6/∘C ) :
View SolutionA metal cube of length of 10.0 mm at 0∘C is heated to 200∘C. Given: its coefficient of linear expansion is 2×10−5K−1. The percent change of its volume is
View SolutionDetermine the lengths of an iron rod and copper ruler at 0∘ C if the difference in their lengths at 50∘C and 450∘C is the same and is equal to 2 cm. The coefficient of linear expansion of iron=12×10−6/K and that of copper=17×10−6/K.
View Solution