A wire of cross-sectional area A forms three sides of a square and is free to rotate about axis OO'. If the structure is deflected by an angle θ from the vertical when current i is passed through it in a magnetic field B acting vertically upward and density of the wire is ρ, then the value of θ is given by

2AρgiB=cotθ
2AρgiB=tanθ
AρgiB=sinθ
Aρg2iB=cosθ
The correct Answer is:a
Let a is the side of squre.

Torque of current =MBsin(90−θ)
=MBcosθ=ia2Bcosθ
Mass of each side =m=ρAa
Torque of gravity =2mga2sinθ+mgasinθ
=2mgasinθ=2ρAga2sinθ
Now, both torques should be same
i.e., ia2Bcosθ=2ρAga2sinθ
⇒cotθ=2ρAgiB
Topper's Solved these Questions
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercises Multiple Correct
25 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercises Assertion -reasoning
8 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercises Subjective
10 Videos
- KINETIC THEORYBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORYEXERCISE - Question Bank
31 Videos
- Magnetism and MatterBOOK - CENGAGE PHYSICSCHAPTER - Magnetism and MatterEXERCISE - Question Bank
50 Videos
Similar Questions
A wire of c.s.S = 2.5mm2 is bent to make the three sides of a square and is free to turn about the horizontal axis ∈fity Find the magnetic induction B which is vertically upward if the frame is deflected by θ=30∘) from the vertical when a current I=16A is passed through the wire. The density of the wire σ=8900kgm−3
View SolutionA copper wire with cross-sectional area S=2.5mm2 bent to make three sides of a square can turn about a horizontal axis OO' (Fig). The wire is located in unifrom vertical magnetic field. Find the magnetic induction if on passing a current I=16A through the wire the latter deflects by an angle θ=20∘.
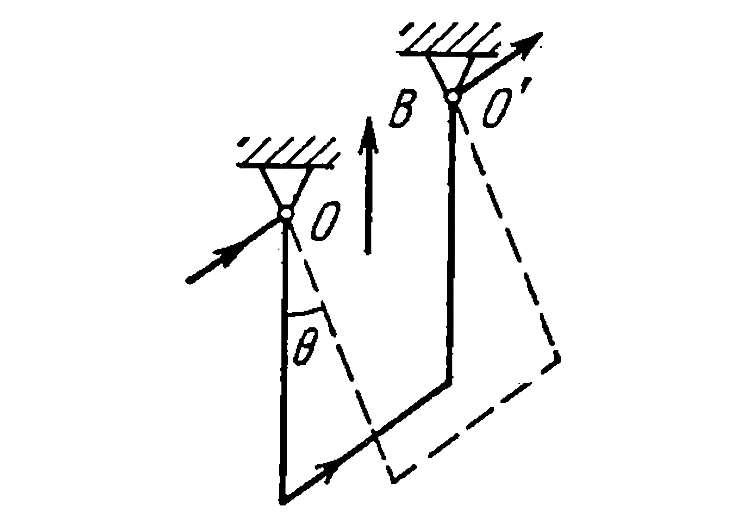
View Solution
Knowledge Check
A wire of length 'L' is bent in to an arc of a circle and found to subtentd and angle of 'θ' radians at the centre.If a current of 'I' is passed through it, the magnetic induction at the center of the circle is
Aμ0iθ22πLBμ0iθ24πLCμ0iθ4πLDμ0iθ2πLAs is the cross sectional area and ρ is the specific resistance of a potentiometer wire. If I is the current passing through the potentiometer wire, then the potential gradient along the length of the wire is given by
AIAρBIAρCIρADIρAA wire bent in the form of a sector of radius r subtending an angle θ∘ at centre as shown in figure is carrying a current I. The magnetic field at O is :
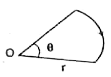 Aμ0I2rθBμ0I2r(θ/180)Cμ0I2r(θ/360)Dzero
Aμ0I2rθBμ0I2r(θ/180)Cμ0I2r(θ/360)Dzero
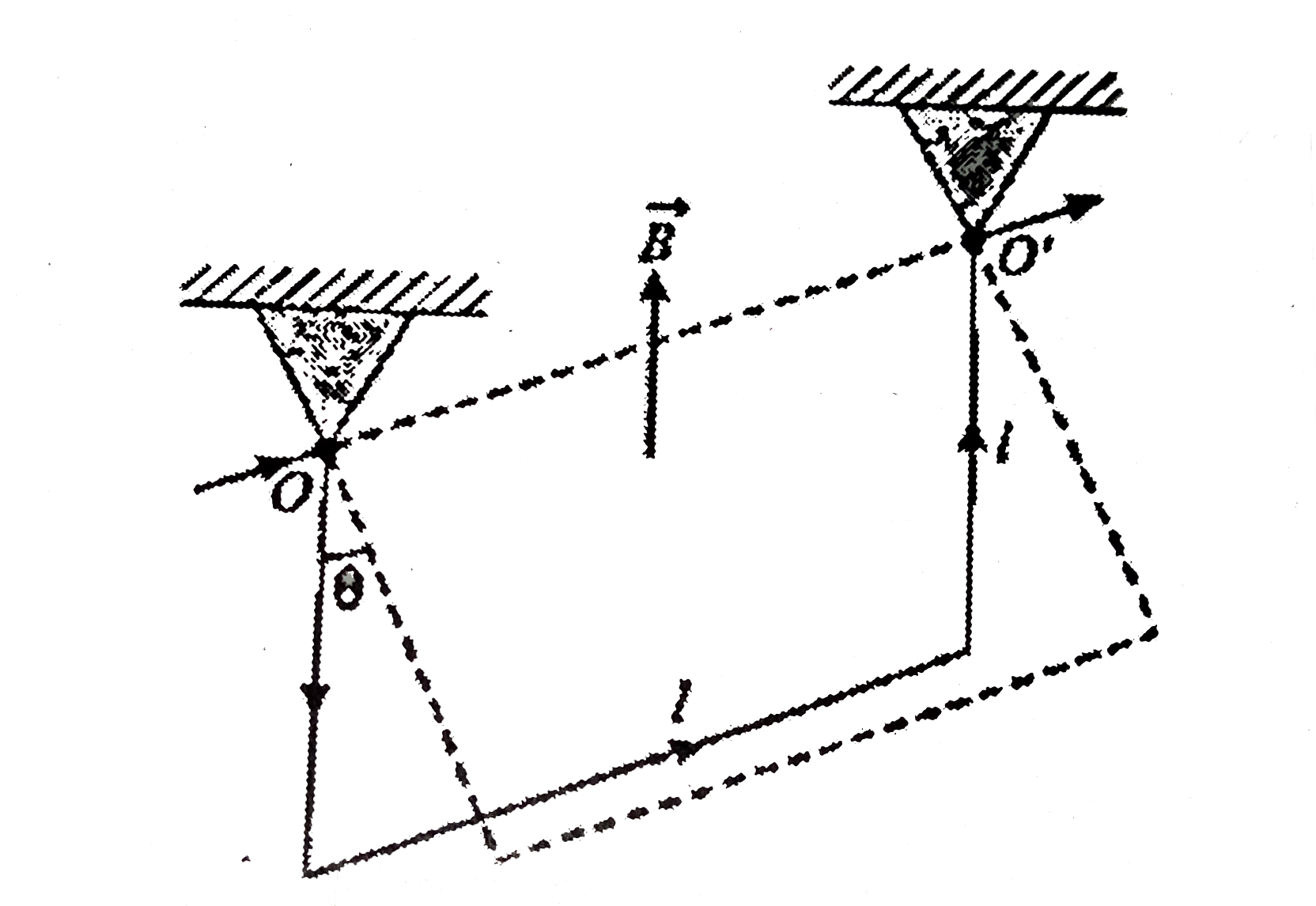
A copper wire with density ρ with cross-sectional area S bent to make three sides of a squae frame which can turn about a horizontal axis OO' as shown in figure. The wire is located in uniform vertical magnetic field. Find the magnetic induction if on passing a current I through the wire the frame deflects by an angle θ in its equilibrium position.
View SolutionA wire of length l is bent in the form of an equilateral triangle and carries an electric current i. (a) Find the magnetic field B at the centre. (b) If the wire is bent in the form of a square, what would be the value of B at the centre?
View SolutionWhen current is passed through a circular wire prepared from a conducting material, the magnetic field produced at its centre is B. Now a loop having two turns is prepared from the same wire and the same current is passed through it. The magnetic field at its centre will be :
View SolutionA circular coil of radius 20cm and 20 turns of wire is mounted vertically with its plane in magnetic meridian.A small magnetic needle (free to rotate about vertical axis) is placed at the center of the coil.It is deflected through 45∘ when a current is passed through the coil and in equilbrium (Horizontal component of earth's field is 0.34×10−4T).The current in coil is:
View SolutionA straight peice of conducting wire with mass M and length L is placed on a frictionless incline tilited at an angle θ from the horizontal (as shown in fig) There is a uniform. Vertical magnetic field at all points (produced by an arrangement of magnets not shown in fig. To keep the wire from sliding down the incline, a voltage source is

attached to the ends of the wire. When just the right amount of current flows through the wire, the wire remains at rest. Determine the magnitude and direction of the current in the wire that will cause the wire to remain at rest.
View Solution
